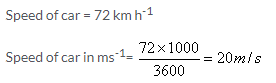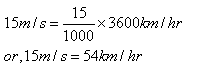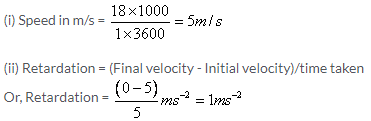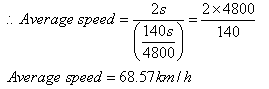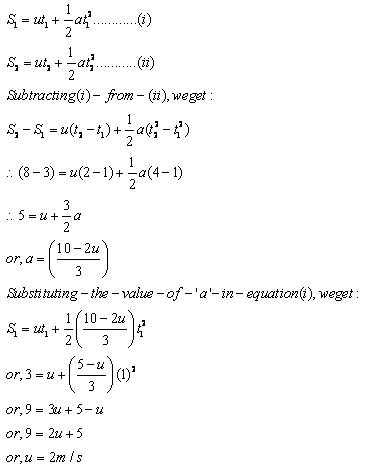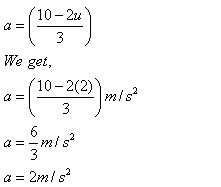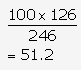Selina ICSE Solutions Archives

Selina Concise Biology Class 9 ICSE Solutions Digestive System

November 28, 2020August 24, 2018 by Veerendra

Selina Concise Biology Class 9 ICSE Solutions Digestive System

APlusTopper.com provides step by step solutions for Selina Concise ICSE Solutions for Class 9 Biology Chapter 9 Chapter 11 Digestive System. You can download the Selina Concise Biology ICSE Solutions for Class 9 with Free PDF download option. Selina Publishers Concise Biology for Class 9 ICSE Solutions all questions are solved and explained by expert teachers as per ICSE board guidelines.

Download Formulae Handbook For ICSE Class 9 and 10

ICSE Solutions Selina ICSE Solutions

Selina ICSE Solutions for Class 9 Biology Chapter 11 Digestive System

Exercise 1

Solution A.

(iii) stomach into intestine
(i) HCl and pepsin
(iii) colon
(iii) Premolars, molars – Grinding

Solution B.1.

Solution B.2.

The two reflexes which occur when a person chews and swallows food are:

Reflex when a person chews – Secretion of saliva
Reflex when a person swallows – Tongue presses upward and back against the roof (palate)

Solution B.3.

(ii) Both the statements are wrong. Small intestine is longer (7 metres) than large intestine (1.5 metres). Also, large intestine is so called because of its width which is larger than that of small intestine.

Solution C.1.

Digestion is the process of breaking down complex food materials into simpler substances by the action of enzymes.

Need for a digestive system:

Large complex molecules like carbohydrates, proteins and lipids need to be broken down to simpler molecules. These simpler molecules can then be absorbed and utilized by the body.
The breaking down of complex food molecules into their simpler form is possible only through the process of digestion.
During digestion, large complex macromolecules present in food are converted into small simpler molecules, which can be simplified in different compartments of digestive system only.

Solution C.2.

SUBSTRATE	END PRODUCTS
Starch	Maltose
Proteins	Small peptides and amino acids
Fats	Fatty acids and glycerol

Solution C.3.

Vitamins are used in their original form by the cells. They do not require digestion. They are either water soluble or fat soluble, hence no enzyme is required to digest vitamins. They are absorbed directly from the digestive tract, transported by blood to the cells, and the cells absorb and use them whenever they need. Besides, vitamins themselves act as catalysts or enzymes in essential chemical reactions that take place in the body.

Solution C.4.

It is very important to chew our food thoroughly as chewing of food helps to break down complex food materials into simpler substances. The act of chewing stimulates the salivary glands to release saliva. The saliva helps to moisten the food and form bolus, which can be swallowed easily. Saliva also contains special enzymes that help to break down carbohydrates.

Solution C.5.

Rectum acts as a temporary storage site for undigested food. It has voluntary smooth muscles that remove the faeces out of the body through the anus.

Solution C.6.

Roughage is a dietary fibre that largely consists of cellulose. It cannot be digested by our body as our body does not contain cellulose-digesting enzymes.

Examples of roughage:

Fruits
Green leafy vegetables

Solution C.7.

Adaptations of ileum for the absorption of digested food:

Very long to provide more surface area for absorption
Presence of large number of villi to further increase the surface area

Solution C.8.

Functions of hydrochloric acid:

It gets mixed with food and kills the bacteria present in food.
It activates pepsin to act on proteins.

Solution D.1.

Vegetarian menu for dinner:

Foodstuffs	Weight (gm/ml)
Cereals	320
Pulses	70
Green leafy vegetables	100
Root vegetables	75
Fruits	75
Milk	600
Fat and oil	30
Brown sugar and jaggery	30

Solution D.2.

Main characteristics of an enzyme:

It is a protein and therefore, gets destroyed by heating.
It acts only on one kind of substance called the substrate. So, it is very specific.
It acts as a catalyst, so it can be used again and again.
It only affects the rate of a chemical reaction and always speeds up the reaction.
It always forms the same end products from the fixed substrate.
It acts best only at a particular pH.
It acts best within a narrow temperature range, usually between 35°C-40°C.

Solution D.3.

The small intestine is the most important organ of the digestive system as it serves both, for digestion and absorption. It receives two digestive juices; the bile and the pancreatic juice in the duodenum. These two juices virtually complete the digestion of starch, proteins, carbohydrates, etc. After the breakdown of food, the small intestine absorbs simple substances such as glucose, amino acids, etc.

Solution D.4.

Liver is an important organ in our body as it serves the following functions:

Production of bile
Control of blood sugar levels
Control of amino acid levels
Synthesis of foetal red blood cells
Produce fibrinogen and heparin
Regulate blood volume
Destroy dead red blood cells
Store vitamin and minerals
Excrete toxic and metallic poisons
Produce heat
Detoxification

Solution D.5.

(i) Peristalsis: Peristalsis is the rhythmic contraction and relaxation of the muscles of the alimentary canal that pushes the food along the gut.
(ii) Omnivore: Omnivores are organisms that consume both plants and animals.
(iii) Pylorus: Pylorus is the passage at the lower end of the stomach that opens into the duodenum.
(iv) Kilocalorie: A kilocalorie is a unit of energy. It is the energy required to raise the temperature of 1 kg of water by 1 Celsius.
(v) Basal metabolic rate: Basal metabolic rate refers to the minimum amount of energy in the form of calories that our body requires to complete its normal functions.

Solution D.6.

REGION	ENZYME	ACTION ON FOOD
Stomach	Pepsin	Acts on proteins and converts it into polypeptides
Small intestine
Duodenum	Amylopectin	Acts on starch and converts it into maltose
Duodenum	Trypsin	Acts on remaining proteins, proteoses and peptones to produce peptides and amino acids
Ileum	Erepsin	Acts on proteins and polypeptides and converts them into small peptides and amino acids
	Sucrase	Acts on sucrose and converts it into glucose and fructose
	Lactase	Acts on lactose and converts it into glucose and galactose

Solution D.7.

Importance of water in our body:

Water is the major component of blood, which carries nutrients and oxygen, to and from all the cells.
It is the major component of saliva and mucous, which lubricate the membranes that line our digestive system beginning with the mouth.
It helps in regulating the temperature of the body.
Water is very essential for digestion as well as absorption of food.

Solution D.8.

Test for starch:

Takethe food item to be tested. Put it into a test-tube containing water and boil to make a solution.
Cool the solution and add 2-3 drops of dilute iodine solution to it.
Blue-black colour of the solution indicates the presence of starch in the food item.

Test for proteins:

Take the food item to be tested in a test tube.
Add few drops of dilute nitric acid to it.
Heat the test-tube gently.
Rinse off the acid with water and add few drops of ammonium hydroxide to it.
Colour changefrom colourless to yellow and then from yellow to orange redindicates the presence of protein in the food item.

Solution E.1.

Selina Concise Biology Class 9 ICSE Solutions Digestive System 1

Solution E.2.

While swallowing saliva in the mouth, the larynx is pulled upwards to bring it close to the back of the tongue, when a flap called epiglottis closes its opening. Then, it goes towards the oesophagus.

Solution E.3.

Organ	Enzyme	Food acted upon	Find product
Stomach	Pepsin	Proteins	Polypeptide
Mouth	Amylase	Starch	Disaccharide
Ileum	Maltase	Maltose	Glucose

Solution E.4.

(a)
1 → Enamel
2 → Dentine
3 → Pulp
4 → Gum
5 → Crown
6 → Cement

(b) The tooth shown in the diagram has only one root, so it is an incisor or canine which is used for biting and piercing.

(c) The part labelled ‘3’ (pulp) is a soft connective tissue present in the pulp cavity of the tooth. It consists of blood capillaries, lymph vessels and nerve fibres. These extend from the crown of the tooth and open through the pulp cavity at the base of the root.

(d) Type of teeth in the mouth of an adult:

Incisors (8) → Used for biting and cutting
Canines (4) → Used for holding and tearing of food
Premolars (8) → Used for grinding and crushing of food
Molars (12)→ Used for grinding and crushing of food

Solution E.5.

a) A total of 20 teeth are present in the given dentition.

(b) The given dentition is that of a herbivore because there are no canines present in the dentition. Canines are required by carnivores as they help in holding and tearing of food. The teeth of herbivores are used for cutting, gnawing, and biting, while the teeth of carnivores are sharper and more suited for catching, killing and tearing the prey.

(d)
selina-icse-solutions-class-9-biology-digestive-system-b1

More Resources for Selina Concise Class 9 ICSE Solutions

Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension

December 3, 2020August 24, 2018 by Veerendra

Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension

ICSE Solutions Selina ICSE Solutions

APlusTopper.com provides step by step solutions for Selina Concise ICSE Solutions for Class 9 Physics Chapter 2 Motion in One Dimension. You can download the Selina Concise Physics ICSE Solutions for Class 9 with Free PDF download option. Selina Publishers Concise Physics for Class 9 ICSE Solutions all questions are solved and explained by expert teachers as per ICSE board guidelines.

Download Formulae Handbook For ICSE Class 9 and 10

Selina ICSE Solutions for Class 9 Physics Chapter 2 Motion in One Dimension

Exercise 2(A)

Solution 1S.

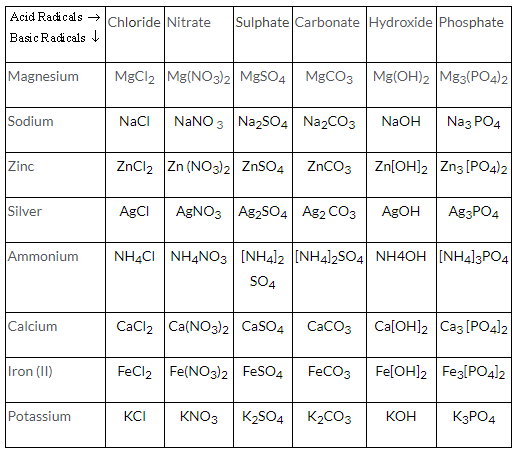
Scalar	Vector
They are expressed only by their magnitudes.	They are expressed by magnitude as well as direction.
They can be added, subtracted, multiplied or divided by simple arithmetic methods.	They can be added, subtracted or multiplied following a different algebra.
They are symbolically written by English letter.	They are symbolically written by their English letter with an arrow on top of the letter.
Example: mass, speed	Example: force, velocity

Solution 2S.

a) Pressure is a scalar quantity.
b) Momentum is a vector quantity.
c) Weight is a vector quantity.
d) Force is a vector quantity.
e) Energy is a scalar quantity.
f) Speed is a scalar quantity.

Solution 3S.

A body is said to be at rest if it does not change its position with respect to its immediate surroundings.

Solution 4S.

A body is said to be in motion if it changes its position with respect to its immediate surroundings.

Solution 5S.

When a body moves along a straight line path, its motion is said to be one-dimensional motion.

Solution 6S.

The shortest distance from the initial to the final position of the body is called the magnitude of displacement. It is in the direction from the initial position to the final position.
Its SI unit is metre (m).

Solution 7S.

Distance is a scalar quantity, while displacement is a vector quantity. The magnitude of displacement is either equal to or less than the distance. The distance is the length of path travelled by the body so it is always positive, but the displacement is the shortest length in direction from initial to the final position so it can be positive or negative depending on its direction. The displacement can be zero even if the distance is not zero.

Solution 8S.

Yes, displacement can be zero even if the distance is not zero.

For example, when a body is thrown vertically upwards from a point A on the ground, after sometime it comes back to the same point A. Then, the displacement is zero, but the distance travelled by the body is not zero (it is 2h; h is the maximum height attained by the body).

Solution 9S.

The magnitude of displacement is equal to distance if the motion of the body is one-dimensional.

Solution 10S.

The velocity of a body is the distance travelled per second by the body in a specified direction.
Its SI unit is metre/second (m/s).

Solution 11S.

The speed of a body is the rate of change of distance with time.
Its SI unit is metre/second (m/s).

Solution 12S.

Speed is a scalar quantity, while velocity is a vector quantity. The speed is always positive-it is the magnitude of velocity, but the velocity is given a positive or negative sign depending upon its direction of motion. The average velocity can be zero but the average speed is never zero.

Solution 13S.

Velocity gives the direction of motion of the body.

Solution 14S.

Instantaneous velocity is equal to average velocity if the body is in uniform motion.

Solution 15S.

If a body travels equal distances in equal intervals of time along a particular direction, then the body is said to be moving with a uniform velocity. However, if a body travels unequal distances in a particular direction in equal intervals of time or it moves equal distances in equal intervals of time but its direction of motion does not remain same, then the velocity of the body is said to be variable (or non-uniform).

Solution 16S.

Average speed is the ratio of the total distance travelled by the body to the total time of journey, it is never zero. If the velocity of a body moving in a particular direction changes with time, then the ratio of displacement to the time taken in entire journey is called its average velocity. Average velocity of a body can be zero even if its average speed is not zero.

Solution 17S.

The motion of a body in a circular path with uniform speed has a variable velocity because in the circular path, the direction of motion of the body continuously changes with time.
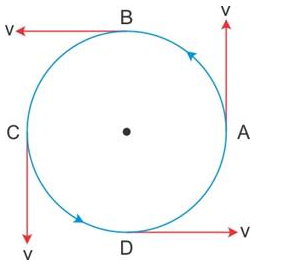
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 1

Solution 18S.

If a body starts its motion from a point and comes back to the same point after a certain time, then the displacement is zero, average velocity is also zero, but the total distance travelled is not zero, and therefore, the average speed in not zero.

Solution 19S.

Acceleration is the rate of change of velocity with time.
Its SI unit is metre/second² (m/s²).

Solution 20S.

Acceleration is the increase in velocity per second, while retardation is the decrease in velocity per second. Thus, retardation is negative acceleration. In general, acceleration is taken positive, while retardation is taken negative.

Solution 21S.

The acceleration is said to be uniform when equal changes in velocity take place in equal intervals of time, but if the change in velocity is not the same in the same intervals of time, the acceleration is said to be variable.

Solution 22S.

Retardation is the decrease in velocity per second.
Its SI unit is metre/second² (m/s²).

Solution 23S.

Velocity determines the direction of motion.

Solution 24S.

(a) Example of uniform velocity: A body, once started, moves on a frictionless surface with uniform velocity.
(b) Example of variable velocity: A ball dropped from some height is an example of variable velocity.
(c) Example of variable acceleration: The motion of a vehicle on a crowded road is with variable acceleration.
(d) Example of uniform retardation: If a car moving with a velocity ‘v’ is brought to rest by applying brakes, then such a motion is an example of uniform retardation.

Solution 25S.

Initially as the drops are equidistant, we can say that the car is moving with a constant speed but later as the distance between the drops starts decreasing, we can say that the car slows down.

Solution 26S.

When a body falls freely under gravity, the acceleration produced in the body due to the Earth’s gravitational acceleration is called the acceleration due to gravity (g). The average value of g is 9.8 m/s².

Solution 27S.

No. The value of ‘g’ varies from place to place. It is maximum at poles and minimum at the Equator on the surface of the Earth.

Solution 28S.

In vacuum, both will reach the ground simultaneously because acceleration due to gravity is same (=g) on both objects.

Solution 1M.

Velocity is a vector quantity. The others are all scalar quantities.

Solution 2M.

m s^-1

Solution 3M.

m s^-2

Solution 4M.

The displacement is zero.

Solution 5M.

5 m s^-1

Solution 1N.

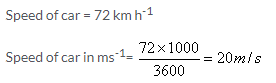
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 2

Solution 2N.

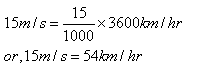
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 3

Solution 3N.

Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 4

Solution 4N.

18 km h^-1 < 10 m s^-1 < 1 km min^-1

Solution 5N.

Total time taken = 3 hours
Speed of the train = 65 km/hr
Distance travelled = speed x time
= 65 x 3 = 195 km

Solution 6N.

Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 5

Solution 7N.

Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 6
(ii) Average velocity of the train is zero because the train stops at the same point from where it starts, i.e. the displacement is zero.

Solution 8N.

Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 7

Solution 9N.

Here, final velocity = 10 m/s
Initial velocity = 0 m/s
Time taken = 2s
Acceleration = (Final Velocity – Initial Velocity)/time
= (10/2) m/s² = 5 m/s²

Solution 10N.

Here, final velocity = 180 m/s
Initial velocity = 0 m/s
Time taken = 0.05 h or 180 s
Acceleration = (Final Velocity – Initial Velocity)/time
= (180-0)/180 m/s² = 1 m/s²

Solution 11N.

Here, final velocity = 20 m/s
Initial velocity = 50 m/s
Time taken = 3 s
Acceleration = (Final Velocity – Initial Velocity)/time
= (20 – 50)/3 m/s² = -10 m/s²Negative sign here indicates that the velocity decreases with time, so retardation is 10 m/s².

Solution 12N.

Here, final velocity = 18 km/h or 5 m/s
Initial velocity = 0 km/h
Time taken = 2 s
Acceleration = (Final Velocity – Initial Velocity)/time
= (5 – 0) / 2 m/s² = 2.5 m/s²

Solution 13N.

Acceleration = Increase in velocity/time taken
Therefore, increase in velocity = Acceleration × time taken
= (5 × 2) m/s
= 10 m/s

Solution 14N.

Initial velocity of the car, u = 20 m/s
Retardation = 2 m/s²Given time, t = 5 s
Let ‘v’ be the final velocity.
We know that, Acceleration = Rate of change of velocity /time
= (Final velocity – Initial velocity)/time
Or, -2 = (v – 20) / 5
Or, -10 = v – 20
Or, v = -20 + 10 m/s
Or, v = -10 m/s
Negative sign indicates that the velocity is decreasing.

Solution 15N.

Initial velocity of the bicycle, u = 5 m/s
Acceleration = 2 m/s²Given time, t = 5 s
Let ‘v’ be the final velocity.
We know that, acceleration = Rate of change of velocity/time
= (Final velocity – Initial velocity)/time
Or 2 = (v – 5)/5
Or, 10 = (v – 5)
Or, v = -5 – 10
Or, v = -15 m/s
Negative sign indicates that the velocity is decreasing.

Solution 16N.

Initial velocity of the bicycle, u = 18 km/hr
Time taken, t = 5 s
Final velocity, v = 0 m/s (As the car comes to rest)
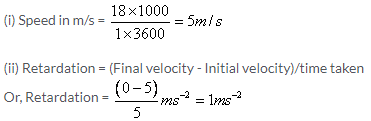
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 8
(iii) Let ‘V’ be the speed of the car after 2 s of applying the brakes.
Then, Acceleration = (V – 5)/ 2
Or, -1 = (V – 5)/2
Or, V = -2 + 5
Or, V = 3 m/s²

Exercise 2(B)

Solution 1S.

For the motion with uniform velocity, distance is directly proportional to time.

Solution 2S.

From displacement-time graph, the nature of motion (or state of rest) can be understood. The slope of this graph gives the value of velocity of the body at any instant of time, using which the velocity-time graph can also be drawn.

Solution 3S.

(a) Slope of a displacement-time graph represents velocity.
(b) The displacement-time graph can never be parallel to the displacement axis because such a line would mean that the distance covered by the body in a certain direction increases without any increase in time, which is not possible.

Solution 4S.

(a) There is no motion, the body is at rest.
(b) It depicts that the body is moving away from the starting point with uniform velocity.
(c) It depicts that the body is moving towards the starting point with uniform velocity.
(d) It depicts that the body is moving with variable velocity.

Solution 5S.

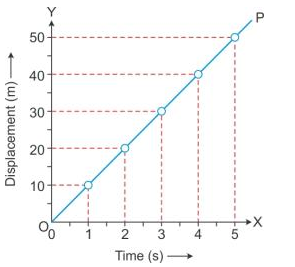
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 9

Solution 6S.

(i) The slope of the velocity-time graph gives the value of acceleration.
(ii) The total distance travelled by a body in a given time is given by the area enclosed between the velocity-time graph and X-axis (without any sign).
(iii) The displacement of a body in a given time is given by the area enclosed between the velocity-time graph and X-axis (with proper signs).

Solution 7S.

Vehicle A is moving with a faster speed because the slope of line A is more than the slope of line B.

Solution 8S.

(a) Fig. 4.33 (a) represents uniformly accelerated motion. For example, the motion of a freely falling object.
(b) Fig. 4.33 (b) represents motion with variable retardation. For example, a car approaching its destination.

Solution 9S.

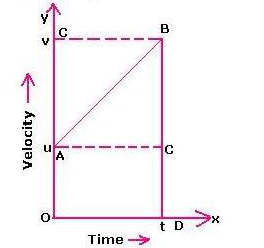
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 10
In this graph, initial velocity = u
Velocity at time t = v
Let acceleration be ‘a’
Time = t
Then, distance travelled by the body in t s = area between the v-t graph and X-axis
Or distance travelled by the body in t s = area of the trapezium OABD
= (1/2) × (sum of parallel sides) × (perpendicular distance between them)
= (1/2) × (u + v) × (t)
= (u + v)t /2

Solution 10S.

The slope of the velocity-time graph represents acceleration.

Solution 11S.

Car B has greater acceleration because the slope of line B is more than the slope of line A.

Solution 12S.

For body A: The graph is a straight line. So, the slope gives constant velocity. Hence, the acceleration for body A is zero.
For body B: The graph is a straight line. So, the slope gives constant velocity. Hence, the acceleration for body B is also zero.
For body C: The slope of the graph is decreasing with time. Hence, the acceleration is negative.
For body D: The slope of the graph is increasing with time. Hence, the acceleration is positive.

Solution 13S.

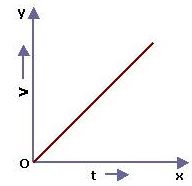
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 11
Velocity-time for a body moving with uniform velocity and uniform acceleration.

Solution 14S.

Retardation is calculated by finding the negative slope.

Solution 15S.

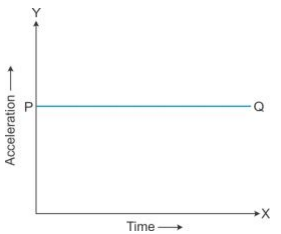
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 12
The area enclosed between the straight line and time axis for each interval of time gives the value of change in speed in that interval of time.

Solution 16S.

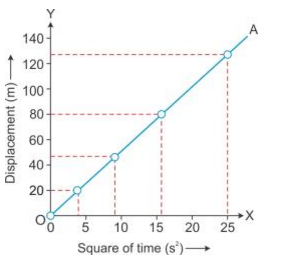
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 13

Solution 17S.

For motion under uniform acceleration, such as the motion of a freely falling body, distance is directly proportion to the square of the time.

Solution 18S.

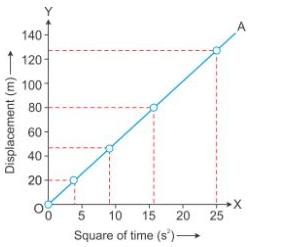
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 14

The value of acceleration due to gravity (g) can be obtained by doubling the slope of the S – t² graph for a freely falling body.

Solution 1M.

B
Acceleration is uniform.

Solution 2M.

The solution is C.
The speed-time graph is a straight line parallel to the time axis.

Solution 3M.

D
A straight line inclined to the time axis.

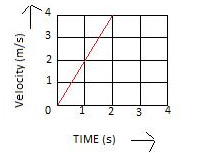
Solution 1N.

Velocity of body at t = 1s is 2 m/s
Velocity of body at t = 2s is 4 m/s
Velocity of body at t = 3s is 6 m/s
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 15

Solution 2N.

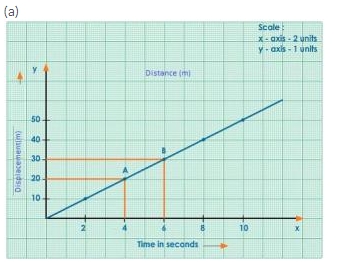
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 16
Displacement-time graph
From the part AB of the graph,
Average velocity = (Displacement at B – Displacement at A)/Time taken
= (30 – 20) m/( 6 – 4) s
= (10/2) m/s
= 5 m/s
(b) (i) From the graph, the displacement of car at 2.5 s is 12.5 m.
(ii) From the graph, the displacement of car at 4.5 s is 22.5 m.

Solution 3N.

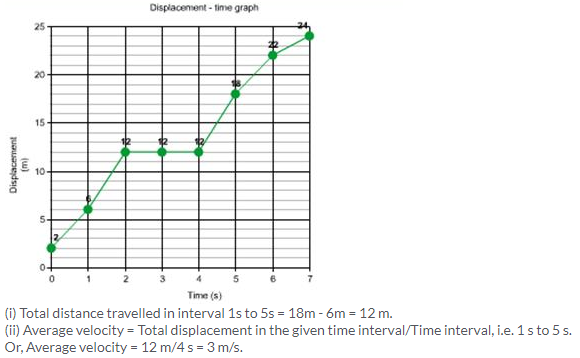
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 17

Solution 4N.

(a) (i) Velocity from 0 to 5 s = Displacement /time
= (3/5) m/s
= 0.6 m/s

(ii) Velocity from 5 s to 7 s = Displacement /time
= (0/2) m/s
= 0 m/s.

(iii) Velocity from 7 s to 9 s = Displacement /time
= (7 – 3)/(9 – 7) m/s
= (4/2) m/s
= 2 m/s

(b) From, 5 s to 9 s, displacement = 7m – 3m = 4m.
Time elapsed between 5 s to 9 s = 4 s
Average velocity = Displacement/time
= (4/4) m/s
= 1 m/s

Solution 5N.

(i) Displacement in first 4s = 10 m
Therefore, the average velocity = Displacement/time
= (10/4) m/s
= 2.5 m/s

(ii) Initial position = 0 m
Final position at the end of 10 s = -10m
Displacement = Final position – Initial position
= (-10) m – 0
= -10 m

(iii) At 7 s and 13 s, the cyclist reaches his starting point.

Solution 6N.

(i) Initially, the car B was 40 km ahead of car A.
(ii) Straight line depicts that cars A and B are moving with uniform velocities.
For car A
Displacement at t = 1 h is 40 m
Velocity = Displacement /time
= (40/1) km/h
= 40 km/h

For car B
Displacement at t = 4 h is (120 – 40) km, i.e. 80 km
Velocity = Displacement /time
= (80/4) km/h
= 20 km/h

(iii) Car A catches car B in 2 hours.
(iv) After starting, car A will catch car B at 80 km.

Solution 7N.

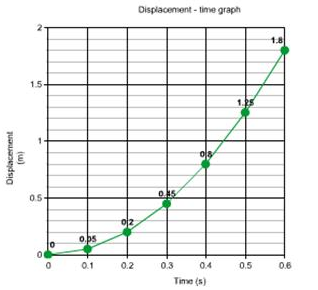
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 18

Solution 8N.

Velocity of the body at t = 1 s is 1 m/s.
Displacement of the body at t = 1 s is velocity × time = (1) × (1) m or 1 m.

Velocity of the body at t = 2s is 2 m/s.
Displacement of the body at t = 1 s is velocity × time = (2) × (2) m or 4 m.

Velocity of the body at t = 3 s is 3 m/s.
Displacement of the body at t = 3 s is velocity × time = (3) × (3) m or 9 m

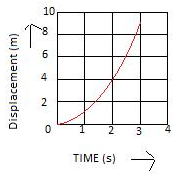
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 19

Solution 9N.

(i) Distance travelled in any part of the graph can be determined by finding the area enclosed by the graph in that part with the time axis.

(ii) Distance travelled in part BC = Area of the rectangle tBC2t = base × height.
= (2t – t) × v_o = v_ot

Distance travelled in part AB = Area of the triangle ABt
= (1/2) × base × height
= (1/2) × t × v_o = (1/2) v_o t
Therefore, distance travelled in part BC:distance travelled in part AB :: 2:1.

(iii) (a) BC shows motion with uniform velocity.
(b) AB shows motion with uniform acceleration.
(c) CD shows motion with uniform retardation.

(iv) (a) The magnitude of acceleration is lower as the slope of line AB is less than that of line CD.
(b) Slope of line AB = v_o/t
Slope of line CD = v_o/0.5t
Slope of line AB/Slope of line CD = (v_o /t)/(v_o /0.5t)
Slope of line AB:Slope of line CD :: 1:2.

Solution 10N.

(i) Acceleration in the part AB = Slope of AB
= tan (∠BAD)
= (30/4) ms^-2 = 7.5 ms^-2

Acceleration in the part BC = 0 ms^-2Acceleration in the part CD = slope of CD = -tan (∠CDA)
= -(30/2) ms^-2 = -15 ms^-2

(ii) Displacement of part AB = Area of ΔAB4 = (1/2) (4) (30)
= 60 m
Displacement of part BC = Area of rectangle 4BC8
= (30) × (4) = 120 m
Displacement of part CD = Area of ΔC8D = (1/2) (2) (30)
= 30 m

(iii) Total displacement = Displacement of part AB + Displacement of part BC + Displacement of part CD
= 60 + 120 + 30 = 210 m

Solution 11N.

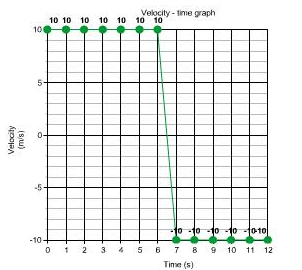
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 20
Distance travelled in first 6 s = velocity × time
= 10 m/s × 6
= 60 m/s

Distance travelled in next 6 s = velocity × time
= 10 m/s × 6
= 60 m/s

Total distance travelled in 12 s = (60 + 60) m = 120 m
Total displacement = 0, as the ball returns its starting point.

Solution 12N.

(i) From 0 to 4 seconds, the motion is uniformly accelerated and from 4 to 6 seconds, the motion is uniformly retarded.

(ii) Displacement of the particle at 6 s = (1/2) (6) (2) = 6 m

(iii) The particle does not change its direction of motion.

(iv) Distance travelled by the particle from 0 to 4s (D1) = (1/2) (4) (2) = 4 m
Distance travelled by the particle from 4 to 6s (D2) = (1/2) (2) (2) = 2 m
D1:D2:: 4:2
D1:D2:: 2:1

(v) Acceleration from 0 to 4 s = (2/4) ms^-2 or 0.5 ms^-2Retardation from 4 s to 6 s = (2/2) ms^-2 or 1 ms^-2.

Exercise 2(C)

Solution 1S.

Three equations of a uniformly accelerated motion are
v = u + at
s = ut + (1/2)at²v² = u² + 2as

Solution 2S.

Derivation of equations of motion

First equation of motion:
Consider a particle moving along a straight line with uniform acceleration ‘a’. At t = 0, let the particle be at A and u be its initial velocity, and at t = t, let v be its final velocity.
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 21
Acceleration = Change in velocity/Time
a = (v – u)/t
at = v – u
v = u+ at … First equation of motion.

Second equation of motion:
Average velocity = Total distance traveled/Total time taken Average velocity = s/t …(1)
Average velocity can be written as (u+v)/2 Average velocity = (u+v)/2 …(2)
From equations (1) and (2) s/t = (u+v)/2 …(3)
The first equation of motion is v = u + at.
Substituting the value of v in equation (3), we get
s/t = (u + u + at)/2 s = (2u + at) t/2 = 2ut + at²/2 = 2ut/2 + at²/2
s = ut + (1/2) at² …Second equation of motion.

Third equation of motion:
The first equation of motion is v = u + at. v – u = at … (1)
Average velocity = s/t …(2)
Average velocity =(u+v)/2 …(3)
From equation (2) and equation (3) we get,
(u + v)/2 = s/t …(4)
Multiplying eq (1) and eq (4) we get,
(v – u)(v + u) = at × (2s/t) (v – u)(v + u) = 2as
[We make the use of the identity a² – b² = (a + b) (a – b)]
v² – u² = 2as …Third equation of motion.

Solution 3S.

Solution 1M.

v = u + at

Solution 2M.

5 km

Solution 1N.

Initial velocity u = 0
Acceleration a = 2 m/s²Time t = 2 s
Let ‘S’ be the distance covered.
Using the second equation of motion,
S = ut + (1/2) at²S = 0 + (1/2) (2) (2)²S = 4 m

Solution 2N.

Initial velocity u = 10 m/s
Acceleration a = 5 m/s²Time t = 5s
Let ‘S’ be the distance covered.
Using the second equation of motion,
S = ut + (1/2) at²S = (10)(5) + (1/2) (5) (5)²S = 50 + 62.5
S = 112.5 m

Solution 3N.

Acceleration = Change in velocity/time taken
In the first two seconds,
Acceleration = [(33.6 – 30)/2] km/h² = 1.8 km/h² = 0.5 m/s² …(i)

In the next two seconds,
Acceleration = [(37.2 – 33.6)/2] km/h² = 1.8 km/h² = 0.5 m/s²…(ii)
From (i) and (ii), we can say that the acceleration is uniform.

Solution 4N.

Initial velocity u = 0 m/s
Acceleration a = 2 m/s²Time t = 5 s

(i) Let ‘v’ be the final velocity.
Then, (v – u)/5 = 2
v = 10 m/s

(ii) Let ‘s’ be the distance travelled.
Using the third equation of motion,
v²– u²= 2as
We get,
(10)² – (0)² = 2(2) (s)
Thus, s = (100/4) m = 25 m

Solution 5N.

Initial velocity u = 20 m/s
Final velocity v = 0
Distance travelled s = 10 cm = 0.1 m
Let acceleration be ‘a’.
Using the third equation of motion,
v²– u²= 2as
We get,
(0)² – (20)² = 2(a) (0.1)
a = -(400/0.2) m/s²a = -2000 m/s²Thus, retardation = 2000 m/s²

Solution 6N.

Initial velocity u = 20 m/s
Final velocity v = 0
Time taken t = 5 s
Let acceleration be ‘a’.
Using the first equation of motion,
v = u + at
0 = 20 + 5a
a = -4 m/s²Thus, retardation = 4 m/s²

Solution 7N.

Let ‘s’ be the distance between stations A and B.
(i) Average speed = Total distance/total time taken
Here, total distance = s + s = 2s
Total time taken = Time taken to travel from A to B + Time taken to travel from B to A.
= [(s/ 60) + (s/ 80)] s
= [ 140 s / 4800] s

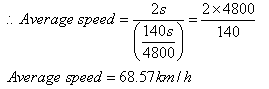
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 23

(ii) Average velocity = Displacement/total time taken
Because the train starts and ends at the same station, the displacement is zero. Thus the average velocity is zero.

Solution 8N.

Initial velocity u = 90 km/h = 25 m/s
Final velocity v = 0 m/s
Acceleration a = -0.5 m/s²

(i) Let ‘V’ be the velocity after time t = 10 s
Using the first equation of motion,
v = u + at
We get,
V = 25 + (-0.5) (10) m/s
V = 25 – 5 = 20 m/s

(ii) Let t’ be the time taken by the train to come to rest.
Using the first equation of motion,
v = u + at
We get,
t’ = [(0 – 25)/ (-0.5)] s
t’ = 50 s

Solution 9N.

Distance travelled s = 100 m
Average velocity V = 20 m/s
Final velocity v = 25 m/s

(i) Let u be the initial velocity.
Average velocity = (Initial velocity + Final velocity)/2
V = (u + v)/2
20 = (u + 25)/2
u = 40 – 25 = 15 m/s

(ii) Let ‘a’ be the acceleration of the car.
Using the third equation of motion,
v² – u² = 2as
We get,
(25)² – (15)² = 2 (a) (100)
625 – 225 = 200 a
a = (400/200) m/s² = 2 m/s²

Solution 10N.

Final velocity v = 0
Acceleration = -25 cm/s² or -0.25 m/s²Time taken t = 20 s

(i) Let ‘u’ be the initial velocity.
Using the first equation of motion,
v = u + at
We get,
u = v – at
u = 0 – (-0.25)(20) = 5 m/s

(ii) Let ‘s’ be the distance travelled.
Using the third equation of motion,
v² – u² = 2as
We get,
(0)² – (5)² = 2 (-0.25) (s)
s = (25/0.5) = 50 m.

Solution 11N.

Initial velocity u = 0 m/s
Distance travelled s = 270 m
Time taken to travel s distance = 3 s
Let ‘a’ be the uniform acceleration.
Using the second equation of motion,
S = ut + (1/2) at²We get,
270 = 0 + (1/2) a (3)²270 = 9a/2
a = 60 m/s²

Let v be the velocity of the body 10 s after the start.
Using the first equation of motion, we get
v = u + at
v = 0 + (60)(10) = 600 m/s

Solution 12N.

Let the constant acceleration with which the body moves be ‘a’.
Given, the body travels distance S₁ = 3 m in time t₁ = 1 s.
Same body travels distance S₂= 8 m in time t₂= 2 s.

(i) Let ‘u’ be the initial velocity.
Using the second equation of motion,
S = ut + (1/2) at²Substituting the value for S₁ and S₂, we get
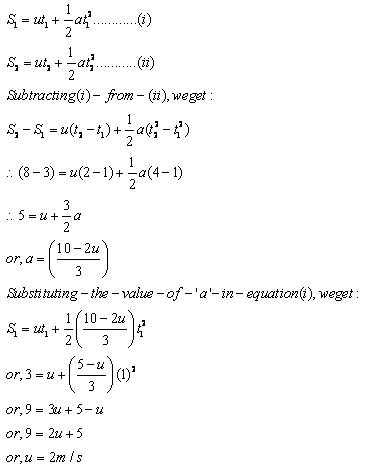
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 24

(ii) Putting u = 2 m/s in the equation
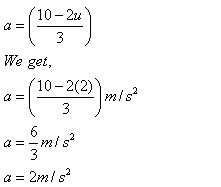
Selina Concise Physics Class 9 ICSE Solutions Motion in One Dimension - 25

Solution 13N.

Initial velocity u = 25 m/s
Final velocity v = 0

(i) Before the brakes are applied, let S be the distance travelled.
Distance = Speed × time
S = (25) × (5) m
S = 125 m

(ii) Acceleration = (Final velocity – Initial velocity)/Time taken
= [(0 – 25)/15] ms^-2
= (-5/2) ms^-2 = -2.5 ms^-2Therefore, retardation = 2.5 ms^-2

(iii) After applying brakes, the time taken to come to stop = 10 s
Let S’ be the distance travelled after applying the brakes.
Initial velocity u = 25 m/s
Final velocity v = 0
Using the third equation of motion,
v² – u² = 2as
We get,
(0)² – (25)² = 2 (-2.5) (S’)
625 = 5(S’)
S’ = 125 m

Solution 14N.

Given, the initial velocity u = 75 km/s
Final velocity v = 120 km/s
Time taken = 6 s

(i) Acceleration = (Final velocity – Initial velocity)/time taken
= [(120 – 75)/6] kms^-2
= (45/6) kms^-2 = 7.5 kms^-2

(ii) Distance travelled by the aircraft in the first 10 s = Distance travelled in the first 6 s + Distance travelled in the next 4 s.
Distance travelled in the first 6s (S₁) = ut + (1/2) at²(S₁) = ut + (1/2) at²(S₁) = (75)(6) + (1/2) (7.5)(6)²(S₁) = 450 + 135
(S₁) = 585 km

Distance travelled in the next 4 s (S₂) = Speed × time
Speed at the end of 6 s is 120 km/s.
(S₂) = (120) (4)
(S₂) = 480 km
Thus, the distance travelled by the aircraft in the first 10 s = (S₁) + (S₂) = 585 + 480 = 1065 km.

Solution 15N.

(i) For the first 10 s, initial velocity u = 0
Acceleration a = 2 m/s²Time taken t = 10 s
Let ‘v’ be the maximum velocity reached.
Using the first equation of motion
v = u + at
We get
V = (0) + (2) (10) = 20 m/s

(ii) For the last 50 s: Final velocity = 0 m/s, initial velocity = 20 m/s.
Acceleration = (Final velocity – Initial velocity)/time
= (0 – 20)/50 = -0.4 m/s²Retardation = 0.4 m/s²

(iii) Total distance travelled = Distance travelled in the first 10 s + Distance travelled in 200 s + Distance travelled in last 50 s
Distance travelled in first 10s (s₁) = ut + (1/2) at²S₁= (0) + (1/2) (2) (10)²S₁= 100 m
Distance travelled in 200s (s₂) = speed × time
S₂ = (20) (200) = 4000 m

Distance travelled in last 50s (s₃) = ut + (1/2) at²Here, u = 20 m/s, t = 50 s and a = -0.4 m/s²S₃= (20)(50) + (1/2) (-0.4) (50)²S₃= 1000 – 500
S₃= 500 m
Therefore, total distance travelled = S₁+ S₂+ S₃ = 100 + 4000 + 500 = 4600 m

(iv) Average velocity = Total distance travelled/total time taken
= (4600/260) m/s
= 17.69 m/s

More Resources for Selina Concise Class 9 ICSE Solutions

Selina Concise Chemistry Class 9 ICSE Solutions The Language of Chemistry

December 3, 2020August 24, 2018 by Veerendra

Selina Concise Chemistry Class 9 ICSE Solutions The Language of Chemistry

ICSE Solutions Selina ICSE Solutions

APlusTopper.com provides step by step solutions for Selina Concise ICSE Solutions for Class 9 Chemistry Chapter 1 Language of Chemistry. You can download the Selina Concise Chemistry ICSE Solutions for Class 9 with Free PDF download option. Selina Publishers Concise Chemistry for Class 9 ICSE Solutions all questions are solved and explained by expert teachers as per ICSE board guidelines.

Download Formulae Handbook For ICSE Class 9 and 10

Selina ICSE Solutions for Class 9 Chemistry Chapter 4 The Language of Chemistry

Page No: 8

Question 1.
What is a symbol? What information does it convey?
Solution:
A symbol is the short form which stands for the atom of a specific element or the abbreviations used for the names of elements.

It represents a specific element.
It represents one atom of an element.
A symbol represents how many atoms are present in its one gram (gm) atom.
It represents the number of times an atom is heavier than one atomic mass unit (amu) taken as a standard.

Question 2
Why is the symbol S for sulphur, but Na for sodium and Si for silicon?
Solution:
In most cases, the first letter of the name of the element is taken as the symbol for that element and written in capitals (e.g. for sulphur, we use the symbol S). In cases where the first letter has already been adopted, we use a symbol derived from the Latin name (e.g. for sodium/Natrium, we use the symbol Na). In some cases, we use the initial letter in capital together with a small letter from its name (e.g. for silicon, we use the symbol Si).

Question 3.
Write the full form of IUPAC. Name the elements represented by the following symbols:
Au, Pb, Sn, Hg
Solution:
The full form of IUPAC is International Union of Pure and Applied Chemistry.
Names of the elements:
Au – Gold
Pb – Lead
Sn – Tin
Hg – Mercury

Question 4.
If the symbol for Cobalt, Co, were written as CO, what would be wrong with it?
Solution:
Co stands for Cobalt. If we write CO, then it would mean that it is a compound containing two non-metal ions, i.e. carbon and oxygen, which forms carbon monoxide gas.

Question 5(d).
2H₂
Solution:
(a) H stands for one atom of hydrogen.
(b) H₂ stands for one molecule of hydrogen.
(c) 2H stands for two atoms of hydrogen.

Question 6.
What is meant by atomicity? Name the diatomic element.
Solution:
The number of atoms of an element that join together to form a molecule of that element is known as its atomicity. Diatomic molecules: H₂, O₂, N₂, Cl₂

Question 7(a).
Explain the terms ‘valency‘ and ‘variable valency‘.
Solution:

Valency of Na is +1 because it can lose one electron.
Valency of O is -2 because it can accept two electrons.

Variable valency: It is the combining capacity of an element in which the metal loses more electrons from a shell next to a valence shell in addition to electrons of the valence shell.

Question 7(b).
How are the elements with variable valency named? Explain with an example.
Solution:
If an element exhibits two different positive valencies, then

for the lower valency, use the suffix -OUS at the end of the name of the metal
for the higher valency, use the suffix -IC at the end of the name of the metal.

Example:

Element	Lower valency	Higher valency
Ferrum (Iron)	Ferrous (Fe²⁺)	Ferric (Fe³⁺)

Question 8.
Give the formula and valency of:

aluminate ………………… .
chromate ………….…….. .
aluminium ………………. .
cupric ………………… .

Solution:

	Name	Formula	Valency
a.	Aluminate	AlO₂	-2
b.	Chromate	CrO₄	-2
c.	Aluminium	Al	+3
d.	Cupric	Cu	+2

Question 9.b
What is the significance of formula?
Solution:
Chemical formula: The chemical formula of a substance (element or compound) is a symbolic representation of the actual number of atoms present in one molecule of that substance.
It also indicates the fixed proportion by weight in which atoms combine.
Rules:
(i) The positive and negative radicals are written side by side (+ve first) with their charge as a superscript on the right side.
(ii) Charges are then interchanged and written as a subscript.
(iii) The final formula is written without the sign of charge, e.g. Hg₂O

Hg¹⁺O^2-
Hg₂O

Question 10(a).
What do you understand by the following terms?
Acid radical
Solution:
Acid radical: The electronegative or negatively charged radical is called an acid radical.
Examples: Cl^–, O^2-

Question 10(b).
What do you understand by the following terms? Basic radical
Solution:
Basic radical: The electropositive or positively charged radical is called a basic radical.
Examples: K⁺, Na⁺

Question 11.

Match the following:

Compound	Formula
(a) Boric acid	i. NaOH
(b) Phosphoric acid	ii. SiO₂
(c) Nitrous acid	iii. Na₂CO₃
(d) Nitric acid	iv. KOH
(e) Sulphurous acid	v. CaCO₃
(f) Sulphuric acid	vi. NaHCO₃
(g) Hydrochloric acid	vii. H₂S
(h) Silica (sand)	viii. H₂O
(i) Caustic soda (sodium hydroxide)	ix. PH₃
(j) Caustic potash (potassium hydroxide)	x. CH₄
(k) Washing soda(sodium carbonate)	xi. NH₃
(l) Baking soda(sodium bicarbonate)	xii. HCl
(m) Lime stone.(calcium carbonate)	xiii. H₂SO₃
(n) Water	xiv. HNO₃
(o) Hydrogen sulphide	xv. HNO₂
(p) Ammonia	xvi. H₃BO₃
(q) Phosphine	xvii. H₃PO₄
(r) Methane	xviii. H₂SO₄

Solution:

Compound	Formula (Ans)
(a) Boric acid	xvi. H₃BO₃
(b) Phosphoric acid	xvii. H₃PO₄
(c) Nitrous acid	xv. HNO₂
(d) Nitric acid	xiv. HNO₃
(e) Sulphurous acid	xiii. H₂SO₃
(f) Sulphuric acid	xviii. H₂SO₄
(g) (a) Hydrochloric acid	xii. HCl
(h) Silica (sand)	ii. SiO₂
(i) Caustic soda (sodium hydroxide)	i. NaOH
(j) Caustic potash (potassium hydroxide)	iv. KOH
(k) Washing soda (sodium carbonate)	iii. Na₂CO₃
(l) Baking soda (sodium bicarbonate)	vi. NaHCO₃
(m) Lime stone (calcium carbonate)	v. CaCO₃
(n) Water	viii. H₂O
(o) Hydrogen sulphide	vii. H₂S
(p) Ammonia	xi. NH₃
(q) Phosphine	ix. PH₃
(r) Methane	x. CH₄

Question 12.
Select the basic and acidic radicals in the following compounds.

MgSO₄
(NH₄)₂SO₄
Al₂(SO₄)₃
ZnCO₃
Mg(OH)₂

Solution:

	Acidic radical	Basic radical
MgSO₄	SO₄^–	Mg⁺
(NH₄)₂SO₄	SO₄^–	NH₄⁺
Al₂(SO₄)₃	SO₄^–	Al³⁺
ZnCO₃	CO₃^–	Zn²⁺
Mg(OH)₂	OH^–	Mg²⁺

Question 13.
Write chemical formula of the sulphate of Aluminium, Ammonium and Zinc.
Solution:
Valencies of aluminium, ammonium and zinc are 3, 1 and 2, respectively.
The valency of sulphate is 2.
Hence, chemical formulae of the sulphates of aluminium, ammonium and zinc are Al₂(SO₄)₃, (NH₄)₂SO₄and ZnSO₄.

Question 14.
The valency of an element A is 3 and that of element B is 2. Write the formula of the compound formed by the combination of A and B
Solution:
Formula of the compound = A₂B₃

Question 15.
Write the chemical names of the following compounds:

Ca₃(PO₄)₂
K₂CO₃
K₂MnO₄
Mn₃(BO₃)₂
Mg(HCO₃)₂
Na₄Fe(CN)₆
Ba(ClO₃)₂
Ag₂SO₃
(CH₃COO)₂Pb
Na₂SiO₃

Solution:
Chemical names of compounds:

Ca₃(PO₄)₂ – Calcium phosphate
K₂CO₃ – Potassium carbonate
K₂MnO₄– Potassium manganate
Mn₃(BO₃)₂ – Manganese (II) borate
Mg(HCO₃)₂ – Magnesium hydrogen carbonate
Na₄Fe(CN)₆ – Sodium ferrocyanide
Ba(ClO₃)₂ – Barium chlorate
Ag₂SO₃ – Silver sulphite
(CH₃COO)₂Pb – Lead acetate
Na₂SiO₃ – Sodium silicate

Question 16
Write the basic radicals and acidic radicals of the following and then write the chemical formulae of these compounds.

Barium sulphate
Bismuth nitrate
Calcium bromide
Ferrous sulphide
Chromium sulphate
Calcium silicate
Potassium ferrocyanide
Stannic oxide
Magnesium phosphate
Sodium zincate
Stannic phosphate
Sodium thiosulphate
Potassium manganate
Nickel bisulphate

Solution:

Compounds	Acidic radical	Basic radical	Chemical formulae
Barium sulphate	SO₄^2-	Ba²⁺	BaSO₄
Bismuth nitrate	NO₃^–	Bi³⁺	Bi(NO₃)₃
Calcium bromide	Br^–	Ca²⁺	CaBr₂
Ferrous sulphide	S^2-	Fe²⁺	FeS
Chromium sulphate	SO₄^2-	Cr³⁺	Cr₂(SO₄)₃
Calcium silicate	SiO₄^2-	Ca²⁺	Ca₂SiO₄
Potassium ferrocyanide	[Fe(CN)₆]^4-	K¹⁺	K₄[Fe(CN)₆]
Stannic oxide	O^2-	Sn²⁺	SnO₂
Magnesium phosphate	(PO₄)^3-	Mg²⁺	Mg₃(PO₄)₂
Sodium zincate	ZnO^2-	Na¹⁺	Na₂ZnO₂
Stannic phosphate	(PO₄)^3-	Sn⁴⁺	Sn₃(PO₄)₄
Sodium thiosulphate	(S₂O₃)^2-	Na¹⁺	Na₂S₂O₃
Potassium manganate	MnO₄^2-	K¹⁺	K₂MnO₄
Nickel bisulphate	HSO₄^1-	Ni³⁺	Ni(HSO₄)₃

Question 16.
Give the names of the following compounds.

NaClO
NaClO₂
NaClO₃
NaClO₄

Solution:

NaClO – Sodium hypochlorite
NaClO₂ – Sodium chlorite
NaClO₃ – Sodium chlorate
NaClO₄ – Sodium perchlorate

Question 18(a).
Complete the following statements by selecting the correct option :
The formula of a compound represents
i. an atom
ii. a particle
iii. a molecule
iv. a combination
Solution:
iii. The formula of a compound represents a molecule.

Question 18(b).
Complete the following statements by selecting the correct option :
The correct formula of aluminium oxide is
i. AlO₃ii. AlO₂ iii. Al₂O₃
Solution:
iii. The correct formula of aluminium oxide is Al₂O₃.

Question 18(c).
Complete the following statements by selecting the correct option :
The valency of nitrogen in nitrogen dioxide (NO₂) is
i. one
ii. two
iii. three
iv. four
Solution:
iv. The valency of nitrogen in nitrogen dioxide (NO₂) is four.

Page No: 13

Question 1.
Balance the following equations:

Fe + H₂O → Fe₃O₄ + H₂
Ca + N₂ → Ca₃N₂
Zn + KOH → K₂ZnO₂ + H₂
Fe₂O₃ + CO → Fe + CO₂
PbO + NH₃ → Pb + H₂O + N₂
Pb₃O₄ → PbO + O₂
PbS + O₂ → PbO + SO₂
S + H₂SO₄→ SO₂ + H₂O
S + HNO₃ → H₂SO₄ + NO₂ + H₂O
MnO₂ + HCl → MnCl₂ + H₂O + Cl₂
C + H₂SO₄ → CO₂ + H₂O + SO₂
KOH + Cl₂ → KCl + KClO + H₂O
NO₂ +H₂O → HNO₂ + HNO₃
Pb₃O₄ + HCl → PbCl₂ + H₂O + Cl₂
H₂O + Cl₂ → HCl + O₂
NaHCO₃ → Na₂CO₃ + H₂O + CO₂
HNO₃ + H₂S → NO₂ + H₂O + S
P + HNO₃ → NO₂ + H₂O + H₃PO₄
Zn + HNO₃ → Zn(NO₃)₂ + H₂O + NO₂

Solution:
Balanced chemical equations:

3Fe + 4H₂O → Fe₃O₄ + 4H₂
3Ca + N₂ → Ca₃N₂
Zn + 2KOH → K₂ZnO₂ + H₂
Fe₂O₃ + 3CO → 2Fe + 3CO₂
3PbO + 2NH₃ → 3Pb + 3H₂O + N₂
2Pb₃O₄ → 6PbO + O₂
2PbS + 3O₂ → 2PbO + 2SO₂
S + 2H₂SO₄→ 3SO₂ + 2H₂O
S + 6HNO₃ → H₂SO₄ + 6NO₂ + 2H₂O
MnO₂ + 4HCl → MnCl₂ + 2H₂O + Cl₂
C + 2H₂SO₄ → CO₂ + H₂O + SO₂
2KOH + Cl₂ → KCl + KClO + H₂O
2NO₂ + H₂O → HNO₂ + HNO₃
Pb₃O₄ + 8HCl → 3PbCl₂ + 4H₂O + Cl₂
2H₂O + 2Cl₂ → 4HCl + O₂
2NaHCO₃ → Na₂CO₃ + H₂O + CO₂
2HNO₃ + H₂S → 2NO₂ + 2H₂O + S
P + 5HNO₃ → 5NO₂ + H₂O + H₃PO₄
Zn + 4HNO₃ → Zn(NO₃)₂ + 2H₂O + 2NO₂

Page No: 17

Question 1.
Fill in the blanks:

Dalton used symbol _____ for oxygen _____ for hydrogen.
Symbol represents _____ atom(s) of an element.
Symbolic expression for a molecule is called _____. .
Sodium chloride has two radicals. Sodium is a _____ radical while chloride is _____ radical.
Valency of carbon in CH₄ is _____ , in C₂H₆_____, in C₂H₄ ___ and in C₂H₂ is ____.
Valency of Iron in FeCl₂ is _____ and in FeCl₃ it is ____ .
Formula of iron (ill) carbonate is _____ .

Solution:

Dalton used symbol [O] for oxygen,[H] for hydrogen.
Symbol represents gram atom(s) of an element.
Symbolic expression for a molecule is called molecular formula.
Sodium chloride has two radicals. Sodium is a basic radical, while chloride is an acid radical.
Valency of carbon in CH₄ is 4, in C₂H₆4, in C₂H₄4 and in C₂H₂ is 4.
Valency of iron in FeCl₂ is 2 and in FeCl₃ it is 3.
Formula of iron (III) carbonate is Fe₂[CO₃]₃.

Question 2.
Complete the following table.

Acid Radicals Basic Radicals	Chloride	Nitrate	Sulphate	Carbonate	Hydroxide	Phosphate
Magnesium	MgCl₂	Mg(NO₃)₂	MgSO₄	MgCO₃	Mg(OH)₂	Mg₃(PO₄)₂
Sodium
Zinc
Silver
Ammonium
Calcium
Iron (II)
Potassium

Solution:

Selina Concise Chemistry Class 9 ICSE Solutions The Language of Chemistry image - 3

Question 3.
Sodium chloride reacts with silver nitrate to produce silver chloride and sodium nitrate

Write the equation.
Check whether it is balanced, if not balance it.
Find the weights of reactants and products.
State the law which this equation satisfies.

Solution:
(a) NaCl+ AgNO₃ → NaNO₃ + AgCl↓
(b) It is a balanced equation.
(c) Weights of reactants:NaCl – 58.44, AgNO₃ – 169.87
Weights of products: NaNO₃ – 84.99, AgCl – 143.32
NaCl + AgNO₃ → NaNO₃ + AgCl
(23+35.5) + (108+14+48) → (23+14+48) + (108+35.5)
58.5 + 170 → 85 + 143.5
228.5 g → 228.5 g
(d) Law of conservation of mass: Matter is neither created nor destroyed in the course of a chemical reaction.

Question 4(a).
What information does the following chemical equation convey? Zn + H₂SO₄ → ZnSO₄+ H₂Solution:
(a) This equation conveys the following information:

The actual result of a chemical change.
Substances take part in a reaction, and substances are formed as a result of the reaction.
Here, one molecule of zinc and one molecule of sulphuric acid react to give one molecule of zinc sulphate and one molecule of hydrogen.
Composition of respective molecules, i.e. one molecule of sulphuric acid contains two atoms of hydrogen, one atom of sulphur and four atoms of oxygen.
Relative molecular masses of different substances, i.e. molecular mass of
Zn = 65
H₂SO₄ = (2+32+64) = 98
ZnSO₄= (65+32+64) = 161
H₂ = 2
22.4 litres of hydrogen are formed at STP.

Question 4(b).
What information do the following chemical equations convey? Mg + 2HCl → MgCl₂+ H₂Solution:
(b) This equation conveys the following information:

Magnesium reacts with hydrochloric acid to form magnesium chloride and hydrogen gas.
24 g of magnesium reacts with 2(1 + 35.5) = 73 g of hydrochloric acid to produce (24 + 71), i.e. 95 g of magnesium chloride.
Hydrogen produced at STP is 22.4 litres.

Question 5(a).
What are polyatomic ions? Give two examples.
Solution:
(a) A poly-atomic ion is a charged ion composed of two or more atoms covalently bounded that can be carbonate (CO₃^2-)and sulphate (SO₄^2-)

Question 5(b).
Name the fundamental law that is involved in every equation.
Solution:
(b) The fundamental laws which are involved in every equation are:

A chemical equation consists of formulae of reactants connected by plus sign (+) and arrow (→) followed by the formulae of products connected by plus sign (+).
The sign of an arrow (→) is to read ‘to form’. It also shows the direction in which reaction is predominant.

Question 6(a).
What is the valency of : fluorine in CaF₂
Solution:
(a) Valency of fluorine in CaF₂is -1.

Question 6(b).
What is the valency of :
sulphur in SF₆Solution:

(b) Valency of sulphur in SF₆ is -6.

Question 6(c).

What is the valency of :

phosphorus in PH₃
Solution:
(c) Valency of phosphorus in PH₃ is +3.

Question 6(d).
What is the valency of : carbon in CH₄

Solution:

(d) Valency of carbon in CH₄ is +4.

Question 6(e).
What is the valency of :
nitrogen in the following compounds:
(i) N₂O₃ (ii) N₂O₅ (iii) NO₂ (iv) NO
Solution:
(e) Valency of nitrogen in the given compounds:

N₂O₃ = N is +3
N₂O₅= N is +5
NO₂ = N is +4
NO = N is +2

Question 7.
Why should an equation be balanced? Explain with the help of a simple equation.
Solution:
According to law of conservation of mass, “matter can neither be created nor be destroyed in a chemical reaction”. This is possible only, if total number of atoms on the reactants side is equals to total number of atoms on products side. Thus, a chemical reaction should be always balanced.
Let us consider an example,
Fe + H₂O → Fe₃O₄ + H₂In this equation number of atoms on both sides is not the same, the equation is not balanced.
The balanced form of this equation is given by,
3Fe + 4H₂O → Fe₃O₄ + 4H₂

Question 8(a).
Write the balanced chemical equations of the following reactions. sodium hydroxide + sulphuric acid → sodium sulphate + water
Solution:
2NaOH + H₂SO₄ → Na₂SO₄ + 2H₂O

Question 8(b).
Write the balanced chemical equations of the following reactions. potassium bicarbonate + sulphuric acid → potassium sulphate + carbon dioxide + water
Solution:
2KHCO₃ + H₂SO₄ → K₂SO₄ + 2CO₂ + 2H₂O

Question 8(c).
Write the balanced chemical equations of the following reactions. iron + sulphuric acid → ferrous sulphate + hydrogen.
Solution:
Fe + H₂SO₄ → FeSO₄ + H₂

Question 8(d).
Write the balanced chemical equations of the following reactions. chlorine + sulphur dioxide + water → sulphuric acid + hydrogen chloride
Solution:
Cl₂ + SO₂ + 2H₂O → H₂SO₄ + 2HCl

Question 8(e).
Write the balanced chemical equations of the following reactions. silver nitrate → silver + nitrogen dioxide + oxygen”
Solution:
2AgNO₃ → 2Ag + 2NO₂ + O₂

Question 8(f).
Write the balanced chemical equations of the following reactions.
copper + nitric acid → copper nitrate + nitric oxide + water
Solution:
3Cu + 8HNO₃ → 3Cu(NO₃)₂ + 2NO + 4H₂O

Question 8(g).
Write the balanced chemical equations of the following reactions.
ammonia + oxygen → nitric oxide + water
Solution:

Question 8(h).
Write the balanced chemical equations of the following reactions.
barium chloride + sulphuric acid → barium sulphate + hydrochloric acid
Solution:
BaCl₂ + H₂SO₄ → BaSO₄ + 2HCl

Question 8(i).
Write the balanced chemical equations of the following reactions.
zinc sulphide + oxygen → zinc oxide + sulphur dioxide
Solution:
2ZnS + 3O₂ → 2ZnO + 2SO₂

Question 8(j).
Write the balanced chemical equations of the following reactions.
aluminium carbide + water → aluminium hydroxide + methane
Solution:
Al₄C₃ + 12H₂O → 4Al(OH)₃ + 3CH₄

Question 8(k).
Write the balanced chemical equations of the following reactions.
iron pyrites(FeS₂) + oxygen → ferric oxide + sulphur dioxide
Solution:
4FeS₂ + 11O₂ → 2Fe₂O₃ + 8SO₂

Question 8(l).
Write the balanced chemical equations of the following reactions.
potassium permanganate + hydrochloric acid → potassium chloride + manganese chloride + chlorine + water
Solution:
2KMnO₄ + HCl → 2KCl + 2MnCl₂ + 5Cl₂ + 8H₂O

Question 8(m).
Write the balanced chemical equations of the following reactions.
aluminium sulphate + sodium hydroxide → sodium sulphate + sodium meta aluminate + water.
Solution:
Al₂(SO₄)₃+ 8NaOH → 3Na₂SO₄ + 2NaAlO₂ + 4H₂O

Question 8(n).
Write the balanced chemical equations of the following reactions.
aluminium + sodium hydroxide + water → sodium meta aluminate + hydrogen
Solution:
2Al + 2NaOH + 2H₂O → 2NaAlO₂ + 3H₂

Question 8(o).
Write the balanced chemical equations of the following reactions.
potassium dichromate + sulphuric acid → potassium sulphate + chromium sulphate + water + oxygen.
Solution:
2K₂Cr₂O₇ + 8H₂SO₄ → 2K₂SO₄ + 2Cr₂(SO₄)₃ + 8H₂O + 3O₂

Question 8(p).
Write the balanced chemical equations of the following reactions.
potassium dichromate + hydrochloric acid → Potassium chloride + chromium chloride + water + chlorine
Solution:
K₂Cr₂O₇ + 14HCl → 2KCl + 2CrCl₃ + 7H₂O + 3Cl₂

Question 8(q).
Write the balanced chemical equations of the following reactions.
sulphur + nitric acid → sulphuric acid + nitrogen dioxide + water.
Solution:
S + HNO₃ → H₂SO₄ + NO₂ + H₂O

Question 8(r).
Write the balanced chemical equations of the following reactions.
sodium chloride + manganese dioxide + sulphuric acid → sodium hydrogen sulphate + manganese sulphate + water + chlorine.
Solution:
2NaCl + MnO₂ + 3H₂SO₄ → 2NaHSO₄ + MnSO₄ + 2H₂O + Cl₂

Question 9(a).
Define atomic mass unit.
Solution:
Atomic mass unit (amu) is equal to one-twelfth the mass of an atom of carbon-12 (atomic mass of carbon taken as 12).

Question 9(b)(ii)
Calculate the molecular mass of the following:
(NH₄)₂CO₃
Given atomic mass of Cu = 63·5, H = 1, O= 16, C = 12, N = 14, Mg = 24, S = 32
Solution:
Molecular mass of (NH₄)₂CO₃= (2 × 14) + (8 × 1) + 12 + (3 × 16)
= 28 + 8 + 12 + 48
= 96

Question 9(b)(iii)
Calculate the molecular mass of the following:
(NH₂)₂CO
Given atomic mass of Cu = 63·5, H = 1, O= 16, C = 12, N = 14, Mg = 24, S = 32
Solution:
Molecular mass of (NH₂)₂CO
= (14 × 2) + (4 × 1) + 12 + 16
= 28 + 4 + 12 + 16
= 60

Question 9(b)(iv)
Calculate the molecular mass of the following:
Mg₃N₂Given atomic mass of Cu = 63·5, H = 1, O = 16, C = 12, N = 14, Mg = 24, S = 32
Solution:
Molecular mass of Mg₃N₂= (3 × 24) + (2 × 14)
= 72 + 28
= 100

Question 10(a).
Choose the correct answer from the options given below.
Modern atomic symbols are based on the method proposed by
i. Bohr
ii. Dalton
iii. Berzelius
iv. Alchemist
Solution:
iii. Berzelius

Question 10(b).
Choose the correct answer from the options given below.
The number of carbon atoms in a hydrogen carbonate radical is
i. One
ii. Two
iii. Three
iv. Four
Solution:
One

Question 10(c).
Choose the correct answer from the options given below.
The formula of iron (III) sulphate is
i. Fe₃SO₄
ii. Fe(SO₄)₃iii. Fe₂(SO₄)₃iv. FeSO₄
Solution:
iii. Fe₂(SO₄)₃

Question 10(d).
Choose the correct answer from the options given below.
In water, the hydrogen-to-oxygen mass ratio is
i. 1: 8
ii. 1: 16
iii. 1: 32
iv. 1: 64
Solution:
i. 1:8

Question 10(e).
Choose the correct answer from the options given below.
The formula of sodium carbonate is Na₂CO₃ and that of calcium hydrogen carbonate is
i. CaHCO₃ii. Ca(HCO₃)₂iii. Ca₂HCO₃iv. Ca(HCO₃)₃Solution:
i. Ca(HCO₃)₂

Solution 11.
(a) A molecular formula represent The Molecule of an element or of a Compound.
(b) The molecular formula of water (H₂O) represents 18 parts by mass of water.
(c) A balanced equation obeys the law of conservation of mass wherever unbalanced equation does not obey this law.
(d) CO and Co represent carbon-monoxide and cobalt respectively.

Solution 12.

Relative molecular mass of CHCl3
= 12 + 1 + (3 × 35.5)
= 12 + 1 + 106.5
= 119.5
Relative molecular mass of (NH4)2 Cr2O7
= (14 × 2) + (1× 8) + (52 × 2) + (16 × 7)
= 28 + 8 + 104 + 112
= 252
Relative molecular mass of CuSO4· 5H2O
= 63.5 + 32 + (16 × 4) + 5(2 + 16)
= 159.5 + 90
= 249.5
Relative molecular mass of (NH4)2SO4
= (2 × 14) + (8 × 1) + 32 + (4 × 16)
= 28 + 8 + 32 + 64
= 132
Relative molecular mass of CH3COONa
= (12 × 2) + (1× 3) + (16 × 2) + 23
= 24 + 3 + 32 + 23
= 82
Potassium chlorate (KClO3)
= 39.1+ 35.5 + (16 × 3)
= 39.1+ 35.5 + 48
= 122.6
Ammonium chloroplatinate (NH4)2PtCl6
= (14 × 2) + (1 × 8) + 195.08 + (35.5 × 6)
= 28 + 8 + 195.08 + 213
= 444.08

Solution 13.

Compound	Empirical formula
(a) Benzene (C₆H₆)	CH
CompoundEmpirical formula (b) Glucose (C₆H₁₂O₆)	CH₂O
CompoundEmpirical formula (c) Acetylene (C₂H₂)	CH
CompoundEmpirical formula (d) Acetic acid (CH₃COOH)	CH₂O

Solution 14.
Relative molecular mass of MgSO₄·7H₂O
=24 + 32 + (16 × 4) + 7(2 + 16)
=24 + 32 + 64 + 126
=246
26 g of Epsom salt contains 126 g of water of crystallisation.
Hence, 100 g of Epsom salt contains
Selina Concise Chemistry Class 9 ICSE Solutions The Language of Chemistry image - 5
The % of H₂O in MgSO₄·7H₂O = 51.2

Solution 15.
(a) Relative molecular mass of Ca(H₂PO₄)₂= 40.07 + (1 × 4) + (30.9 × 2) + (16 × 8)
= 40.07 + 4 + 61.8 + 128
= 233.87
233.87 g Ca(H₂PO₄)₂contains 61.8 g P
So, 100 g Ca(H₂PO₄)₂contains

The % of P in Ca(H₂PO₄)₂ is 26.42%.
(b) Relative molecular mass of Ca₃(PO₄)₂= (40.07 × 3) + (30.9 × 2) + (16 × 8)
= 120.21 + 61.8 + 128
= 310.01
310.01 g Ca₃(PO₄)₂contains 61.8 g P
So, 100 g Ca(H₂PO₄)₂contains
(IMAGE)
The % of P in Ca(H₂PO₄)₂ is 19.93%.

Solution 16.
Relative molecular mass of KClO₃= 39.09 + 35.5 + (3 × 16)
= 122.59 g
Selina Concise Chemistry Class 9 ICSE Solutions The Language of Chemistry image - 7
The percentages of K, Cl and O in KClO₃ are 31.9%, 28.9% and 39.1%, respectively.

Solution 17.
Relative molecular mass of urea is

Element	No. of atoms	Atomic mass	Total
N	2	14	28
C	1	12	12
H	4	1	4
O	1	16	16

[12 + 16 + 28 + 4] = 60
Hence, relative molecular mass of urea = 60
Selina Concise Chemistry Class 9 ICSE Solutions The Language of Chemistry image - 8

More Resources for Selina Concise Class 9 ICSE Solutions

Selina Concise Biology Class 9 ICSE Solutions Tissues: Plant And Animal Tissues

August 25, 2018August 24, 2018 by Veerendra

Selina Concise Biology Class 9 ICSE Solutions Tissues: Plant And Animal Tissues

APlusTopper.com provides step by step solutions for Selina Concise ICSE Solutions for Class 9 Biology Chapter 3 Tissues: Plant And Animal Tissues. You can download the Selina Concise Biology ICSE Solutions for Class 9 with Free PDF download option. Selina Publishers Concise Biology for Class 9 ICSE Solutions all questions are solved and explained by expert teachers as per ICSE board guidelines.

Download Formulae Handbook For ICSE Class 9 and 10

ICSE Solutions Selina ICSE Solutions

Exercise 1

Solution A.

(c) Parenchyma
(a) Fibrous connective tissue
(a)Meristem- Actively dividing cells

Solution B.1.

(a) Apical or terminal meristematic tissue
(b) Protective tissue
(c) Columnar epithelium (Epithelial tissue)
(d) Ligament (Connective tissue)
(e) Conducting tissue
(f) Sclerenchyma (Supporting tissue)

Solution B.2.

Sclerenchyma composed of long, narrow and thick cells, which have become dead, forms the least specialized tissue in plants. This tissue forms the walls and boundaries of plant cells and provides strength to tissue plant parts.

Solution B.3.

(a) Tissue
(b) Permanent tissue cells
(c) Cambium

Solution B.4.

(a) Tips of roots
(b) Nose
(c) Lining of mouth
(d) Veins of leaves
(e) Lining of trachea
(f) Bones

Solution B.5.

(a) Squamous epithelium
(b) Cuboidal epithelium
(c) Neuron
(d) Ciliated columnar epithelium

Solution C.1.

Ciliated columnar epithelium is found in the lining of trachea. This epithelium has thread-like projections called cilia at their free ends. The cilia constantly keep lashing and move the materials which enter this organ.

Solution C.2.

Nervous tissue or neurons are specialized group of cells. This tissue is concerned with perception and responses of animals.
The nervous tissue constitutes the nervous system, which is an organ system. It controls and coordinates all the systems of the body.

Solution C.3.

Muscular tissue (Cardiac muscles)
Epithelial tissue (Lining of blood vessels of the heart)
Connective tissue (Fluid connective tissue in the form of red blood corpuscles)

Solution C.4.

A tissue is a group of similar cells from the same origin that together carry out a specific function. An egg is a zygote or a cell but a cluster of eggs cannot be considered as a tissue as it does not form an organ like a tissue. Instead it gives rise to a new individual organism if gets fertilised.

Solution C.5.

Striated muscles: Provide the force for locomotion and all voluntary movements of the body. These muscles are found in the limbs.
Unstriated muscles: Provide movements for the passage of food in the intestines. These muscles are found in iris of the eye, lining of blood vessels, urinary bladder, etc.
Cardiac muscles: Provide rhythmic contraction and relaxation movements. These muscles are found only in the heart.

Solution D.1.

(a) Cell and tissue

Cell	Tissue
A cell is the structural and functional unit of all living beings. E.g. epithelial cell	A tissue is a group of similar cells which perform a specific function. E.g. nervous tissue

(b) Organ and organism

Organ	Organism
Several tissues together contribute to specific functions inside the body and constitute an organ. E.g. stomach	Several organ systems together constitute the organism. E.g. human being

Organ	Organelle
Several tissues together contribute to specific functions inside the body and constitute an organ. E.g. stomach	Parts of the cell that have a definite function in the cell. E.g. mitochondria

(d) Organ and organ system

Organ	Organ system
Several tissues together contribute to specific functions inside the body and constitute an organ. E.g. stomach	Many organs act together to perform a specific life process and constitute an organ system. E.g. digestive system

Solution D.2.

(a) Parenchyma and Collenchyma

Parenchyma	Collenchyma
(i) Consists of large thin-walled living cells with a single large vacuole (ii) Intercellular spaces may or may not be present	(i) Consists of elongated cells having thickening in their cell walls (ii) Intercellular spaces are totally absent

(b) Meristematic tissue and Permanent tissue

Meristematic tissue	Permanent tissue
(i) Have the capacity to divide (ii) Do not have intercellular spaces	(i) Have lost the capacity to divide (ii) Have large intercellular spaces

Sclerenchyma	Parenchyma
(i) Consist of dead cells (ii) Have thick cell walls	(i) Consist of living cells (ii) Have thin cell walls

(d) Cells of involuntary muscle and voluntary muscle

Cells of involuntary muscle	Cells of voluntary muscle
(i) Small and spindle-shaped	(i) Long and cylindrical
(ii) Uninucleate	(ii) Multinucleate
(iii) Lack stripes or striations	(iii) Show stripes or striations
(iv) Found in the walls of the intestine and lining of blood vessels	(iv) Found in the arms, legs, face and neck

(e) Fibres of voluntary muscle and cardiac muscle

Fibres of voluntary muscle	Fibres of cardiac muscle
(i) Long and cylindrical	(i) Short and branched
(ii) Multinucleate	(ii) Uninucleate
(iii) Under the control of one’s own will or volition	(iii) Not under the control of one’s own will or volition
(iv) Found in the arms, legs, face and neck	(iv) Found in the heart

Solution E.1.

(a) The given diagram is of the phloem tissue because the cells show cellular contents unlike the xylem tissue which contains hollow cells without any cellular contents.
(b)
1 → Sieve cell
2 → Phloem parenchyma cell
3 → Companion cell
4 → Sieve plate
(c) The phloem is a food-conducting tissue and is likely to be found in the leaves and stem of plants to carry the food manufactured in the leaves to various parts of the plant.
(d)
1 → Sieve cells: Help in the transport of food from leaves to storage organs and other parts of the plant.
2 → Phloem parenchyma cells: Storage of starch, fat and other organic food material.
3 → Companion cells: Help in the functioning of the sieve tube cells.
4 → Sieve plate: Perforations in the sieve plates allow water and dissolved organic solutes to flow along the sieve tube.

Solution E.2.

(a) The given diagram shows a nerve cell or neuron.
(b)
1 → Dendrites/Dendrons
2 → Axon
3 → Nucleus
4 → Cyton/Perikaryon
5 → Neurolemma
6 → Axon endings
(c) The nerve cell is likely to be found in the nervous system of the human body. The function of the nerve cell is to transmit messages from one part of the body to another. It is associated with perception and responses of animals.

More Resources for Selina Concise Class 9 ICSE Solutions

Selina Concise Biology Class 9 ICSE Solutions Nutrition

August 25, 2018August 24, 2018 by Veerendra

Selina Concise Biology Class 9 ICSE Solutions Nutrition

APlusTopper.com provides step by step solutions for Selina Concise ICSE Solutions for Class 9 Biology Chapter 10 Nutrition. You can download the Selina Concise Biology ICSE Solutions for Class 9 with Free PDF download option. Selina Publishers Concise Biology for Class 9 ICSE Solutions all questions are solved and explained by expert teachers as per ICSE board guidelines.

Download Formulae Handbook For ICSE Class 9 and 10

ICSE Solutions Selina ICSE Solutions

Selina ICSE Solutions for Class 9 Biology Chapter 10 Nutrition

Exercise 1

Solution A.

(c) Fructose and glucose
(d) Potassium – Banana
(b) and (c). (Note: Marasmus is mainly caused due to the deficiency of proteins, but as per the options provided, the right answer would be both carbohydrates and fats.)
(a) A, D and E
(c) Carrot
(a) C

Solution B.1.

(a) T (True)
(b) F (False). Kwashiorkor is a severe protein deficiency disease.
(c) F (False). Iodine is required for the proper working of thyroid.
(d) F (False). Antibodies are proteins produced by the immune system of the body, when it detects harmful substances called antigens.
(e) T (True)

Solution B.2.

(i) Fluorine
(ii) Iodine
(iii) Iron

Solution C.1.

CARBOHYDRATES	EXAMPLES	USES
(i) Monosaccharides	1.Glucose	Provides an instant source of energy
(i) Monosaccharides	2.Fructose	Needed for maintaining a healthy body
(ii) Disaccharides	1.Sucrose	Needed for good health
(ii) Disaccharides	2.Maltose	Further broken down to produce glucose molecule which provides energy to the body
(iii) Polysaccharides	1.Cellulose	Acts as roughage which prevents constipation
(iii) Polysaccharides	2. Glycogen	Reserve carbohydrate in humans and stored in liver and muscles

Solution C.2.

Balanced diet is defined as the one which contains all the principal constituents of food in proper quantity.
Balanced diet is the one that provides at least 50% of energy from carbohydrate, 35% from fat, and 15% from protein. The precise optimal quantities of each nutrient will vary with age, sex and activity.

Solution C.3.

Bones are generally made of calcium and iron. Milk and milk products are rich in calcium and Vitamin A. That is why, a doctor advises a bone patient to include more of milk and milk products in his everyday food to make his bones and teeth strong. Milk also prevents oxidation of Vitamin A. Calcium present in milk even helps in clotting of blood. Milk, is therefore a wholesome food.

Solution D.1.

Need for food by the body:

Growth: Food is necessary for building new protoplasm or cells. This helps in the growth of an organism.
Repair: Food provides materials for the repair of worn out or damaged cells and tissues.
Energy: We obtain energy from food. This energy is required for carrying out various life functions.
Maintenance: Nutrients obtained through food help to maintain the chemical composition of cells.
Provision of raw materials: Raw materials required for the synthesis of products such as enzymes, hormones, sweat, milk, etc. are obtained through food.
Protection: Food provides protection from diseases and infection.

Solution D.2.

Proteins are the body building foods. They provide the chemical material for the growth and repair of body cells and tissues. At the time of emergency, proteins can also be oxidized in the body to release energy.
Protein deficiency disease of young children: Kwashiorkor

Solution D.3.

Whole grain atta, fruits and green leafy vegetables are the chief sources of roughage.

Roughage does not provide any nutrients to our body. It still has nutritive value and is essential for the proper functioning of the gut.
It absorbs a lot of water and retains it. In this way, it keeps faecal matter soft preventing constipation.
It combines with intestinal waste and makes it bulky.
It helps in the formation of stools and helps our body to expel the undigested waste food.
Roughage expands the intestinal lumen and helps in slow and smooth movement of food through the gastrointestinal tract. This movement is required for proper and complete digestion of food and for the elimination of intestinal waste.
Roughage stimulates secretion from the digestive tract and also helps in removal of cholesterol, fatty secretory substances and toxins from the body.

Solution E.1.

VITAMIN	RICH SOURCE	DEFICIENCY DISEASE
(i) Thiamine	Whole grain	Beri-beri
Niacin	Milk	(ii) Pellagra
(iii) Ascorbic acid	(iv) Citrus fruit	Scurvy
Calciferol	(v) Fish liver oil	(vi) Rickets
(vii) Retinol	Carrot, yellow fruit	(viii) Night blindness

Solution E.2.

Mineral	Function	Rich Source
Iodine	Promotes the secretion of thyroxine by the thyroid gland	Iodised salt, sea foods
Iron	Formation of haemoglobin	Whole cereals, fish
Calcium	Allows muscle contraction and clotting of blood	Dairy foods, beans
Potassium	Controls nerve and muscle activity, fluid balance, secretion of neurotransmitter	Banana, potato

More Resources for Selina Concise Class 9 ICSE Solutions