Number Of Tangents From A Point On A Circle

Number Of Tangents From A Point On A Circle

(i) There is no tangent passing through a point lying inside the circle.
(ii) There is one and only one tangent passing through a point lying on a circle.
(iii) There are exactly two tangents through a point lying outside a circle.
Length Of Tangent
The length of the line segment of the tangent between a given point and the given point of contact with the circle is called the length of the tangent from the point to the circle.
Results On Tangents
Theorem 1: The tangent at any point of a circle is perpendicular to the radius through the point of contact.
Given: A circle with centre O and a tangent AB at a point P of the circle.

To prove: OP ⊥ AB.
Construction: Take a point Q, other than P, on AB. Join OQ.
Proof: Q is a point on the tangent AB, other than the point of contact P.
∴ Q lies outside the circle.
Let OQ intersect the circle at R.
Then, OR < OQ        [a part is less than the whole]          …(i)
But, OP = OR           [radii of the same circle].                   ….(ii)
∴ OP < OQ                [from (i) and (ii)].
Thus, OP is shorter than, any other line segment joining O to any point of AB, other than P.
In other words, OP is the shortest distance between the point O and the line AB.
But, the shortest distance between a point and a line is the perpendicular distance.
∴ OP ⊥ AB.

Read More:

• How To Construct A Tangent To A Circle From An External Point
• RS Aggarwal Class 10 Solutions Circles

Theorem 2: (Converse of Theorem 1)
A line drawn through the end of a radius and perpendicular to it is a tangent to the circle.
Given: A circle with centre O in which OP is a radius and AB is a line through P such that OP ⊥ AB.

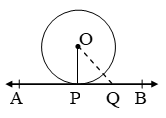
To prove: AB is a tangent to the circle at the point P.
Construction: Take a point Q, different from P, on AB. Join OQ.
Proof: We know that the perpendicular distance from a point to a line is the shortest distance between them.
∴ OP ⊥ AB ⇒ OP is the shortest distance from O to AB.
∴ OP < OQ.
∴ Q lies outside the circle
[∵ OP is the radius and OP < OQ].
Thus, every point on AB, other than P, lies outside the circle.
∴ AB meets the circle at the point P only.
Hence, AB is the tangent to the circle at the point P.

Theorem 3: The lengths of tangents drawn from an external point to a circle are equal.
Given: Two tangents AP and AQ are drawn from a point A to a circle with centre O.

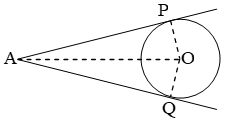
To prove: AP = AQ
Construction: Join OP, OQ and OA.
Proof: AP is a tangent at P and OP is the radius through P.
∴ OP ⊥ AP.
Similarly, OQ ⊥ AQ.
In the right triangle OPA and OQA, we have
OP = OQ                       [radii of the same circle]
OA = OA                       [common]
∴ ∆OPA ≅ ∆OQA       [by RHS–congruence]
Hence, AP = AQ.

Theorem 4: If two tangents are drawn from an external point then
(i) They subtend equal angles at the centre, and
(ii) They are equally inclined to the line segment joining the centre to that point.
Given: A circle with centre O and a point A outside it. Also, AP and AQ are the two tangents to the circle.

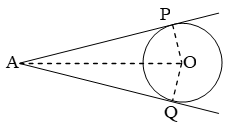
To prove: ∠AOP =∠AOQ and ∠OAP = ∠OAQ.
Proof: In ∆AOP and ∆AOQ, we have
AP = AQ             [tangents from an external point are equal]
OP = OQ             [radii of the same circle]
OA = OA            [common]
∴ ∆AOP ∆AOQ          [by SSS–congruence].
Hence, ∠AOP = ∠AOQ and ∠OAP = ∠OAQ.

Tangents From A Point On A Circle Examples

Example 1:    From a point P, 10 cm away from the centre of a circle, a tangent PT of length 8 cm is drawn. Find the radius of the circle.
Sol.    Let O be the centre of the given circle and let P be a point such that  OP = 10 cm.

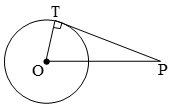
Let PT be the tangent such that PT = 8 cm.
Join OT.
Now, PT is a tangent at T and OT is the radius through T.
∴ OT ⊥ PT.
In the right ∆OTP, we have
OP² = OT² + PT²            [by Pythagoras’ theorem]
\(\Rightarrow OT=\sqrt{O{{P}^{2}}-P{{T}^{2}}}=\sqrt{{{(10)}^{2}}-{{(8)}^{2}}}\text{ cm} \)
= √36 cm = 6 cm.
Hence, the radius of the circle is 6 cm.

Example 2:    In the given figure, PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length TP.

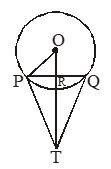
Sol.    Join OP and OT Let OT intersect PQ at a point R.
Then, TP = TQ and ∠PTR = ∠QTR.
∴ TR ⊥ PQ and TR bisects PQ.
∴ PR = RQ = 4 cm.
\( \text{Also,  OR}=\sqrt{O{{P}^{2}}-P{{R}^{2}}}=\sqrt{{{5}^{2}}-{{4}^{2}}}\text{ cm} \)
\( =\sqrt{25-16}=\sqrt{9}=3\text{ cm} \)
Let TP = x cm and TR = y cm.
From right ∆TRP, we get
TP² = TR² + PR²
⇒ x² = y² + 16 ⇒ x² – y² = 16 …. (i)
From right ∆OPT, we get
TP² + OP² = OT²
⇒ x² + 52 = (y + 3)²      [∵ OT² = (OR + RT)²]
⇒ x² – y² = 6y – 16          ….(ii)
From (i) and (ii), we get
6y – 16 = 16 ⇒ 6y = 32 ⇒ y = 16/3.
Putting y = 16/3 in (i), we get
\( {{\text{x}}^{2}}=16+{{\left( \frac{16}{3} \right)}^{2}}=\left( \frac{256}{9}+16 \right)=\frac{400}{9} \)
\( \Rightarrow x=\sqrt{\frac{400}{9}}=\frac{20}{3}  \)
Hence, length TP = x cm = 20/3 cm
= 6.67 cm.

Example 3:    Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTQ = 2∠OPQ.
Sol.    Given: A circle with centre O and an external point T from which tangents TP and TQ are drawn to touch the circle at P and Q.

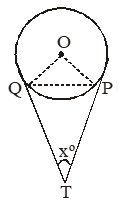
To prove: ∠PTQ = 2∠OPQ.
Proof: Let ∠PTQ = xº. Then,
∠TQP + ∠TPQ + ∠PTQ = 180º
[∵ sum of the ∠s of a triangle is 180º]
⇒ ∠TQP + ∠TPQ = (180º – x)          ….(i)
We know that the lengths of tangent drawn from an external point to a circle are equal.
So, TP = TQ.
Now, TP = TQ
⇒ ∠TQP = ∠TPQ
\( =\frac{1}{2}({{180}^{0}}-x)=\left( 90{}^\text{o}-\frac{x}{2} \right) \)
= (180º –x)=
∴ ∠OPQ = (∠OPT–∠TPQ)
\( ={{90}^{0}}-\left( 90{}^\text{o}-\frac{x}{2} \right)=\frac{x}{2}  \)
⇒ ∠OPQ = \(\frac { 1 }{ 2 }\) ∠PTQ
⇒ ∠PTQ = 2∠OPQ.

Example 4:    Prove that in two concentric circles, the chord of the larger circle which touches the smaller circle, is bisected at the point of contact.
Sol.    Given: Two circles with the same centre O and AB is a chord of the larger circle which touches the smaller circle at P.

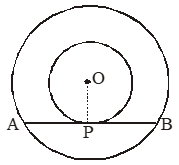
To prove: AP = BP.
Construction: Join OP.
Proof: AB is a tangent to the smaller circle at the point P and OP is the radius through P.
∴ OP ⊥ AB.
But, the perpendicular drawn from the centre of a circle to a chord bisects the chord.
∴ OP bisects AB. Hence, AP = BP.

Example 5:    Prove that the tangents drawn at the ends of a diameter of a circle are parallel.
Sol. Given: CD and EF are the tangents at the end points A and B of the diameter AB of a circle with centre O.

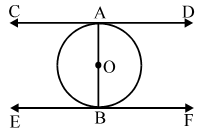
To prove: CD || EF.
Proof: CD is the tangent to the circle at the point A.
∴ ∠BAD = 90°
EF is the tangent to the circle at the point B.
∴ ∠ABE = 90°
Thus, ∠BAD = ∠ABE         (each equal to 90°).
But these are alternate interior angles.
∴ CD || EF

Example 6:    Prove that the line segment joining the point of contact of two parallel tangents to a circle is a diameter of the circle.
Sol.    Given: CD and EF are two parallel tangents at the points A and B of a circle with centre O.

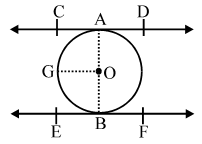
To prove: AOB is a diameter of the circle.
Construction: Join OA and OB.
Draw OG || CD
Proof: OG || CD and AO cuts them.
∴ ∠CAO + ∠GOA = 180°
⇒ ∠GOA = 180°
⇒ ∠GOA = 90°
Similarly, ∠GOB = 90°
∴ ∠GOA + ∠GOB = (90° + 90°) = 180°
⇒ AOB is a straight line
Hence, AOB is a diameter of the circle with centre O.

Example 7:    Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segments joining the pointsof contact to the centre.
Sol.    Given: PA and PB are the tangent drawn from a point P to a circle with centre O. Also, the line segments OA and OB are drawn.

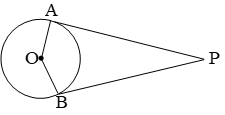
To Prove: ∠APB + ∠AOB = 180°
Proof: We know that the tangent to a circle is perpendicular to the radius through the point of contact.
∴ PA ⊥OA  ⇒  ∠OAP = 90°, and
PB ⊥ OB  ⇒  ∠OBP = 90°.
∴ ∠OAP + ∠OBP = 90°.
Hence, ∠APB + ∠AOB = 180°
[∵ sum of the all the angles of a quadrilateral is 360°]

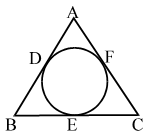
Example 8:     In the given figure, the incircle of ∆ABC touches the sides BC, CA and AB at D, E, F respectively.

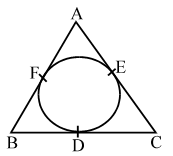
Prove that AF + BD + CE = AE + CD + BF = \(\frac { 1 }{ 2 }\) (perimeter of ∆ABC)
Sol.    We know that the lengths of tangents from an exterior point to a circle are equal.
∴ AF = AE         …. (i)       [tangents from A]
BD = BF            ….. (ii)     [tangents from B]
CE =CD              …. (iii)    [tangents from C]
Adding (i), (ii) and (iii), we get
(AF + BD + CE) = (AE + BF + CD) = k (say)
Perimeter of ∆ABC = (AF + BD +CE) + (AE + BF + CD)
= (k + k) = 2k
∴ k = \(\frac { 1 }{ 2 }\) (perimeter of ∆ABC).
Hence AF + BD + CE = AE + CD + BF = \(\frac { 1 }{ 2 }\) (perimeter of ∆ABC)

Example 9:     A circle touches the side BC of a ∆ABC at P, and touches AB and AC produced at Q and R respectively, as shown in the figure. Show that AQ = \(\frac { 1 }{ 2 }\) (perimeter of ∆ABC)
Sol.    We know that the lengths of tangents drawn from an exterior point to a circle are equal.

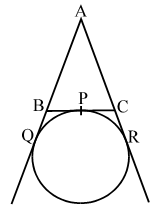
∴ AQ = AR               …. (i)     [tangents from A]
BP = BQ                  …. (ii)     [tangents from B]
CP = CR                  …. (iii)    [tangents from C]
Perimeter of ∆ABC
= AB + BC + AC
= AB + BP + CP + AC
= AB + BQ + CR + AC        [using (ii) and (iii)]
= AQ + AR
= 2AQ       [using (i)].
Hence, AQ = \(\frac { 1 }{ 2 }\) (perimeter of ∆ABC)

Example 10:    Prove that there is one and only one tangent at any point on the circumference of a circle.
Sol. Let P be a point on the circumference of a circle with centre O. If possible, Let PT and PT’ be two tangents at a point P of the circle.
Now, the tangent at any point of a circle is perpendicular to the radius through the point of contact.
∴ OP ⊥PT and similarly, OP⊥PT’
⇒ OPT = 90° and ∠OPT’ = 90°
⇒ OPT = ∠OPT’
This is possible only when PT and PT’ coincide. Hence, there is one and only one tangent at any point on the circumference of a circle.

Example 11:    A quadrilateral ABCD is drawn to circumscribe a circle, as shown in the figure.

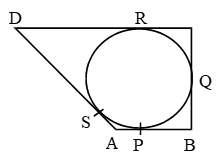
Prove that AB + CD = AD + BC
Sol.    We known that the lengths of tangents drawn from an exterior point to a circle are equal.
∴ AP = AS         ….(i)        [tangents from A]
BP = BQ            ….(ii)      [tangents from B]
CR = CQ            ….(iii)     [tangents from C]
DR = DS            …(iv)      [tangents from D]
∴ AB + CD = (AP + BP) + (CR + DR)
= (AS + BQ) + (CQ + DS)        [using (i), (ii), (iii), (iv)]
= (AS + DS) + (BQ + CQ)
= (AD + BC).
Hence, (AB + CD) = (AD + BC)

Example 12:   Prove that the paralleogram circumscribing a circle, is a rhombus.
Sol.    Given: A parallelogram ABCD circumsribes a circle with centre O.

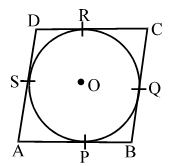
To prove: AB = BC = CD = AD
Proof: we know that the lengths of tangents drawn from an exterior point to a circle are equal.
∴ AP = AS          …. (i)      [tangents from A]
BP = BQ            …. (ii)     [tangents from B]
CR = CQ           …. (iii)     [tangents from C]
DR = DS           …. (iv)     [tangents from D]
∴ AB + CD = AP + BP + CR + DR
= AS + BQ + CQ + DS         [From (i), (ii), (iii), (iv)]
= (AS + DS) + (BQ + CQ)
= AD + BC
Hence, (AB + CD) = (AD + BC)
⇒ 2AB = 2AD
[∵ opposite sides of a parallelogram are equal]
⇒ AB = AD
∴ CD = AB = AD = BC
Hence, ABCD is a rhombus

Example 13:    Prove that the opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Sol.    Given: A quadrilateral ABCD circumscribes a circle with centre O.

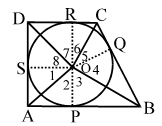
To Prove: ∠AOB+ ∠COD = 180° and ∠BOC + ∠AOD = 180°
Construction: Join OP, OQ, OR and OF
Proof: We know that the tangents drawn from an external point of a circle subtend equal angles at the centre.
∴ ∠1 = ∠ 2, ∠ 3 = ∠ 4, ∠ 5 = ∠ 6 and ∠7= ∠8
And, ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 + ∠7 + ∠8 = 360° [∠s at a point]
⇒ 2 (∠2 + ∠3) + 2 (∠6 + ∠7) = 360° and 2 (∠1+ ∠8) + 2(∠4 + ∠5) = 360°
⇒ ∠2 + ∠3 + ∠6 + ∠7 = 180° and ∠1 + ∠8 + ∠4 + ∠5 = 180°
⇒ ∠AOB + ∠COD = 180° and ∠AOD + ∠BOC = 180°

Example 14:    In the given figure, PQ and RS are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersects PQ at A and RS at B. Prove that ∠AOB = 90º
Sol.    Given: PQ and RS are two parallel tangents to a circle with centre O and AB is a tangent to the circle at a point C, intersecting PQ and RS at A and B respectively.

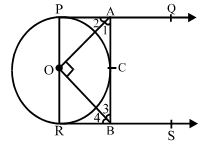
To prove: ∠AOB = 90º
Proof: Since PA and RB are tangents to the circle at P and R respectively and POR is a diameter of the circle, we have
∠OPA = 90º and ∠ORB = 90º
⇒ ∠OPA + ∠ORB = 180º
⇒ PA || RB
We know that the tangents to a circle from an external point are equally inclined to the line segment joining this point to the centre.
∴ ∠2 = ∠1 and ∠4 = ∠3
Now, PA|| RB and AB is a transversal.
∴ ∠PAB + ∠RAB = 180°
⇒ (∠1 + ∠2) + (∠3 + ∠4) = 180°
⇒ 2∠1 + 2∠3 = 180° [ ∵ ∠2 = ∠1 and ∠4 and ∠3 ]
⇒ 2(∠1 + ∠3)= 180°
⇒ ∠1 + ∠3= 90°
From ∆AOB, we have
∠AOB + ∠1 + ∠3 = 180°
[∵ sum of the ∠s of a triangle is 180°]
⇒ ∠AOB + 90° = 180°
⇒ ∠AOB = 90°
Hence, ∠AOB = 90°

Example 15:    ABC is a right triangle, right angled at B. A circle is inscribed in it. The lengths of the two sides containing the right angle are 6 cm and 8 cm. Find the radius of the incircle.
Sol.    Let the radius of the in circle be x cm.

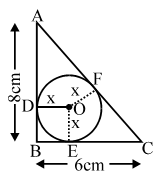
Let the in circle touch the side AB, BC and CA at D, E, F respectively. Let O be the centre of the circle.
Then, OD = OE = OF = x cm.
Also, AB = 8 cm and BC = 6 cm.
Since the tangents to a circle from an external point are equal, we have
AF = AD = (8 – x) cm, and
CF = CE = (6 – x) cm.
∴ AC = AF + CF = (8 – x) cm + (6 – x) cm
= (14 – 2x) cm.
Now , AC² = AB² + BC²
⇒ (14 – 2x)² = 8² + 6² = 100 = (10)²
⇒ 14 – 2x = ±10            ⇒  x = 2 or x = 12
⇒ x = 2      [neglecting x = 12].
Hence, the radius of the in circle is 2 cm.

Example 16:    A point P is 13 cm from the centre of the circle. The length of the tangent drawn from P to the circle is 12cm. Find the radius of the circle.
Sol.    Since tangent to a circle is perpendicular to the radius through the point of contact.

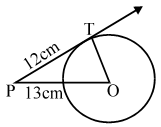
∴ ∠OTP = 90°
In right triangle OTP, we have
OP² = OT² + P²
⇒ 13² = OT² + 12²
⇒ OT² = 13² – 12²
= (13 – 12) (13 + 12) = 25
⇒ OT = 5

Example 17:    Find the length of the tangent drawn from a point whose distance from the centre of a circle is 25 cm. Given that the radius of the circle is 7 cm.
Sol.    Let P be the given point, O be the centre of the circle and PT be the length of tangent from P. Then, OP = 25 cm and OT = 7 cm.

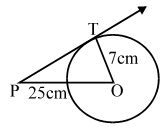
Since tangent to a circle is always perpendicular to the radius through the point of contact.
∴ ∠OTP = 90°
In right triangle OTP, we have
OP² = OT² + PT²
⇒ 25² = 7² + PT²
⇒ PT² = 25² – 7²
= (25 – 7) (25 + 7)
= 576
⇒ PT = 24 cm
Hence, length of tangent from P = 24 cm

Example 18:    In Fig., if AB = AC, prove that BE = EC

Sol.    Since tangents from an exterior point to a circle are equal in length.
∴ AD = AF         [Tangents from A]
BD = BE            [Tangents from B]
CE = CF             [Tangents from C]
Now,
AB = AC
⇒ AB – AD = AC – AD           [Subtracting AD from both sides]
⇒ AB – AD = AC – AF            [Using (i)]
⇒ BD = CF    ⇒   BE = CF            [Using (ii)]
⇒ BE = CE [Using (iii)]

Example 19:    In fig. XP and XQ are tangents from X to the circle with centre O. R is a point on the circle. Prove that, XA + AR = XB + BR.
Sol.    Since lengths of tangents from an exterior point to a circle are equal.

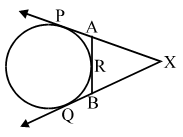
∴  XP = XQ      …. (i)    [From X]
AP = AR           …. (ii)   [From A]
BQ = BR           …. (iii)  [From B]
Now, XP = XQ
⇒ XA + AP = XB + BQ
⇒ XA + AR = XB + BR      [Using equations (i) and (ii)]

Example 20:    PA and PB are tangents from P to the circle with centre O. At point M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.
Sol.    We know that the tangents drawn from an external point to a circle are equal in length.

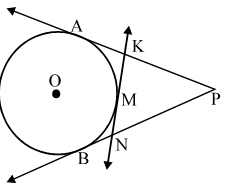
∴ PA = PB          …. (i)         [From P]
KA = KM          …. (ii)         [From K]
and, NB = NM       …. (iii)     [From N]
Adding equations (ii) and (iii), we get
KA + NB = KM + NM
⇒ AK + BN = KM + MN   ⇒   AK + BN = KN

Example 21:    ABCD is a quadrilateral such that ∠D = 90°. A circle (O, r) touches the sides AB, BC, CD and DA at P,Q,R and S respectively. If BC = 38 cm, CD = 25 cm and BP = 27 cm, find r.
Sol.    Since tangent to a circle is perpendicular to the radius through the point.
∴ ∠ORD = ∠OSD = 90°

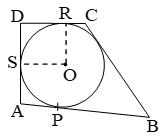
It is given that ∠D = 90° Also, OR = OS. Therefore, ORDS is a square.
Since tangents from an exterior point to a circle are equal in length.
∴ BP = BQ
CQ = CR and DR = DS
Now, BP = BQ
⇒ BQ = 27            [∵BP = 27 cm (Given)]
⇒ BC – CQ = 27
⇒ 38 – CQ = 27   [∵BC = 38 cm ]
⇒ CQ = 11cm
⇒ CR = 11cm          [∵CR = CQ ]
⇒ CD – DR = 11
⇒ 25 – DR = 11      [∵CD = 25cm ]
⇒ DR = 14 cm
But, ORDS is a square.
Therefore, OR = DR = 14 cm
Hence, r = 14 cm

Example 22:    Prove that the tangents at the extremities of any chord make equal angles with the chord.
Sol.    Let AB be a chord of a circle with centre O, and let AP and BP be the tangents at A and B respectively.

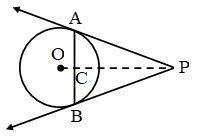
Suppose the tangents meet at P. Join OP. Suppose OP meets AB at C. We have to prove that ∠PAC = ∠PBC In triangles PCA and PCB, we have
PA = PB
[∵ Tangents from an external point are equal]
∠APC = ∠BPC
[∵PA and PB are equally inclined to OP]
and, PC = PC [Common]
So, by SAS – criterion of congruence, we have
∆PAC ≅ ∆PBC
⇒  ∠PAC = ∠PBC

Example 23:    In fig., O is the centre of the circle, PA and PB are tangent segments. Show that the quadrilateral AOBP is cyclic.
Sol.    Since tangent at a point to a circle is perpendicular to the radius through the point.

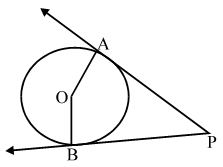
∴ OA ⊥ AP and OB ⊥ BP
⇒ ∠OAP = 90° and ∠OBP = 90°
⇒ ∠OAP +∠OBP = 90° + 90°       ….(i)
In quadrilateral OAPB, we have
∠OAP + ∠APB + ∠AOB + ∠OBP = 360°
⇒ (∠APB + ∠AOB) + (∠OAP +∠OBP) = 360°
⇒ ∠APB + ∠AOB + 180° = 360°
∠APB + ∠AOB = 180°                 ….(ii)
From equations (i) and (ii),we can say that the
quadrilateral AOBP is cyclic.

Example 24:    In fig., circles C(O, r) and C(O’, r/2) touch internally at a point A and AB is a chord of the circle C (O, r) intersecting C(O’,  r/2) at C, Prove that AC = CB.
Sol.    Join OA, OC and OB. Clearly, ∠OCA is the angle in a semi-circle.

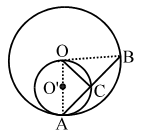
∴ ∠OCA = 90°
In right triangles OCA and OCB, we have
OA = OB = r
∠OCA = ∠OCB = 90°
and OC = OC
So, by RHS criterion of congruence, we get
∆OCA ≅ ∆OCB
⇒ AC = CB

Example 25:    In two concentric circles, prove that all chords of the outer circle which touch the inner circle are of equal length.
Sol.    Let AB and CD be two chords of the circle which touch the inner circle at M and N respectively.

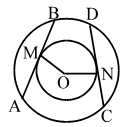
Then, we have to prove that
AB = CD
Since AB and CD are tangents to the smaller circle.
∴ OM = ON = Radius of the smaller circle
Thus, AB and CD are two chords of the larger circle such that they are equidistant from the centre. Hence, AB = CD.