Linear - Quadratic Systems Archives

Solving Linear Quadratic Systems Algebraically

A linear quadratic system is a system containing one linear equation and one quadratic equation
which may be one straight line and one parabola,
or one straight line and one circle.
Algebraic Solutions
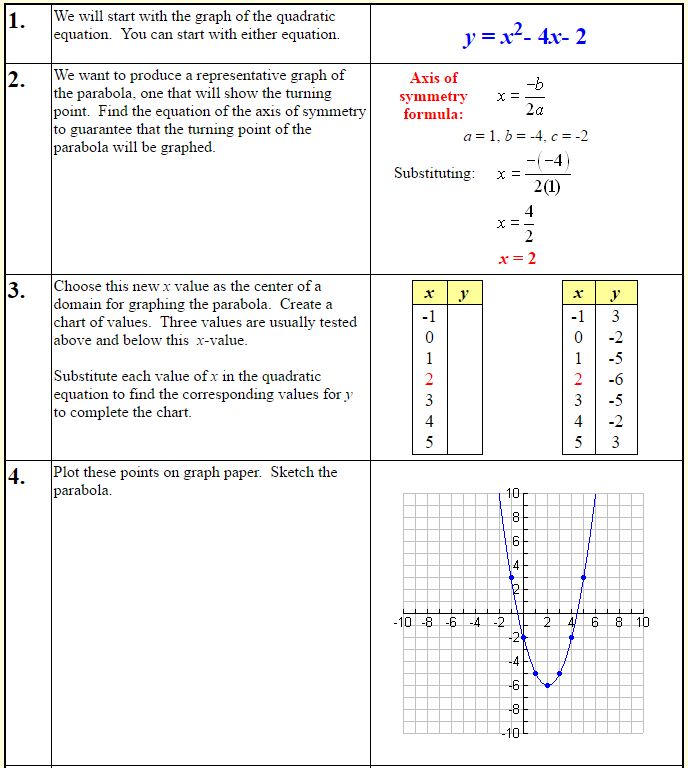
straight line: y = mx + b
parabola: y = ax² + bx + c; a ≠ 0
circle: (x – h)² + (y – k)² = r² ; center (h.k), radius r

Let’s look at how to solve a linear quadratic system of equations algebraically.

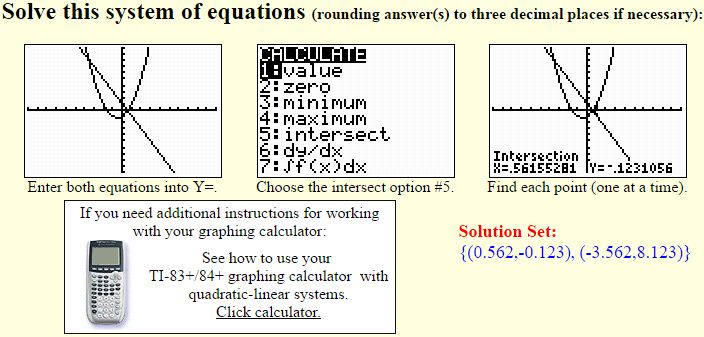
Example 1:
Solve this linear-quadratic system of equations algebraically and check your solution:
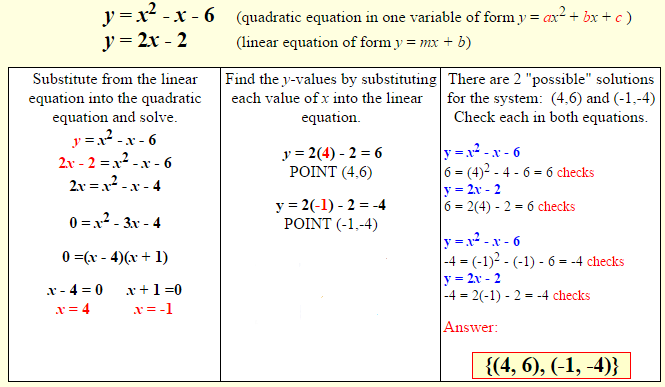
y = x²– 6x + 3 (parabola)
y = -2x + 3 (straight line)
Solution:

Solve for one of the variables in the linear equation.
Note: In this example, this process is already done for us, since y = -2x + 3.
y = -2x + 3
Substitute this value into the quadratic equation, and solve the resulting equation.
• Substitute -2x + 3 for y in the quadratic equation.
• Subtract 3 from both sides; then add 2x to both sides.
• Factor.
• Set each factor equal to zero and solve.
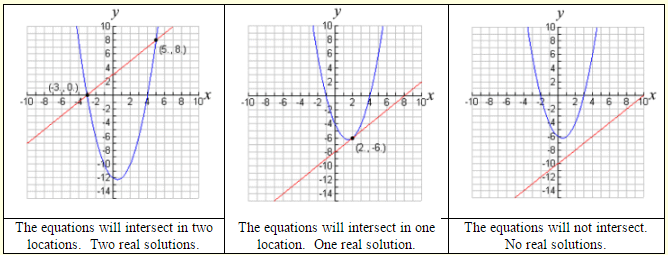
You now have TWO values for x. This tells you that there may be two possible solutions.
TWO SOLUTIONS.
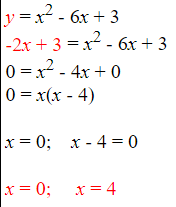
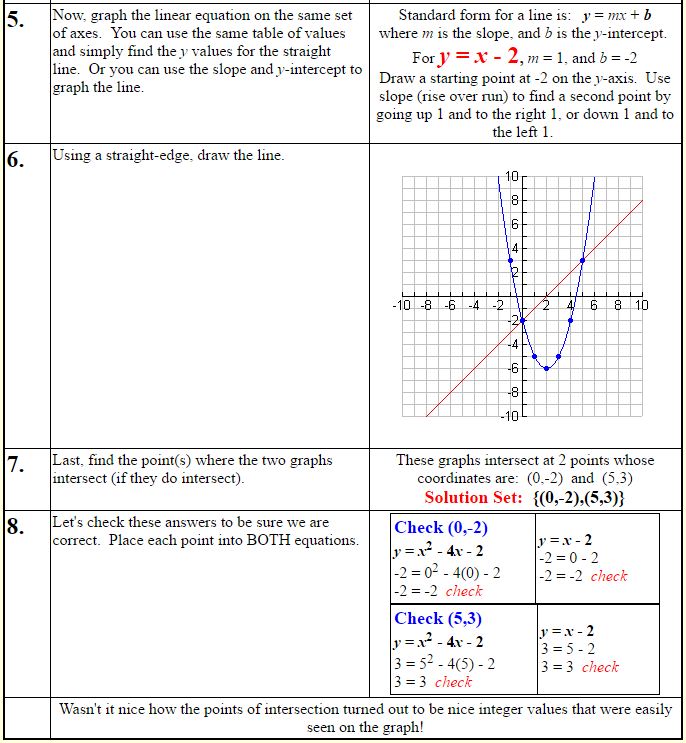
Find the corresponding values for y. Substitute each value into the linear equation in place of x.
Yes, you could substitute in the quadratic equation, but substituting into the linear equation will be easier.
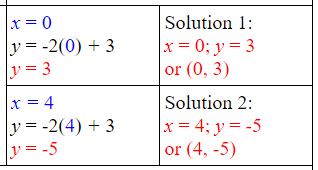
Check: Be sure to check BOTH solutions in both equations.
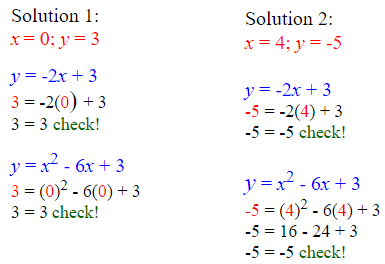
State the final solutions. The solutions may be stated as the set {(0, 3), (4, -5)}

Example 2:
Solve this linear-quadratic system of equations algebraically and check your solution:
y = x²– 6x + 3 (parabola)
2x – y = 13 (straight line)
Solution:

Solve for one of the variables in the linear equation.
2x – y = 13
y = 2x – 13
Substitute this value into the quadratic equation, and solve the resulting equation.
• Substitute 2x – 13 for y in the quadratic equation.
• Add 13 to both sides; then subtract 2x from both sides.
• Factor.
• Set each factor equal to zero and solve.
You now have ONE value for x. This tells you that there may be only one solution.
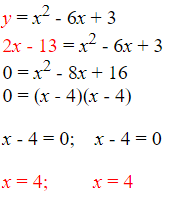
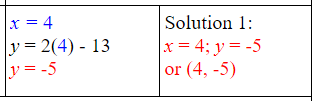
Find the corresponding value for y. Substitute the value into the linear equation in place of x.
Yes, you could substitute in the quadratic equation, but substituting into the linear equation will be easier.
Check: Be sure to check the solution in both equations.
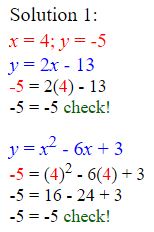
State the final solution. The solution may be stated as (4, -5) or {(4, -5)}

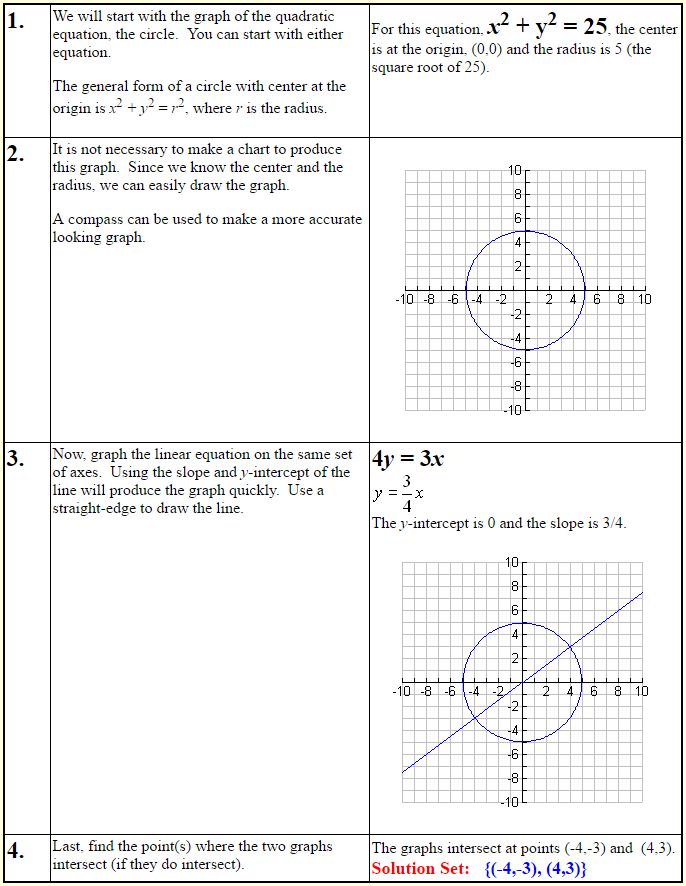
Example 3:
Solve this linear-quadratic system of equations algebraically and check your solution:
x²+ y² = 9 (circle)
x – y = 3 (straight line)
Solution:

Solve for one of the variables in the linear equation.
y = x – 3
Substitute this value into the quadratic equation, and solve the resulting equation.
• Substitute x – 3 for y in the quadratic equation.
• Expand (x – 3)²
• Combine terms.
• Factor.
• Set each factor equal to zero and solve.
You now have TWO values for x. This tells you that there may be two possible solutions.
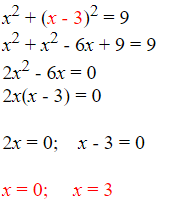
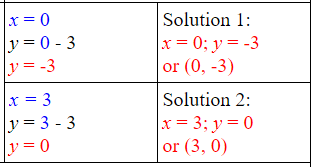
Find the corresponding values for y. Substitute each value into the linear equation in place of x.
Yes, you could substitute in the quadratic equation, but substituting into the linear equation will be easier.
Check: Be sure to check BOTH solutions in both equations.
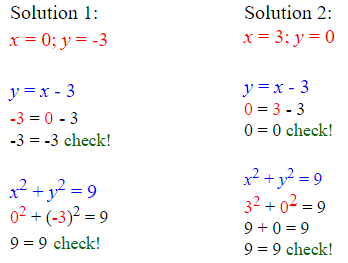
State the final solutions. The solutions may be stated as the set {(0, -3), (3, 0)}

Linear – Quadratic Systems

A quadratic equation is defined as an equation in which one or more of the terms is squared but raised to no higher power. The general form is ax² + bx + c = 0, where a, b and c are constants.

In Algebra and Geometry, we learned how to solve linear – quadratic systems algebraically and graphically. With our new found knowledge of quadratics, we are now ready to attack problems that cannot be solved by factoring, and problems with no real solutions.

Linear – quadratic system: (where the quadratic is in one variable – only one variable is squared)
y = x – 2 (linear)
y = x2 – 4x – 2 (quadratic – a parabola)
This familiar linear- quadratic system, where only one variable is squared in the quadratic, will be the graph of a parabola and a straight line. When a parabola and a straight line are graphed on the same set of axes, three situations are possible.
Linear - Quadratic Systems 1

Keep these images in mind as we proceed to solve these linear-quadratic systems algebraically.

Example 1:
When we studied these systems in Algebra, we encountered situations that could be solved by factoring, such as this first example.
Solve this system of equations algebraically:
Linear - Quadratic Systems 2

Example 2:
Solve this problem manually (without graphing calculator):
y = x² – 4x – 2
y = x – 2
Solution:
Linear - Quadratic Systems 3

The problem we just solved worked out “nicely” since the intersection points were easily seen as integer values on the graph paper. Of course, this does not happen with all graphs. How could we tell, by looking at the graph, if a point of intersection was (2.3, 1.5), for example?
Answer: We could not tell “by looking”. We would have to solve the system algebraically to find such an intersection point, or we would have to use our graphing calculator with the intersect option.

Example 3:
Solve this problem using a graphing calculator:
y = x² + x – 1
y = -2x + 1
Solution:
Linear - Quadratic Systems 5

Example 4:
Linear – quadratic system: (where the quadratic is in two variables – both variables are squared)
4y = 3x (linear)
x² + y² = 25 (quadratic – a circle)
Solution:
Solve this problem manually (without graphing calculator):
Linear - Quadratic Systems 6