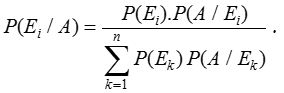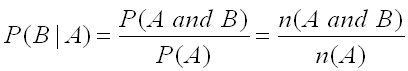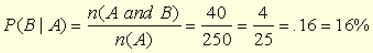Conditional Probability Archives

What are Addition and Multiplication Theorems on Probability?

Addition and Multiplication Theorem of Probability

State and prove addition and multiplication theorem of probability with examples

Equation Of Addition and Multiplication Theorem

Notations :

P(A + B) or P(A∪B) = Probability of happening of A or B
= Probability of happening of the events A or B or both
= Probability of occurrence of at least one event A or B
P(AB) or P(A∩B) = Probability of happening of events A and B together.

(1) When events are not mutually exclusive:
If A and B are two events which are not mutually exclusive, then
P(A∪B) = P(A) + P(B) – P(A∩B)
or P(A + B) = P(A) + P(B) – P(AB)
For any three events A, B, C
P(A∪B∪C) = P(A) + P(B) + P(C) – P(A∩B) – P(B∩C) – P(C∩A) + P(A∩B∩C)
or P(A + B + C) = P(A) + P(B) + P(C) – P(AB) – P(BC) – P(CA) + P(ABC)

(2) When events are mutually exclusive:
If A and B are mutually exclusive events, then
n(A∩B) = 0 ⇒ P(A∩B) = 0
∴ P(A∪B) = P(A) + P(B).
For any three events A, B, C which are mutually exclusive,
P(A∩B) = P(B∩C) = P(C∩A) = P(A∩B∩C) = 0
∴ P(A∪B∪C) = P(A) + P(B) + P(C).
The probability of happening of any one of several mutually exclusive events is equal to the sum of their probabilities, i.e. if A₁, A₂ ……… A_n are mutually exclusive events, then
P(A₁ + A₂ + … + A_n) = P(A₁) + P(A₂) + …… + P(A_n)
i.e. P(Σ A_i) = Σ P(A_i).

(3) When events are independent :
If A and B are independent events, then P(A∩B) = P(A).P(B)
∴ P(A∪B) = P(A) + P(B) – P(A).P(B)

(4) Some other theorems

Let A and B be two events associated with a random experiment, then
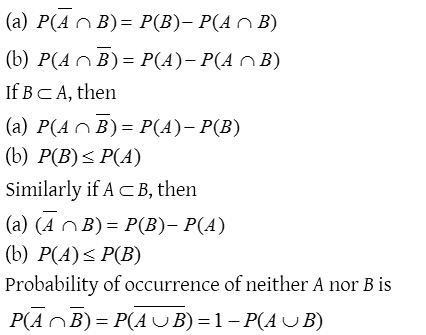
Generalization of the addition theorem :
If A₁, A₂ ……… A_n are n events associated with a random experiment, then
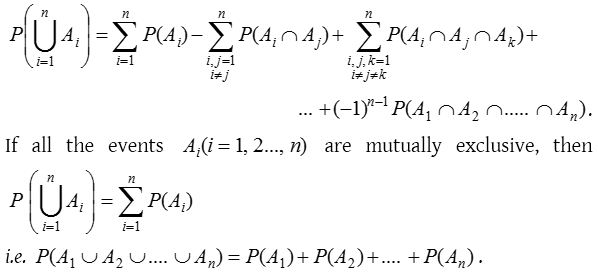
Booley’s inequality : If A₁, A₂ ……… A_n are n events associated with a random experiment, then

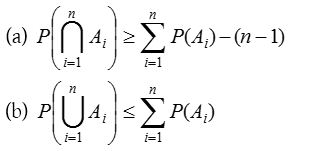
These results can be easily established by using the Principle of mathematical induction.

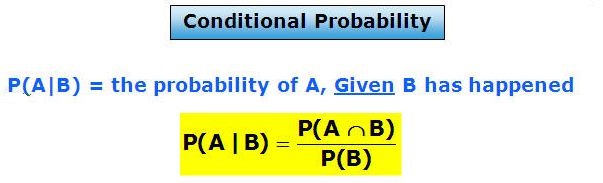
Conditional probability

Let A and B be two events associated with a random experiment. Then, the probability of occurrence of A under the condition that B has already occurred and P(B) ≠ 0, is called the conditional probability and it is denoted by P(A/B).
Thus, P(A/B) = Probability of occurrence of A, given that B has already happened.
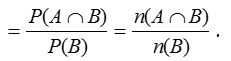
What are Addition and Multiplication Theorems on Probability 4
Similarly, P(B/A) = Probability of occurrence of B, given that A has already happened.

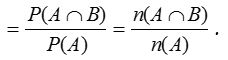
Sometimes, P(A/B) is also used to denote the probability of occurrence of A when B occurs. Similarly, P(B/A) is used to denote the probability of occurrence of B when A occurs.

Multiplication Theorem Of Probability

If A and B are two events associated with a random experiment, then P(A∩B) = P(A).P(B/A), if P(A) ≠ 0 or P(A∩B) = P(B).P(A/B), if P(B) ≠ 0.
Extension of multiplication theorem:
If A₁, A₂ ……… A_n are n events related to a random experiment, then
P(A₁∩A₂∩A₃∩ … ∩A_n) = P(A₁) P(A₂/A₁) P(A₃/A₁∩A₂)……P(A_n/A₁∩A₂∩…∩A_n−1),
where P(A_i/A₁∩A₂∩…∩A_i−1), represents the conditional probability of the event , given that the events A₁, A₂ ……… A_i₋₁ have already happened.
Multiplication theorems for independent events:
If A and B are independent events associated with a random experiment, then P(A∩B) = P(A).P(B) i.e., the probability of simultaneous occurrence of two independent events is equal to the product of their probabilities. By multiplication theorem, we have P(A∩B) = P(A).P(B/A). Since A and B are independent events, therefore P(B/A) = P(B). Hence, P(A∩B) = P(A).P(B).
Extension of multiplication theorem for independent events:
If A₁, A₂ ……… A_n are independent events associated with a random experiment, then
P(A₁∩A₂∩A₃∩ … ∩A_n) = P(A₁) P(A₂)..… P(A_n).
By multiplication theorem, we have
P(A₁∩A₂∩A₃∩ … ∩A_n) = P(A₁) P(A₂/A₁) P(A₃/A₁∩A₂)……P(A_n/A₁∩A₂∩…∩A_n−1)
Since A₁, A₂ ………A_n-1, A_n are independent events, therefore
P(A₂/A₁) = P(A₂), P(A₃/A₁∩A₂) = P(A₃),……, P(A_n/A₁∩A₂∩…∩A_n−1) = P(A_n)
Hence, P(A₁∩A₂∩A₃∩ … ∩A_n) = P(A₁) P(A₂)..… P(A_n).

Probability of at least one of the n independent events:
If p₁, p₂ ……… p_n be the probabilities of happening of n independent events A₁, A₂ ……… A_n respectively, then
What are Addition and Multiplication Theorems on Probability 6

Total probability and Baye’s rule

(1) The law of total probability:
Let S be the sample space and let E₁, E₂ ……… E_n be n mutually exclusive and exhaustive events associated with a random experiment. If A is any event which occurs with E₁ or E₂ or …or E_n, then
P(A) = P(E₁) P(A/E₁) + P(E₂) P(A/E₂) + ….. P(E_n)P(A/E_n).

(2) Baye’s rule:
Let S be a sample space and E₁, E₂ ……… E_n be n mutually exclusive events such that
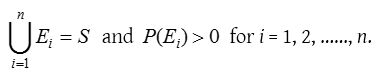
What are Addition and Multiplication Theorems on Probability 7
We can think of E_i’s as the causes that lead to the outcome of an experiment. The probabilities P(E_i), i = 1, 2, ….., n are called prior probabilities. Suppose the experiment results in an outcome of event A, where P(A) > 0. We have to find the probability that the observed event A was due to cause E_i, that is, we seek the conditional probability P(E_i/A). These probabilities are called posterior probabilities, given by Baye’s rule as
What are Addition and Multiplication Theorems on Probability 8

The conditional probability of an event B, in relation to event A, is the probability that event B will occur given the knowledge that an event A has already occurred.

In plain English …
You toss two pennies. The first penny shows HEADS and the other penny rolls under the table and you cannot see it. Now, what is the probability that they are both HEADS? Since you already know that one is HEADS, the probability of getting HEADS on the second penny is 1 out of 2.
The probability changes if you have partial information.
This “affected” probability is called conditional probability.

Notation for conditional probability: \(P(\frac { B }{ A } )\)
read … the probability of B given A.

To establish our formulas for conditional probability, we will need to revisit our previous discussion of independent and dependent events.

If events A and B are independent (where event A has no effect on the probability of event B), then the conditional probability of event B given event A is simply the probability of event B.

Example: Two colored dice (one blue, one yellow) are rolled.
a. What is the probability of rolling “box cars” (two sixes) is \(\frac { 1 }{ 6 } \times \frac { 1 }{ 6 } =\frac { 1 }{ 36 }\)?
b. What is the probability of rolling “box cars” knowing the first toss is a six?
Answer:
a. The probability of getting “box cars” (two sixes) is \(\frac { 1 }{ 6 } \).
b. If, however, we roll the dice and and see that the blue die shows a six (and the yellow die is out of sight), the probability of the yellow die being six is .
The probability of rolling “box cars”, knowing that the first roll is a six, is \(\frac { 1 }{ 6 } \).

The probability changes when you have partial information about the situation.
If events A and B are dependent (where event A has effect on the probability of event B), then we saw that the probability that both events occur is defined by:
\(P(A\quad and\quad B)=P(A)\times P(\frac { B }{ A } )\)

Dividing both sides of this equation by P(A) gives us our formula for conditional probability of event B given event A, where event A affects the probability of event B:
Conditional Probability 2

Assuming P(A), n(A) are not zero.

Example 1: A bag contains 12 red M&Ms, 12 blue M&Ms, and 12 green M&Ms. What is the probability of drawing two M&Ms of the same color in a row?
Answer:
Intuitive: There are a total of 36 M&Ms in the bag. You draw a blue M&M and eat it. There are now 11 blue M&Ms remaining in the bag. There are 35 total M&Ms now remaining. You will now need to draw another blue M&M. The conditional probability will be:
P(Draw blue M&M | First M&M was blue) = \(\frac { 11 }{ 35 }\).
Using the formula:
P(A and B) = \(\frac { 12 }{ 36 } \times \frac { 11 }{ 35 } \)
P(A) = \(\frac { 12 }{ 36 }\)
P(Draw same as first color M&M | First M&M color)
Conditional Probability 3

Example 2: In a school of 1200 students, 250 are seniors, 150 students take math, and 40 students are seniors and are also taking math. What is the probability that a randomly chosen student who is a senior, is taking math?
Answer: These questions can be confusing. It sounds, at first read, that they are asking for the probability of choosing a student who is a senior and who is taking math. Not quite right!
It helps to re-word the question into:
Find the probability that the student is taking math, given that the student is a senior.
B = the student is taking math
n(A) = the student is a senior = 250.
n(A and B) = the student is a senior and is taking math = 40.
Conditional Probability 4