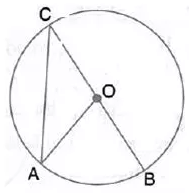
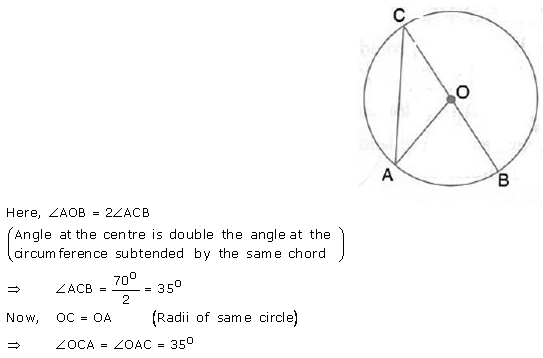
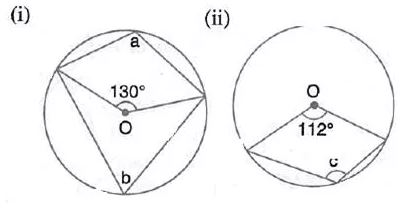
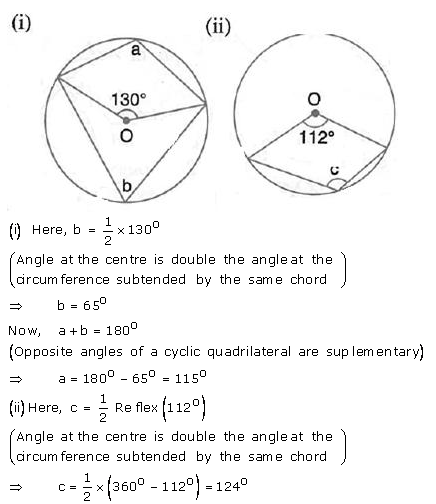
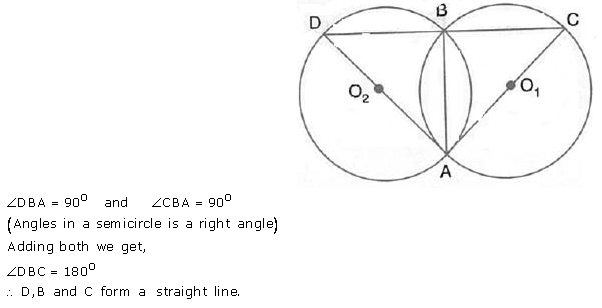
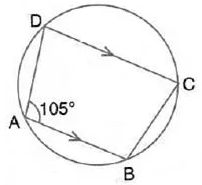
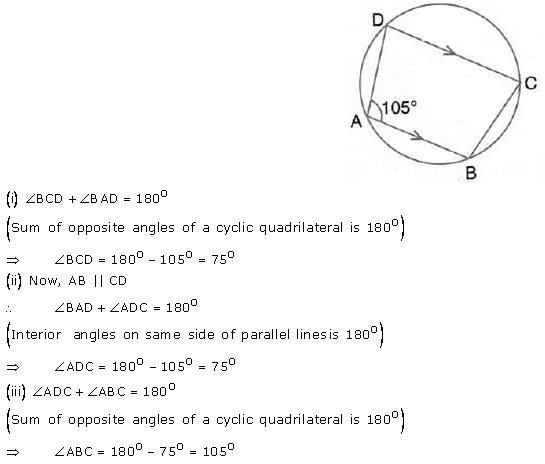
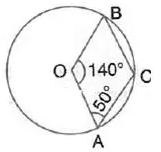
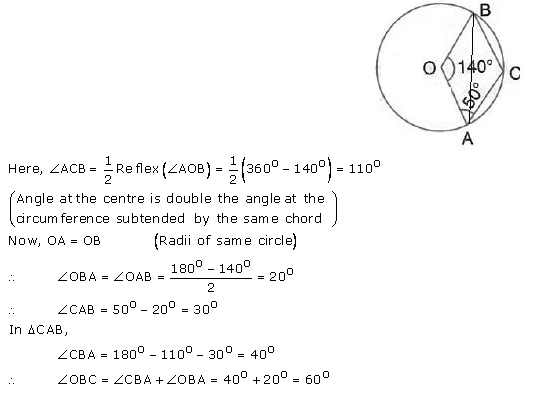
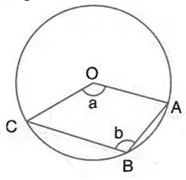
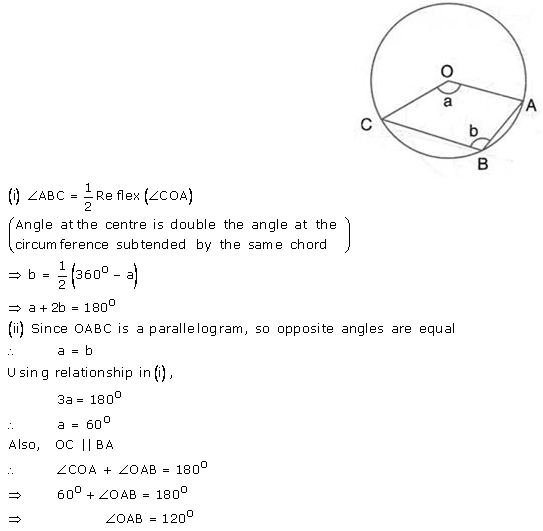
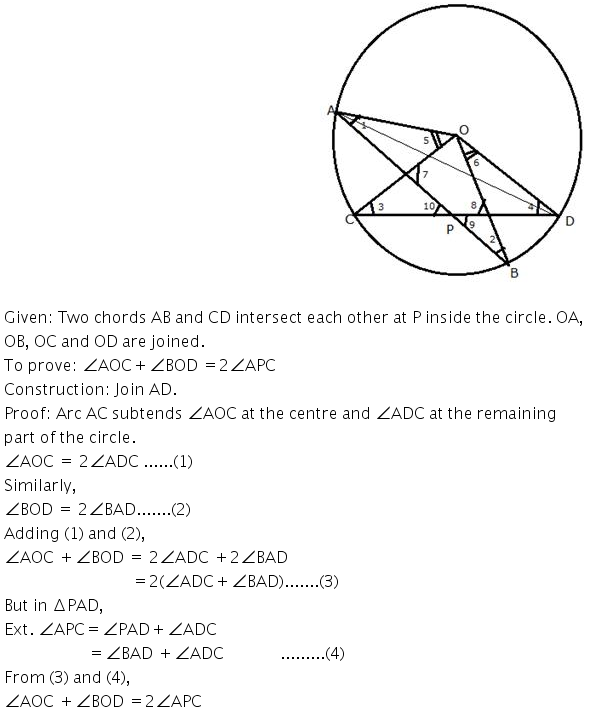
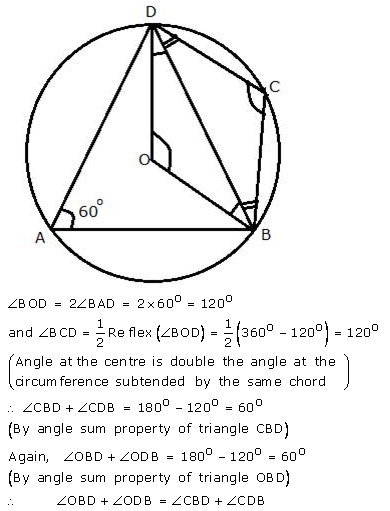
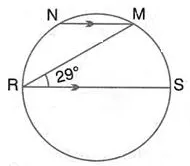
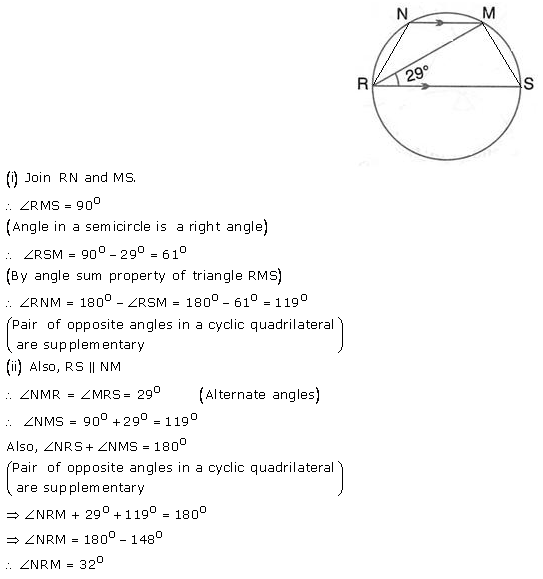
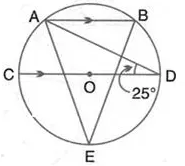
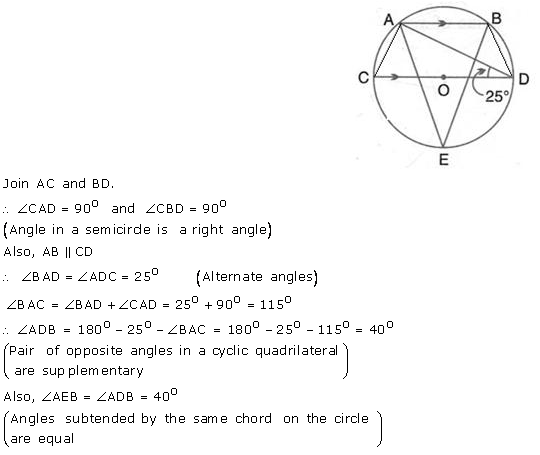
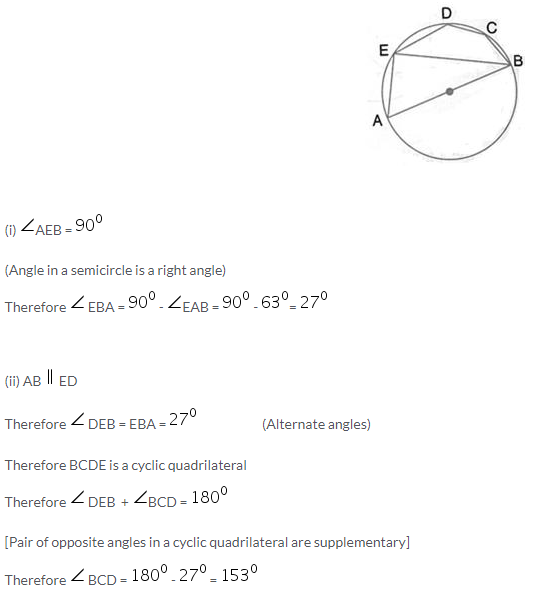
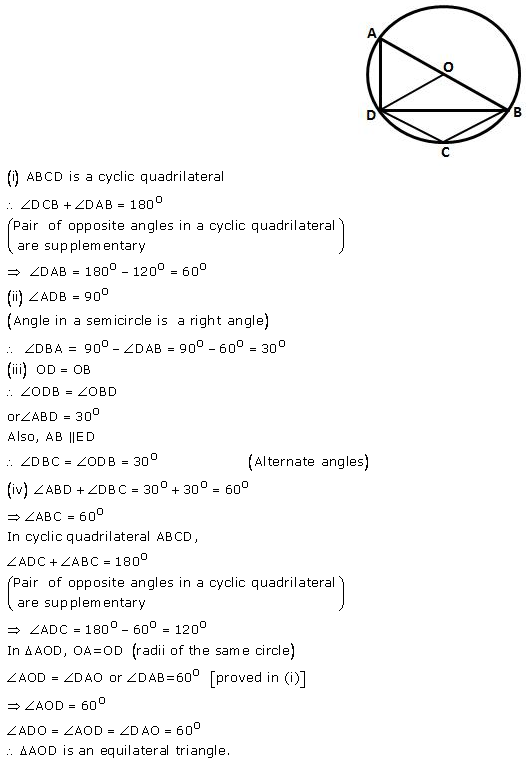
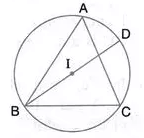
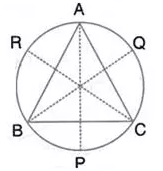
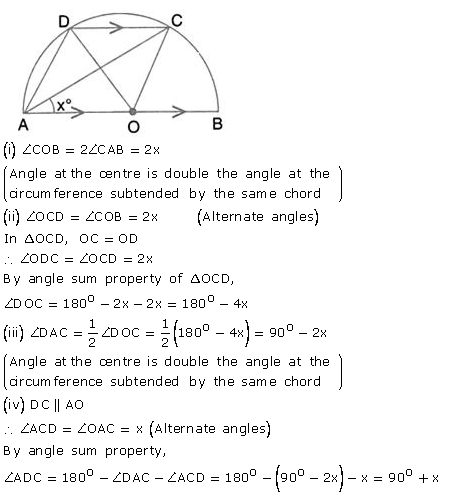
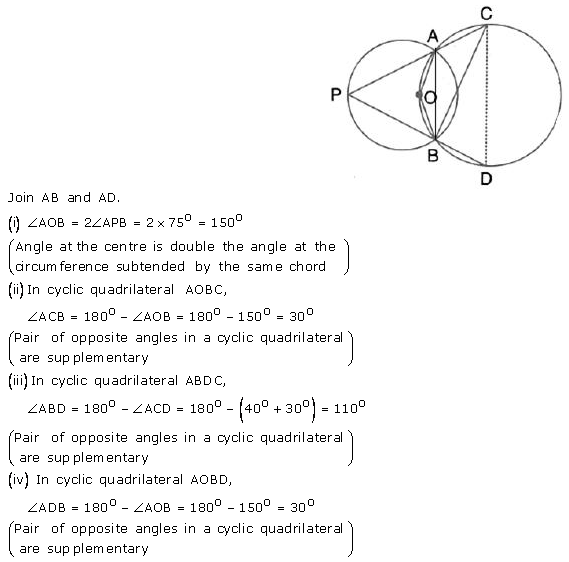
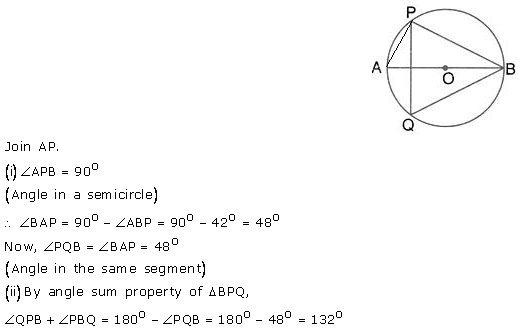
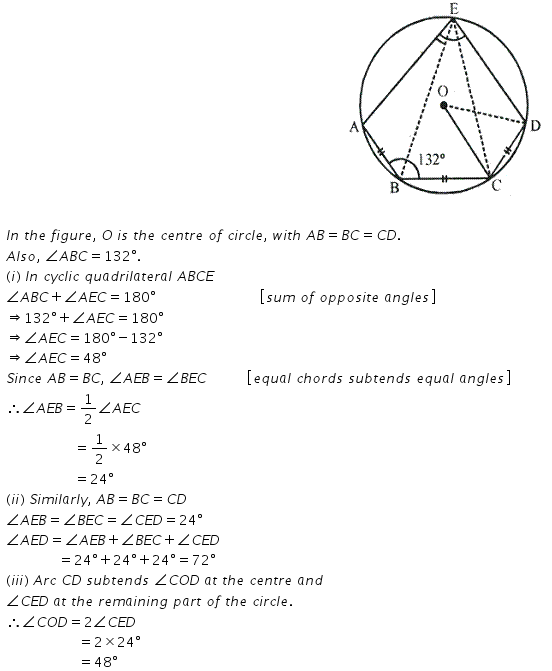
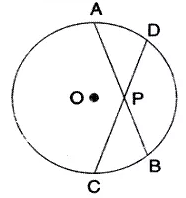
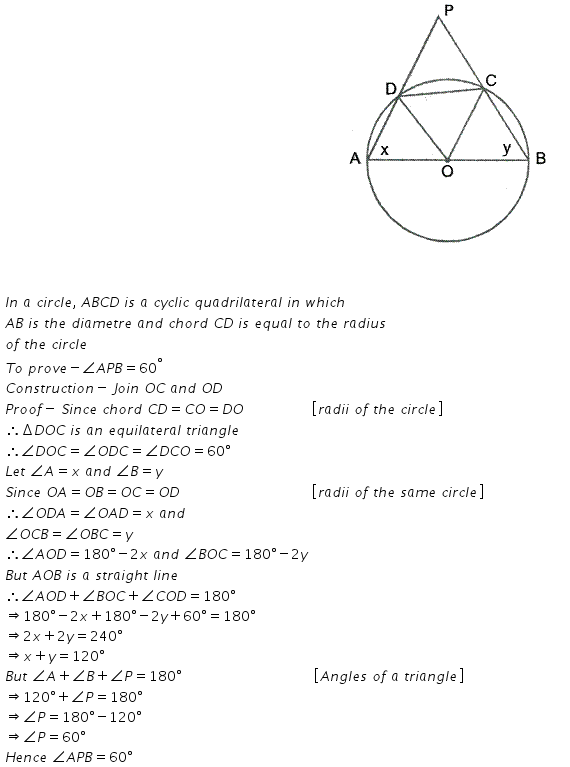
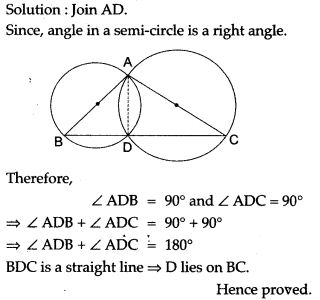
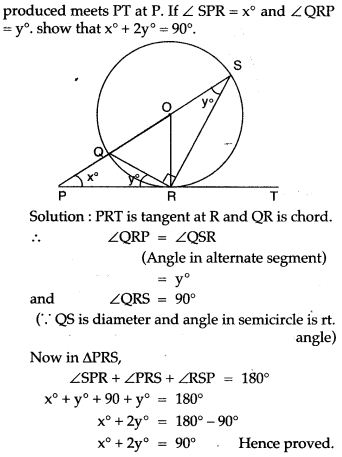
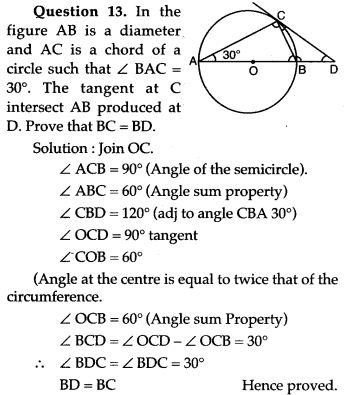
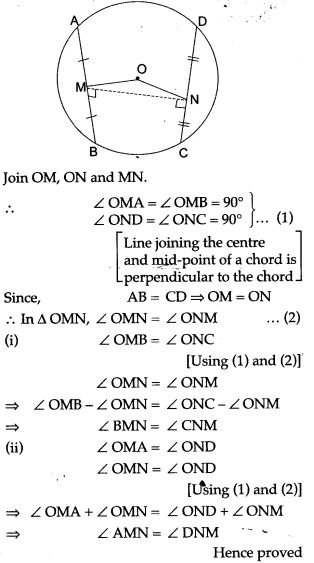
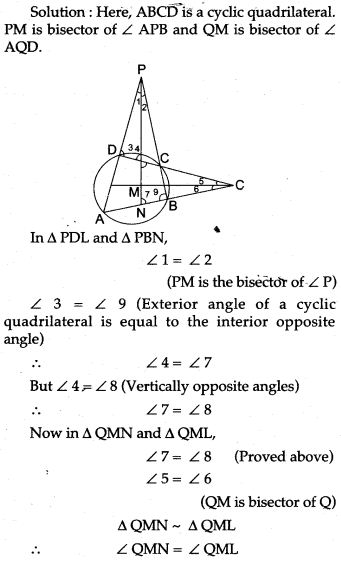
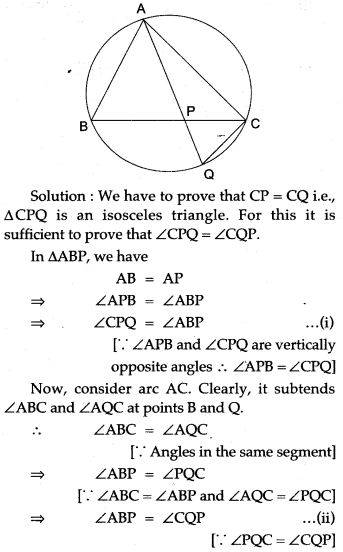
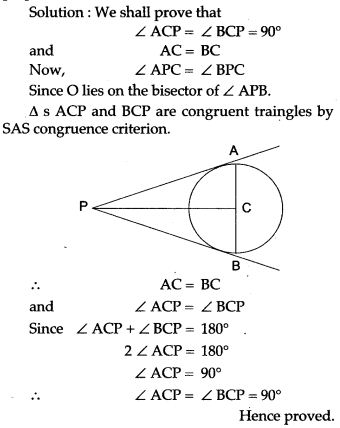
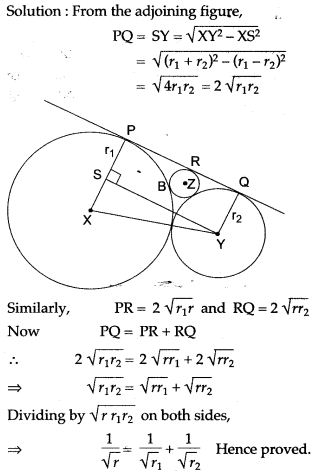
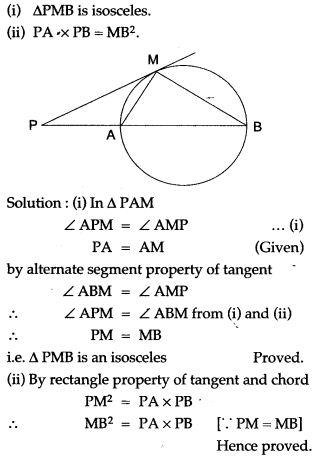
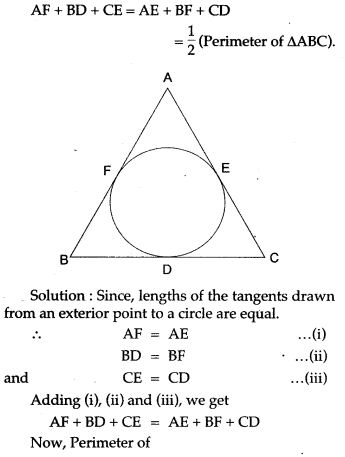
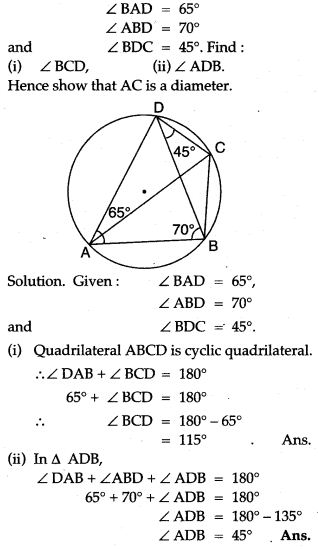
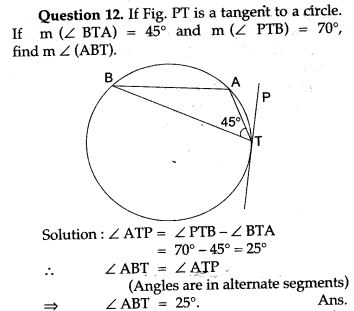
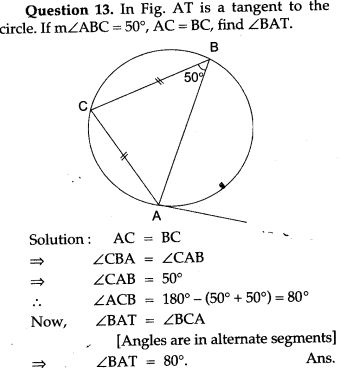
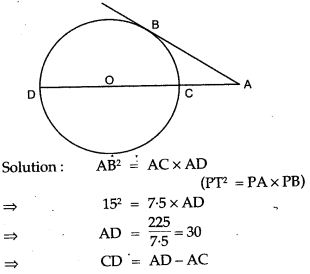
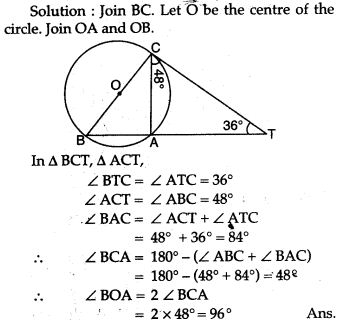
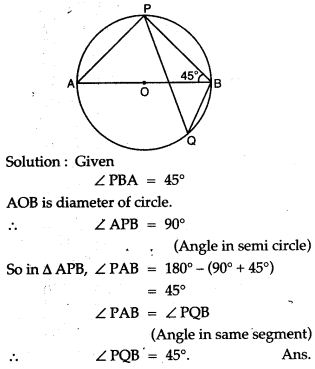
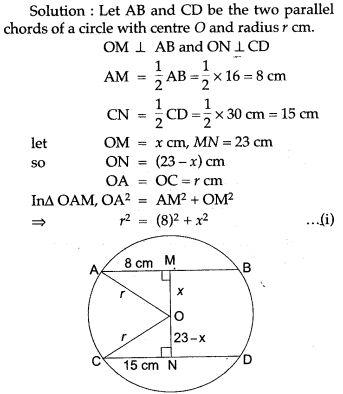
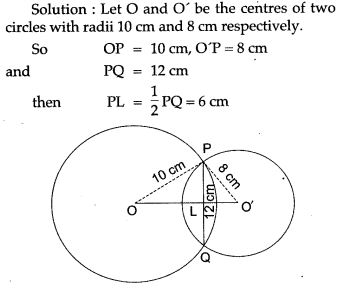
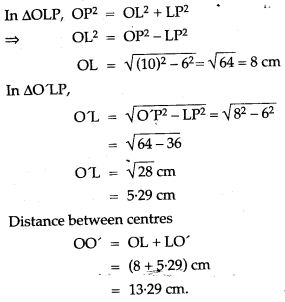
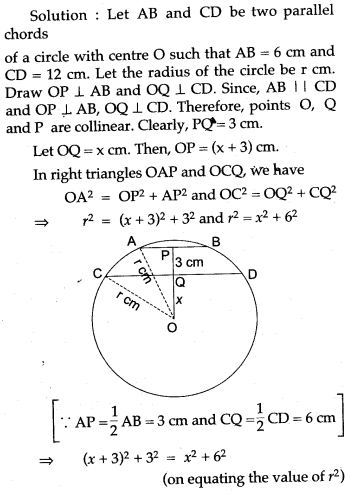
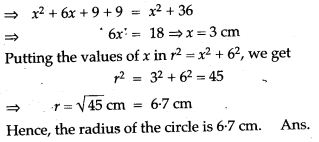
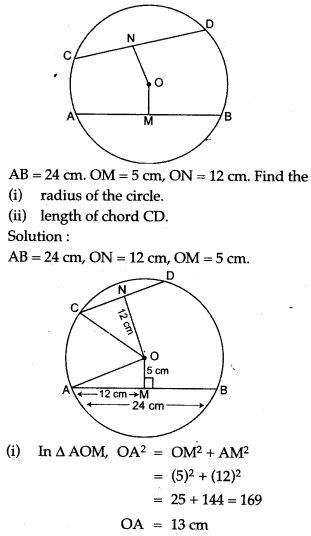
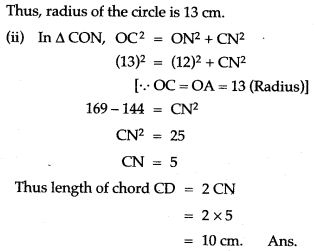
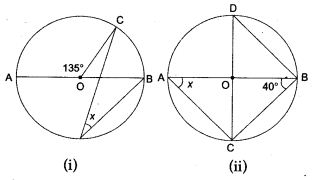
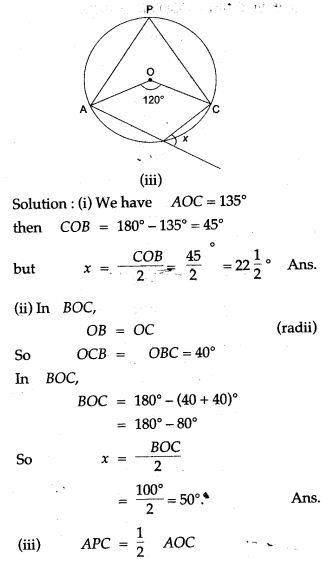
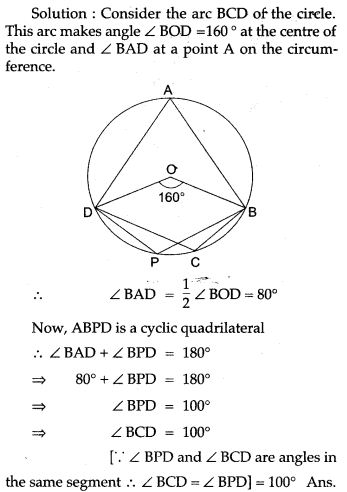
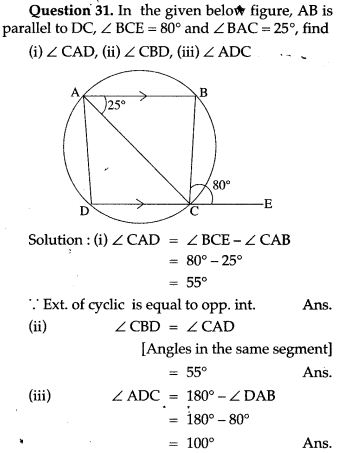
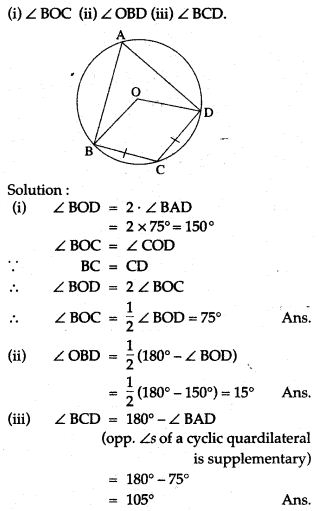
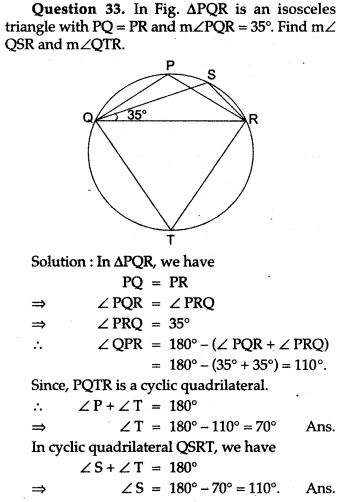
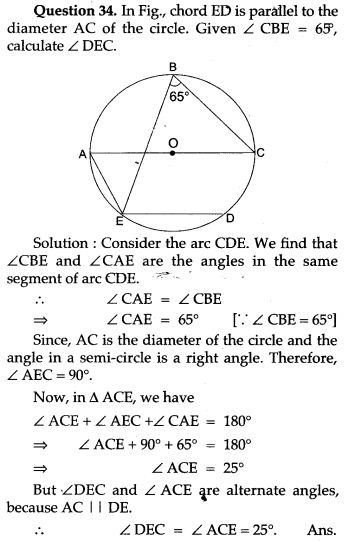
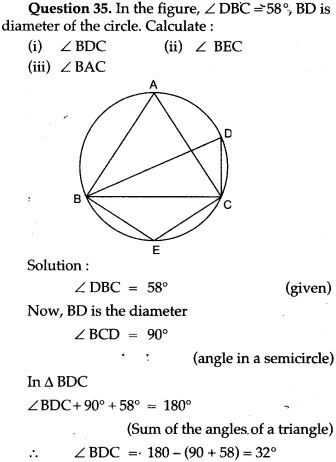
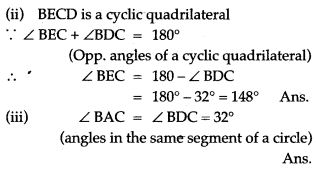
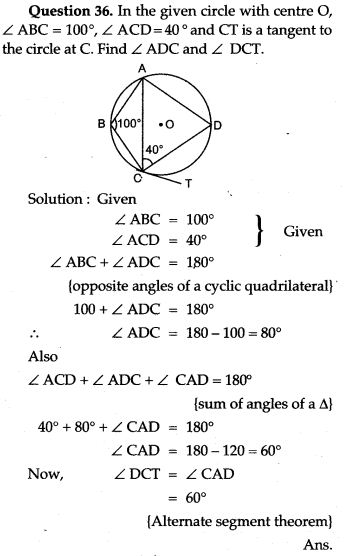
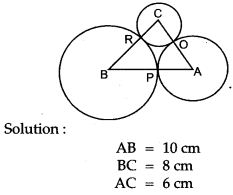
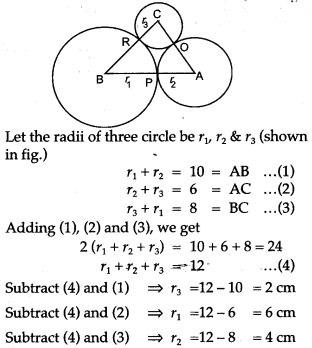
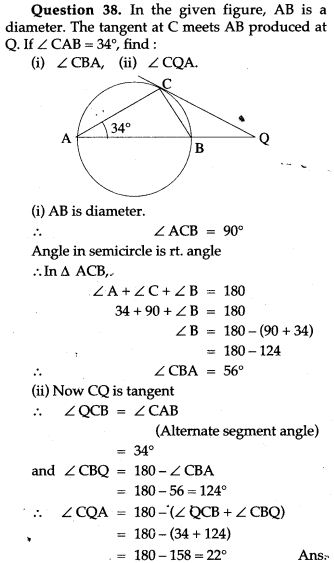
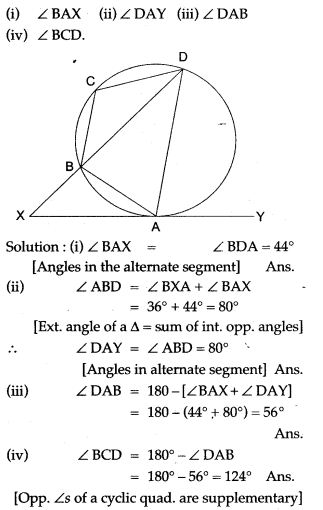
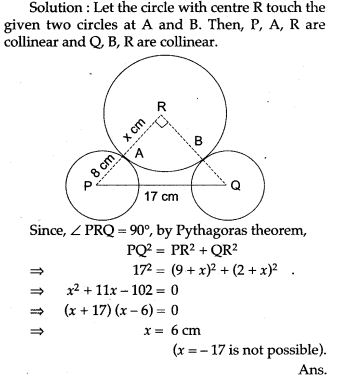
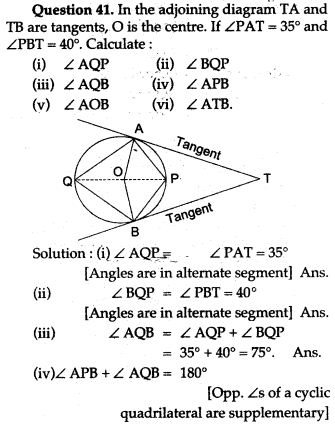
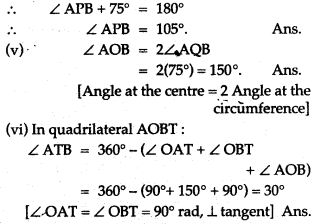
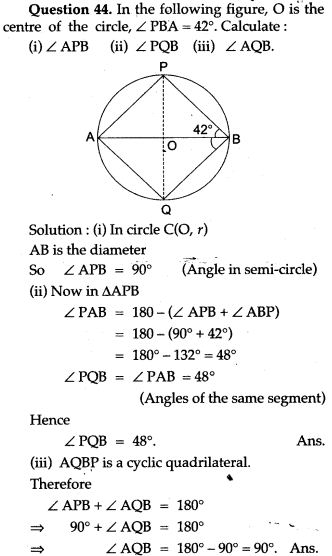
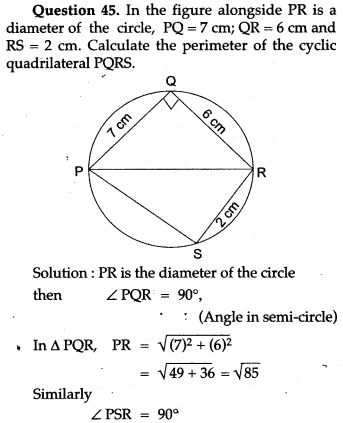
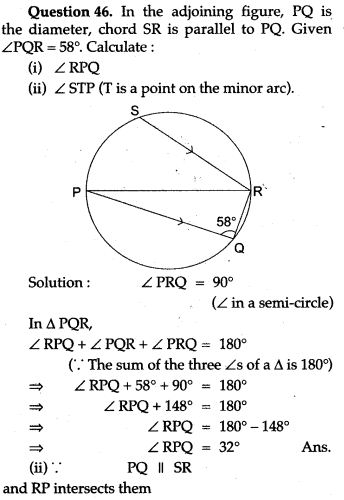
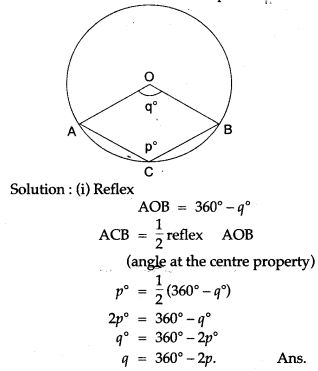
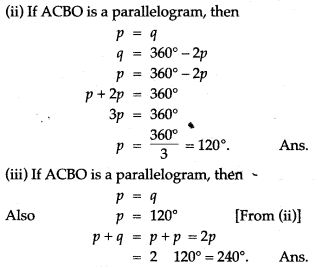
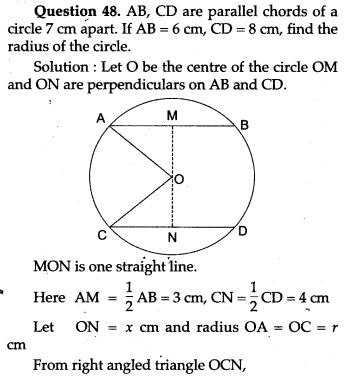
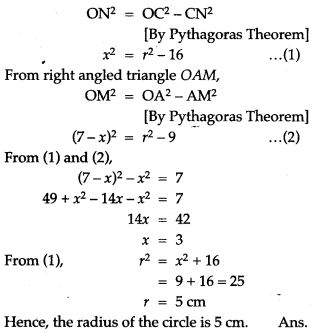
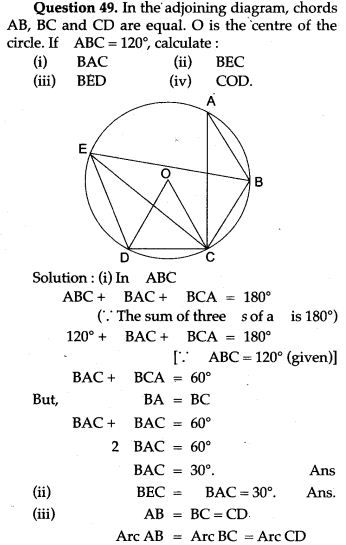
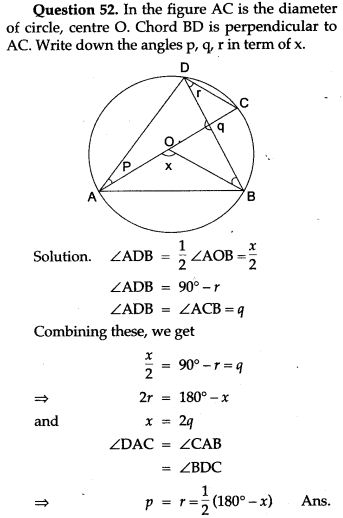
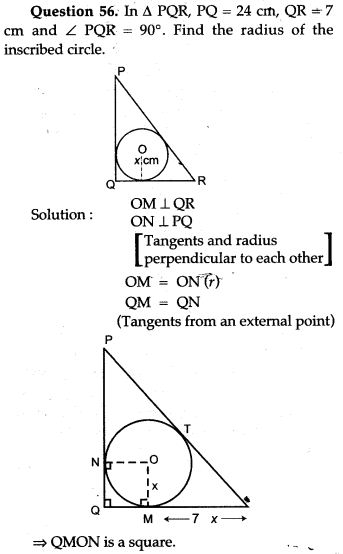
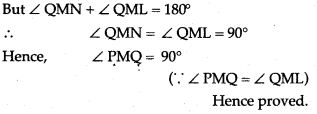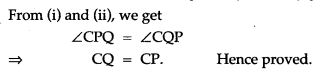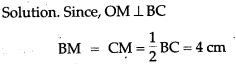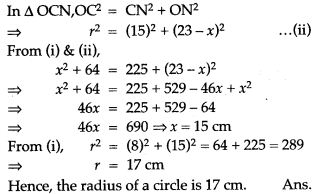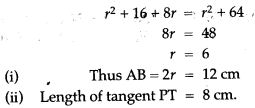Circles Archives

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles)

January 25, 2023May 31, 2022 by Veerendra

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles)

Selina Publishers Concise Mathematics Class 10 ICSE Solutions Chapter 19 Constructions (Circles)

Constructions Circles Exercise 19 – Selina Concise Mathematics Class 10 ICSE Solutions

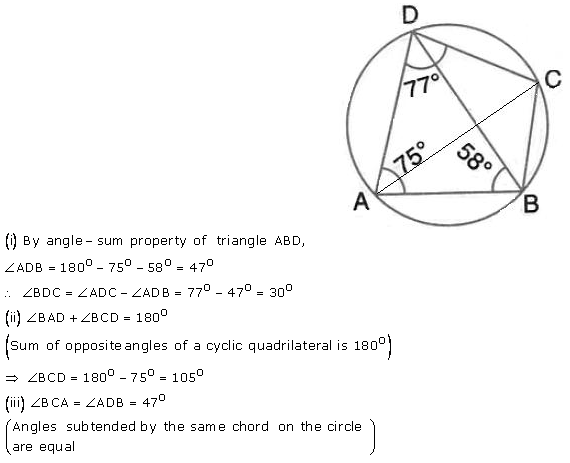
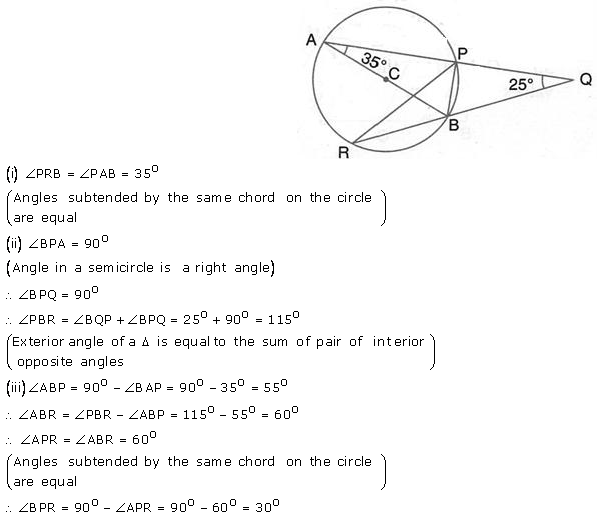
Question 1.
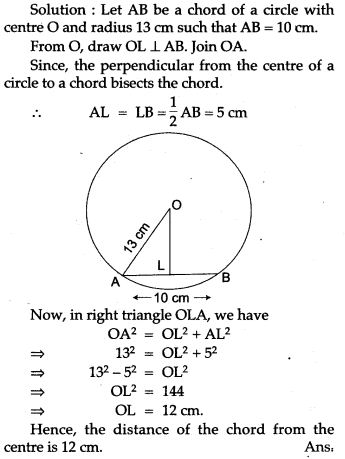
Draw a circle of radius 3 cm. Mark a point P at a distance of 5 cm from the centre of the circle drawn. Draw two tangents PA and PB to the given circle and measure the length of each tangent.
Solution:
Steps of Construction:
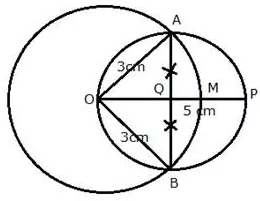
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 1

Draw a circle with centre O and radius 3 cm.
From O, take a point P such that OP = 5 cm
Draw a bisector of OP which intersects OP at M.
With centre M, and radius OM, draw a circle which intersects the given circle at A and B.
Join AP and BP.
AP and BP are the required tangents.
On measuring AP = BP = 4 cm

Question 2.
Draw a circle of diameter of 9 cm. Mark a point at a distance of 7.5 cm from the centre of the circle. Draw tangents to the given circle from this exterior point. Measure the length of each tangent.
Solution:
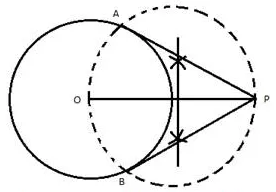
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 2

Draw a circle of diameter 9 cm, taking O as the centre.
Mark a point P outside the circle, such that PO = 7.5 cm.
Taking OP as the diameter, draw a circle such that it cuts the earlier circle at A and B.
Join PA and PB.
Thus, PA and PB are required tangents. PA = PB = 6 cm

Question 3.
Draw a circle of radius 5 cm. Draw two tangents to this circle so that the angle between the tangents is 45º.
Solution:
Steps of Construction:
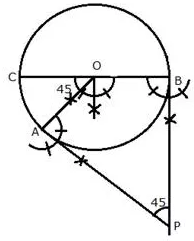
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 3

Draw a circle with centre O and radius BC = 5 cm
Draw arcs making an angle of 180º- 45º = 135º at O such that ∠AOB = 135º
AT A and B, draw two rays making an angle of 90º at each point which meet each other at point P, outside the circle.
AP and BP are the required tangents which make an angle of 45º with each other at P.

Question 4.
Draw a circle of radius 4.5 cm. Draw two tangents to this circle so that the angle between the tangents is 60º.
Solution:
Steps of Construction:
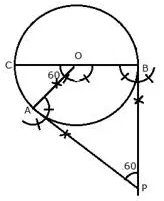
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 4

Draw a circle with centre O and radius BC = 4.5 cm
Draw arcs making an angle of 180º – 60º = 120º at O such that ∠AOB = 120º
AT A and B, draw two rays making an angle of 90º at each point which meet each other at point P, outside the circle.
AP and BP are the required tangents which make an angle of 60º with each other at P.

Question 5.
Using ruler and compasses only, draw an equilateral triangle of side 4.5 cm and draw its circumscribed circle. Measure the radius of the circle.
Solution:
Steps of construction:
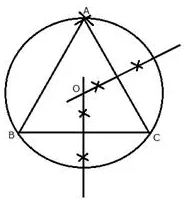
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 5

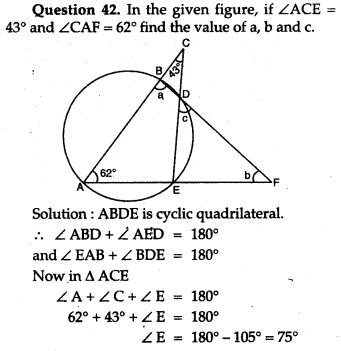
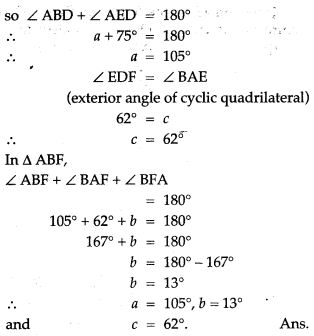
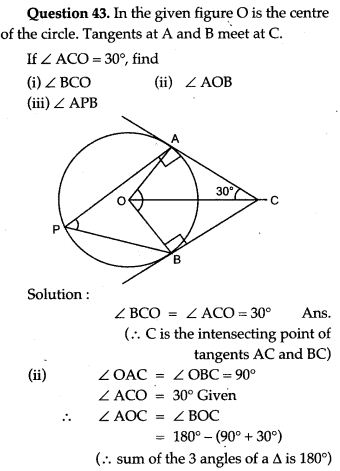
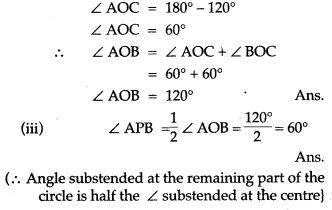
Draw a line segment BC = 4.5 cm
With centers B and C, draw two arcs of radius 4.5 cm which intersect each other at A.
Join AC and AB.
Draw perpendicular bisectors of AC and BC intersecting each other at O.
With centre O, and radius OA or OB or OC draw a circle which will pass through A, B and C.
This is the required circumcircle of triangle ABC.
On measuring the radius OA = 2.6 cm

Question 6.
Using ruler and compasses only.
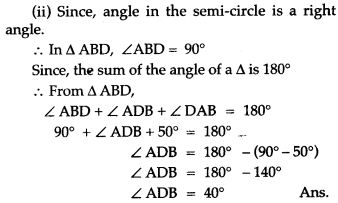
(i) Construct triangle ABC, having given BC = 7 cm, AB – AC = 1 cm and ∠ABC = 45°.
(ii) Inscribe a circle in the ∆ABC constructed in (i) above. Measure its radius.
Solution:
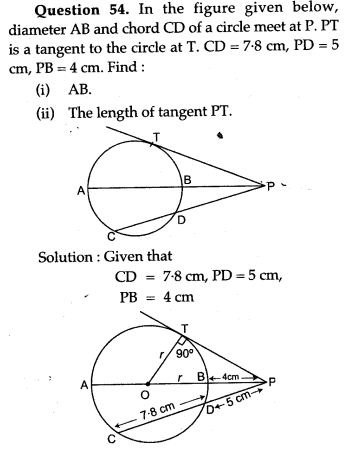
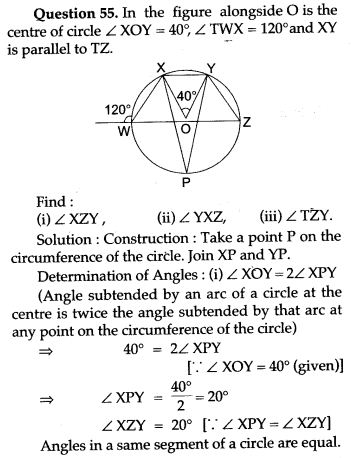
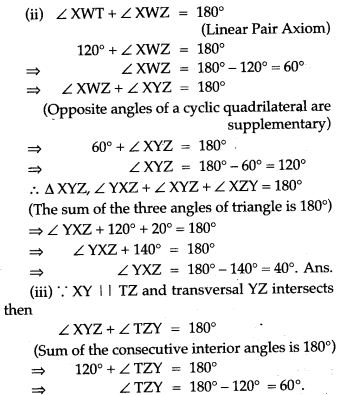
Steps of Construction:
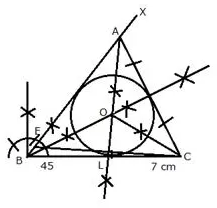
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 6
i) Construction of triangle:

Draw a line segment BC = 7 cm
At B, draw a ray BX making an angle of 45º and cut off BE = AB – AC = 1 cm
Join EC and draw the perpendicular bisector of EC intersecting BX at A.
Join AC.
∆ABC is the required triangle.

ii) Construction of incircle:

Draw angle bisectors of ∠ABC and ∠ACB intersecting each other at O.
From O, draw perpendiculars OL to BC.
O as centre and OL as radius draw circle which touches the sides of the ∆ABC. This is the required in-circle of ∆ABC.
On measuring, radius OL = 1.8 cm

Question 7.
Using ruler and compasses only, draw an equilateral triangle of side 5 cm. Draw its inscribed circle. Measure the radius of the circle.
Solution:
Steps of Construction:
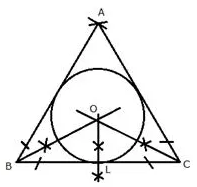
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 7

Draw a line segment BC = 5 cm
With centers B and C, draw two arcs of 5 cm radius each which intersect each other at A.
Join AB and AC.
Draw angle bisectors of ∠B and ∠C intersecting each other at O.
From O, draw OL ⊥ BC.
Now with centre O and radius OL, draw a circle which will touch the sides of ∆ABC
On measuring, OL = 1.4 cm

Question 8.
Using ruler and compasses only,
(i) Construct a triangle ABC with the following data:
Base AB = 6 cm, BC = 6.2 cm and ∠CAB – 60°
(ii) In the same diagram, draw a circle which passes through the points A, B and C and mark its centre as O.
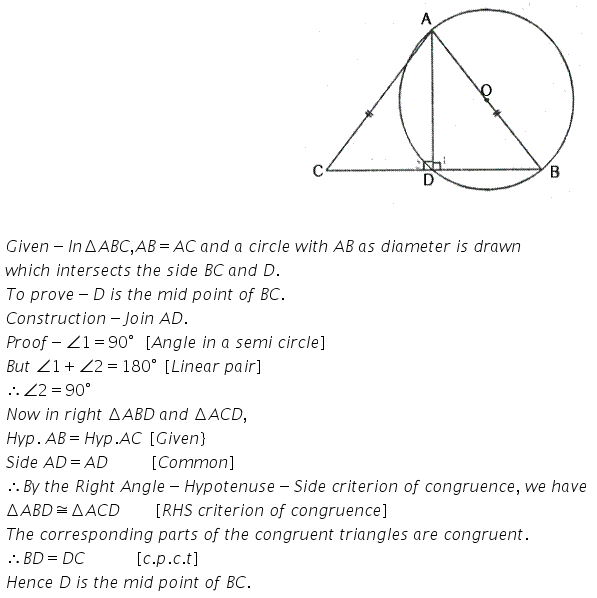
(iii) Draw a perpendicular from O to AB which meets AB in D.
(iv) Prove that AD = BD
Solution:
Steps of Construction:
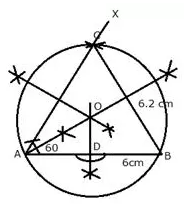
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 8

Draw a line segment AB = 6 cm
At A, draw a ray making an angle of 60º with BC.
With B as centre and radius = 6.2 cm draw an arc which intersects AX ray at C.
Join BC.
∆ABC is the required triangle.
Draw the perpendicular bisectors of AB and AC intersecting each other at O.
With centre O, and radius as OA or OB or OC, draw a circle which will pass through A, B and C.
From O, draw OD ⊥ AB.
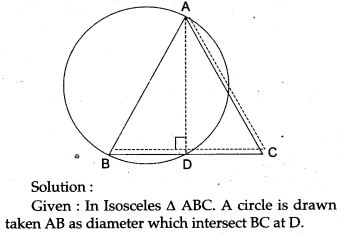
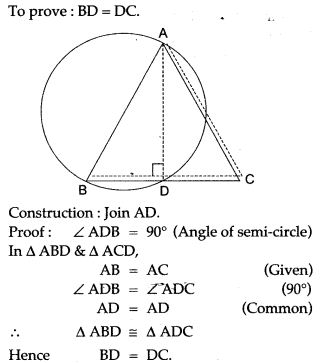
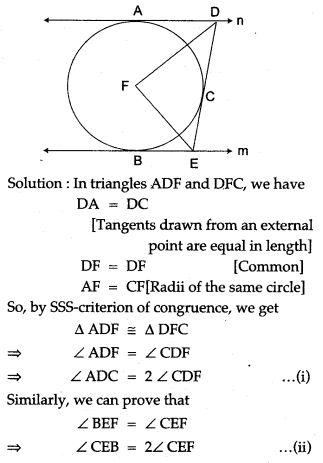
Proof: In right ∆OAD and ∆OBD
OA = OB (radii of same circle)
Side OD = OD (common)
∴ ∆OAD ≅ ∆OBD (RHS)
⇒ AD = BD (CPCT)

Question 9.
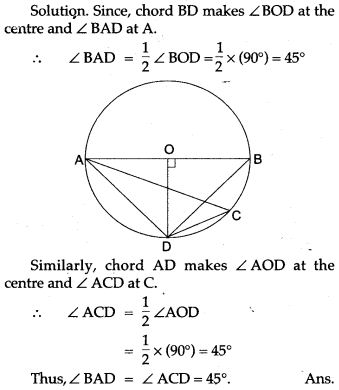
Using ruler and compasses only construct a triangle ABC in which BC = 4 cm, ∠ACB = 45° and perpendicular from A on BC is 2.5 cm. Draw a circle circumscribing the triangle ABC.
Solution:
Steps of Construction:
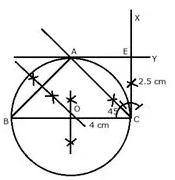
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 9

Draw a line segment BC = 4 cm.
At C, draw a perpendicular line CX and from it, cut off CE = 2.5 cm.
From E, draw another perpendicular line EY.
From C, draw a ray making an angle of 45º with CB, which intersects EY at A.
Join AB.
∆ABC is the required triangle.
Draw perpendicular bisectors of sides AB and BC intersecting each other at O.
With centre O, and radius OB, draw a circle which will pass through A, B and C.
Measuring the radius OB = OC = OA = 2 cm

Question 10.
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
(i) What do you call the point O?
(ii) What is the relation between the distances OA, OB and OC?
(iii) Does the perpendicular bisector of BC pass through O?
Solution:
Steps of Construction:
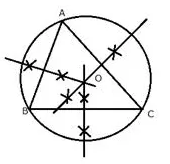
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 10

O is called the circumcentre of circumcircle of ∆ABC.
OA, OB and OC are the radii of the circumcircle.
Yes, the perpendicular bisector of BC will pass through O.

Question 11.
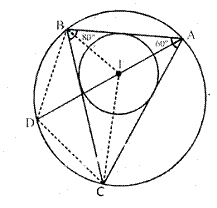
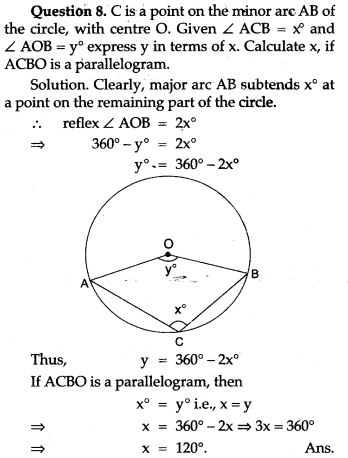
The bisectors of angles A and B of a scalene triangle ABC meet at O.
i) What is the point O called?
ii) OR and OQ are drawn perpendiculars to AB and CA respectively. What is the relation between OR and OQ?
iii) What is the relation between angle ACO and angle BCO?
Solution:
Steps of Construction:
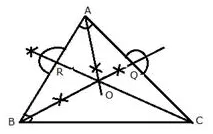
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 11

O is called the incentre of the incircle of ∆ABC.
OR and OQ are the radii of the incircle and OR = OQ.
OC is the bisector of angle C
∴ ∠ACO = ∠BCO

Question 12.
i) Using ruler and compasses only, construct a triangle ABC in which AB = 8 cm, BC = 6 cm and CA = 5 cm.
ii) Find its incentre and mark it I.
iii) With I as centre, draw a circle which will cut off 2 cm chords from each side of the triangle.
Solution:
Steps of Construction:
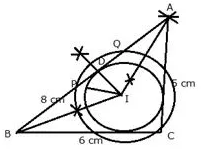
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 12

Draw a line segment BC = 6 cm.
With centre B and radius 8 cm draw an arc.
With centre C and radius 5 cm draw another arc which intersects the first arc at A.
Join AB and AC.
∆ABC is the required triangle.
Draw the angle bisectors of ∠B and ∠A intersecting each other at I. Then I is the incentre of the triangle ABC
Through I, draw ID ⊥ AB
Now from D, cut off \(D P=D Q=\frac{2}{2}=1 \mathrm{cm}\)
With centre I, and radius IP or IQ, draw a circle which will intersect each side of triangle ABC cutting chords of 2 cm each.

Question 13.
Construct an equilateral triangle ABC with side 6 cm. Draw a circle circumscribing the triangle ABC.
Solution:
Steps of Construction:
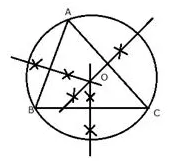
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 13

Draw a line segment BC = 6 cm
With centers B and C, draw two arcs of radius 6 cm which intersect each other at A.
Join AC and AB.
Draw perpendicular bisectors of AC, AB and BC intersecting each other at O.
With centre O, and radius OA or OB or OC draw a circle which will pass through A, B and C.
This is the required circumcircle of triangle ABC.

Question 14.
Construct a circle, inscribing an equilateral triangle with side 5.6 cm.
Solution:
Steps of Construction:
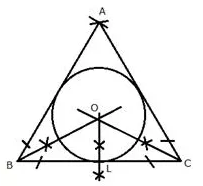
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 14

Draw a line segment BC = 5.6 cm
With centers B and C, draw two arcs of 5.6 cm radius each which intersect each other at A.
Join AB and AC.
Draw angle bisectors of ∠B and ∠Cintersecting each other at O.
From O, draw OL ⊥ BC.
Now with centre O and radius OL, draw a circle which will touch the sides of ∆ABC
This is the required circle.

Question 15.
Draw a circle circumscribing a regular hexagon of side 5 cm.
Solution:
Steps of Construction:
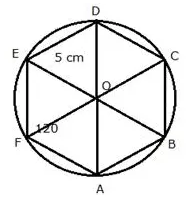
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 15

Draw a regular hexagon ABCDEF with each side equal to 5 cm and each interior angle 120º.
Join its diagonals AD, BE and CF intersecting each other at O.
With centre as O and radius OA, draw a circle which will pass through the vertices A, B, C, D, E and F.
This is the required circumcircle.

Question 16.
Draw an inscribing circle of a regular hexagon of side 5.8 cm.
Solution:
Steps of Construction:
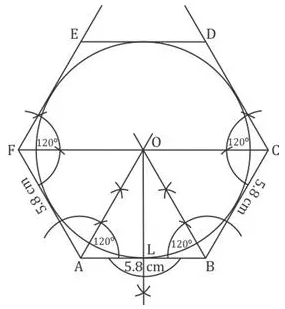
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 16

Draw a line segment AB = 5.8 cm
At A and B, draw rays making an angle of 120° each and cut off AF = BC = 5.8 cm
Again F and C, draw rays making an angle of 120° each and cut off FE = CD = 5.8 cm.
Join DE. Then ABCDEF is the regular hexagon.
Draw the bisectors of ∠A and ∠B intersecting each other at O.
From O, draw OL ⊥ AB
With centre O and radius OL, draw a circle which touches the sides of the hexagon.
This is the required in circle of the hexagon.

Question 17.
Construct a regular hexagon of side 4 cm. Construct a circle circumscribing the hexagon.
Solution:
Steps of Construction:
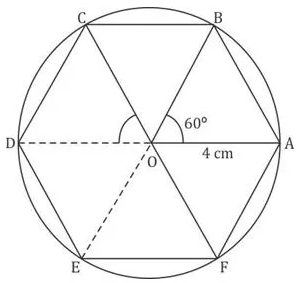
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 17

Draw a circle of radius 4 cm with centre O
Since the interior angle of regular hexagon is 60o, draw radii OA and OB such that ∠AOB = 60°
Cut off arcs BC, CD, EF and each equal to arc AB on given circle
Join AB, BC, CD, DE, EF, FA to get required regular hexagon ABCDEF in a given circle.
The circle is the required circum circle, circumscribing the hexagon.

Question 18.
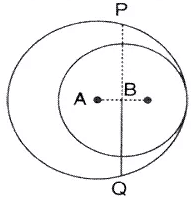
Draw a circle of radius 3.5 cm. Mark a point P outside the circle at a distance of 6 cm from the centre. Construct two tangents from P to the given circle. Measure and write down the length of one tangent.
Solution:
Steps of Construction:
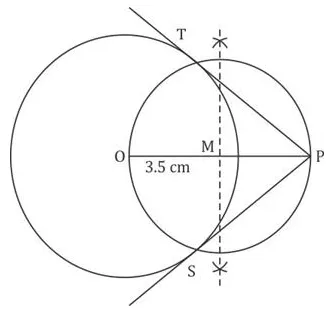
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 18

Draw a line segment OP = 6 cm
With centre O and radius 3.5 cm, draw a circle
Draw the midpoint of OP
With centre M and diameter OP, draw a circle which intersect the circle at T and S
Join PT and PS.
PT and PS are the required tangents. On measuring the length of PT = PS = 4.8 cm

Question 19.
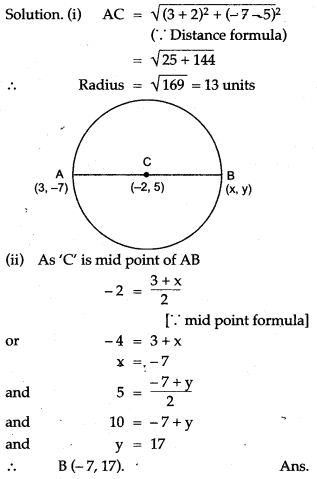
Construct a triangle ABC in which base BC = 5.5 cm, AB = 6 cm and m∠ABC =120˚.
i. Construct a circle circumscribing the triangle ABC.
ii. Draw a cyclic quadrilateral ABCD so that D is equidistant from B and C.
Solution:
Steps of Construction:
i.
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 19
a. Draw a line BC = 5.4 cm.
b. Draw AB = 6 cm, such that m ∠ABC = 120°.
c. Construct the perpendicular bisectors of AB and BC, such that they intersect at O.
d. Draw a circle with O as the radius.
ii.
(e) Extend the perpendicular bisector of BC, such that
it intersects the circle at D.
(f) Join BD and CD.
(g) Here BD = DC.

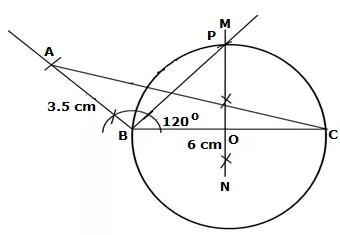
Question 20.
Using a ruler and compasses only :
(i) Construct a triangle ABC with the following data: AB = 3.5 cm, BC = 6 cm and ∠ABC = 120°.
(ii) In the same diagram, draw a circle with BC as diameter. Find a point P on the circumference of the circle which is equidistant from AB and BC.
(iii) Measure ∠BCP.
Solution:
Steps of constructions:

1. Draw a line segment BC = 6 cm.
At B, draw a ray BX making an angle of 120° with BC.
With B as centre and radius 3.5 cm, cut-off AB = 3.5 cm.
Join AC
Thus, ABC is the required triangle.

2. Draw perpendicular bisector MN of BC which cuts BC at point o.
With O as centre and radius = OB, draw a circle.
Draw angle bisector of ∠ABC which meets the cirde at point P.
Thus, point P is equidistant from AB and BC

3. On measuring, ∠BCP = 30°

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 20

Question 21.
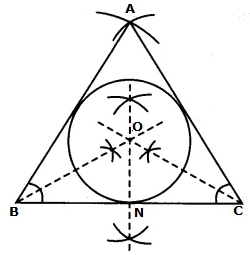
Construct a ∆ABC with BC = 6.5 cm, AB = 5.5 cm, AC = 5 cm. Construct the incircle of the triangle. Measure and record the radius of the incircle.
Solution:
Steps of construction:

Draw BC = 6.5 cm.
With B as centre, draw an arc of radius 5.5 cm.
With C as oentre, draw an arc of radius 5 cm.
Let this arc meets the previous arc at A.
Join AB and AC to get ∆ABC.
Draw the bi sectors of ∠ABC and ∠ACB.
Let these bisectors meet each other at O.
Draw ON ⊥ BC.
With O as centre and radius ON, draw a inarcle that touches all the sides of ∆ABC
By measurement, radius ON = 1.5 cm

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 21

Question 22.
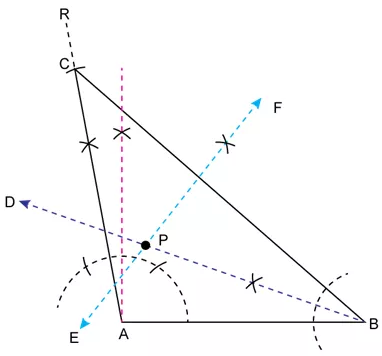
Construct a triangle ABC with AB = 5.5 cm, AC = 6 cm and ∠BAC = 105°. Hence :
(i) Construct the locus of points equidistant from BA and BC.
(ii) Construct the locus of points equidistant from B and C.
(iii) Mark the point which satisfies the above two loci as P. Measure and write the length of PC.
Solution:
Steps of construction:

Draw AB = 5.5 cm
Construct ∠BAR = 1050
With centre A and radius 6 cm, aut off arc on AR at C.
Join BC. ABC is the required triangle.
Draw angle bisector BD of ∠ABC, which is the loss of points equidistant from BA and BC.
Draw perpendicular bisector EF of BC, which is the locus of points equidistant from B and C.
BD and EF intersect each other at point P.
Thus, P satisfies the above two lod.
By measurement, PC = 4.8 cm

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 22
Question 23.
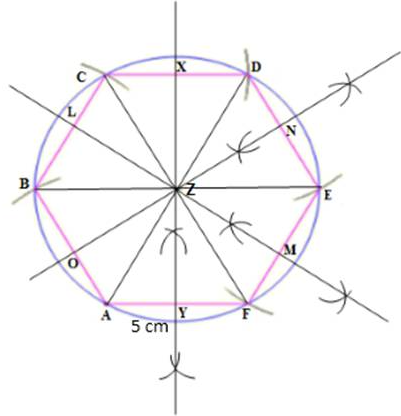
Construct a regular hexagon of side 5 cm. Hence construct all its lines of symmetry and name them. (2016)
Solution:
Steps of construction:

Draw AF measuring 5 cm using a ruler.
With A as the centre and radius equal to AF, draw an arc above AF.
With F as the centre, and same radius cut the previous arc at Z
With Z as the centre, and same radius draw a circle passing through A and F.
With A as the centre and same radius, draw an arc to cut the circle above AF at B.
With B as the centre and same radius, draw an arc to cut the circle at C.
Repeat this process to get remaining vertices of the hexagon at D and E.
Join consecutive arcs on the circle to form the hexagon.
Draw the perpendicular bisectors of AF, FE and DE.
Extend the bisectors of AF, FE and DE to meet CD, BC and AB at X, L and O respectively.
Join AD, CF and EB.

These are the 6 lines of symmetry of the regular hexagon.
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 23

Question 24.
Draw a line AB = 5 cm. Mark a point C on AB such that AC = 3 cm. Using a ruler and a compass only, construct:
(i) A circle of radius 2.5 cm, passing through A and C.
(ii) Construct two tangents to the circle from the external point B. Measure and record the length of the tangents. (2016)
Solution:
Steps for construction:

Draw AB = 5 cm using a ruler.
With A as the centre cut an arc of 3 cm on AB to obtain C.
With A as the centre and radius 2.5 cm, draw an arc above AB.
With same radius, and C as the centre draw an arc to cut the previous arc and mark the intersection as O.
With O as the centre and radius 2.5 cm, draw a circle so that points A and C lie on the circle formed.
Join OB.
Draw the perpendicular bisector of OB to obtain the mid-point of OB, M.
With the M as the centre and radius equal to OM, draw a circle to cut the previous circle at points P and Q.
Join PB and QB. PB and QB are the required tangents to the given circle from exterior point B.

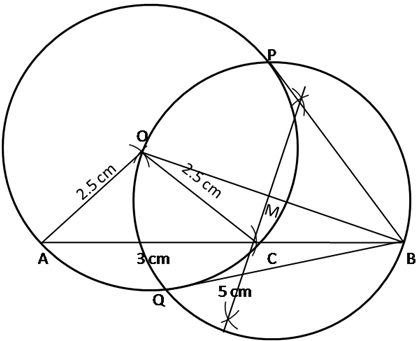
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 24
QB = PB = 3 cm
That is, length of each tangent is 3 cm.

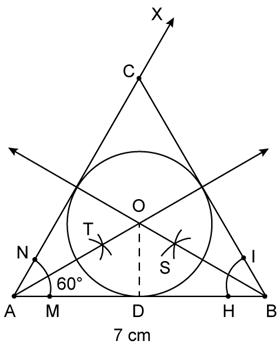
Solution 25.
Steps of construction :

Draw a line AB = 7 cm
Taking P as centre and same radius, draw an arc of a circle which intersects AB at M.
Taking M as centre and with the same radius as before drawn an arc intersecting previously drawn arc, at point N.
Draw the ray AX passing through N, then ∠XAB = 60°
Taking A as centre and radius equal to 5 cm, draw an arc cutting AX at C.
Join BC
The required triangle ABC is obtained.
Draw angle bisector of ∠CAB and ∠ABC
Mark their intersection as O
With O as center, draw a circle with radius OD

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 25

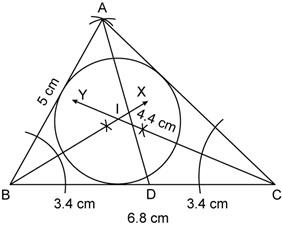
Solution 26.
Steps for construction :

Draw BC = 6.8 cm.
Mark point D where BD = DC = 3.4 cm which is mid-point of BC.
Mark a point A which is intersection of arcs AD = 4.4 cm and AB = 5 cm from a point D and B respectively.
Join AB, AD and AC. ABC is the required triangle.
Draw bisectors of angle B and angle C which are ray BX and CY where I is the incentre of a circle.
Draw incircle of a triangle ABC.

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 26

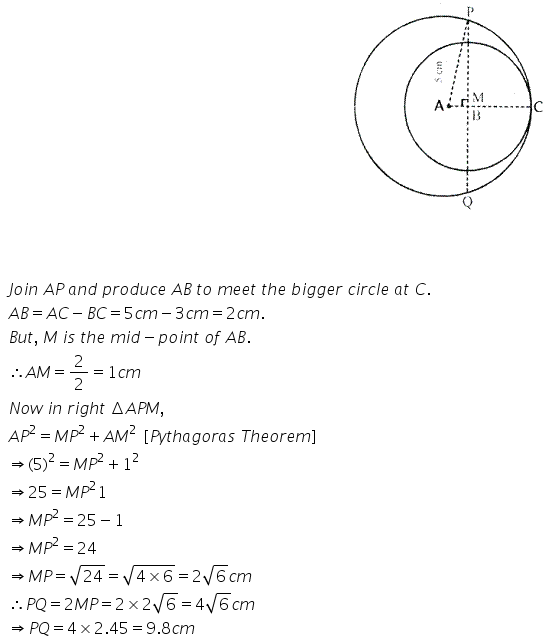
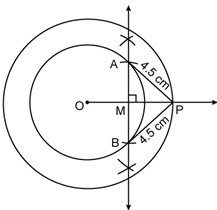
Solution 27.
Steps for construction :

Draw concentric circles of radius 4 cm and 6 cm with centre of O.
Take point P on the outer circle.
Join OP.
Draw perpendicular bisectors of OP where M is the midpoint of OP.
Take a distance of a point O from the point M and mark arcs from M on the inner circle it cuts at point A and B respectively.
Join PA and PB.

We observe that PA and PB are tangents from outer circle to inner circle are equal of a length 4.5 cm each.
Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 27

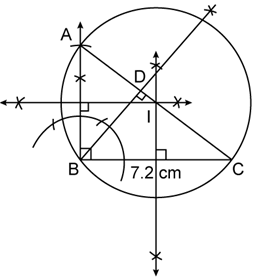
Solution 28.
Steps for construction :

Draw BC = 7.2 cm.
Draw an angle ABC = 90°using compass.
Draw BD perpendicular to AC using compass.
Join BD.
Draw perpendicular bisectors of AB and BC which intersect at I, where I is the circumcentre of a circle.
Draw circumcircle using circumcentre I. we get radius of a circle is 4.7 cm.

Selina Concise Mathematics Class 10 ICSE Solutions Constructions (Circles) image - 28

More Resources for Selina Concise Class 10 ICSE Solutions

ICSE Solutions Selina ICSE Solutions

Selina Concise Mathematics Class 10 ICSE Solutions Circles

November 10, 2023May 26, 2022 by Veerendra

Selina Concise Mathematics Class 10 ICSE Solutions Circles

Selina Publishers Concise Mathematics Class 10 ICSE Solutions Chapter 17 Circles

Circles Exercise 17A – Selina Concise Mathematics Class 10 ICSE Solutions

Circles Class 10 Question 1.
In the given figure, O is the centre of the circle. ∠OAB and ∠OCB are 30° and 40° respectively. Find ∠AOC. Show your steps of working.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 1
Solution:

Question 2.
In the given figure, ∠BAD = 65°, ∠ABD = 70°, ∠BDC = 45°
(i) Prove that AC is a diameter of the circle.
(ii) Find ∠ACB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 3
Solution:

Question 3.
Given O is the centre of the circle and ∠AOB = 70°. Calculate the value of:
(i) ∠ OCA,
(ii) ∠OAC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 5
Solution:

Question 4.
In each of the following figures, O is the centre of the circle. Find the values of a, b, and c.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 7
Solution:

Question 5.
In each of the following figures, O is the centre of the circle. Find the value of a, b, c and d.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 9
Solution:

Question 6.
In the figure, AB is common chord of the two circles. If AC and AD are diameters; prove that D, B and C are in a straight line. O₁ and O₂ are the centres of two circles.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 12
Solution:

Question 7.
In the figure given beow, find :
(i) ∠ BCD,
(ii) ∠ ADC,
(iii) ∠ ABC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 14
Show steps of your workng.
Solution:

Question 8.
In the given figure, O is centre of the circle. If ∠ AOB = 140° and ∠ OAC = 50°; find :
(i) ∠ ACB,
(ii) ∠ OBC,
(iii) ∠ OAB,
(iv) ∠CBA
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 16
Solution:

Question 9.
Calculate :
(i) ∠ CDB,
(ii) ∠ ABC,
(iii) ∠ ACB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 18
Solution:

Question 10.
In the figure given below, ABCD is a eyclic quadrilateral in which ∠ BAD = 75°; ∠ ABD = 58° and ∠ADC = 77°. Find:
(i) ∠ BDC,
(ii) ∠ BCD,
(iii) ∠ BCA.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 20
Solution:

Question 11.
In the following figure, O is centre of the circle and ∆ ABC is equilateral. Find :
(i) ∠ ADB
(ii) ∠ AEB
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 22
Solution:

Question 12.
Given—∠ CAB = 75° and ∠ CBA = 50°. Find the value of ∠ DAB + ∠ ABD
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 24
Solution:

Question 13.
ABCD is a cyclic quadrilateral in a circle with centre O.
If ∠ ADC = 130°; find ∠ BAC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 26
Solution:

Question 14.
In the figure given below, AOB is a diameter of the circle and ∠ AOC = 110°. Find ∠ BDC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 28
Solution:

Question 15.
In the following figure, O is centre of the circle,
∠ AOB = 60° and ∠ BDC = 100°.
Find ∠ OBC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 30

Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 31

Question 16.
ABCD is a cyclic quadrilateral in which ∠ DAC = 27°; ∠ DBA = 50° and ∠ ADB = 33°.
Calculate :
(i) ∠ DBC,
(ii) ∠ DCB,
(iii) ∠ CAB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 32
Solution:

Question 17.
In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If ∠AOC = 80° and ∠CDE = 40°. Find the number of degrees in:
(i) ∠DCE;
(ii) ∠ABC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 34
Solution:

Question 17 (old).
In the figure given below, AB is diameter of the circle whose centre is O. Given that:
∠ ECD = ∠ EDC = 32°.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 36
Show that ∠ COF = ∠ CEF.
Solution:

Question 18.
In the figure given below, AC is a diameter of a circle, whose centre is O. A circle is described on AO as diameter. AE, a chord of the larger circle, intersects the smaller circle at B. Prove that AB = BE.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 38
Solution:

Question 19.
In the following figure,
(i) if ∠BAD = 96°, find BCD and
(ii) Prove that AD is parallel to FE.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 40
Solution:

Question 20.
Prove that:
(i) the parallelogram, inscribed in a circle, is a rectangle.
(ii) the rhombus, inscribed in a circle, is a square.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 42

Question 21.
In the following figure, AB = AC. Prove that DECB is an isosceles trapezium.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 44
Solution:

Question 22.
Two circles intersect at P and Q. Through P diameters PA and PB of the two circles are drawn. Show that the points A, Q and B are collinear.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 46

Question 23.
The figure given below, shows a circle with centre O. Given: ∠ AOC = a and ∠ ABC = b.
(i) Find the relationship between a and b
(ii) Find the measure of angle OAB, if OABC is a parallelogram.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 47
Solution:

Question 24.
Two chords AB and CD intersect at P inside the circle. Prove that the sum of the angles subtended by the arcs AC and BD as the centre O is equal to twice the angle APC.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 49

Question 24 (old).
ABCD is a quadrilateral inscribed in a circle having ∠A = 60°; O is the centre of the circle. Show that: ∠OBD + ∠ODB = ∠CBD + ∠CDB
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 50

Question 25.
In the figure given RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°
Calculate:
(i) ∠RNM;
(ii) ∠NRM.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 51
Solution:

Question 26.
In the figure given alongside, AB || CD and O is the centre of the circle. If ∠ ADC = 25°; find the angle AEB. Give reasons in support of your answer.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 53
Solution:

Question 27.
Two circles intersect at P and Q. Through P, a straight line APB is drawn to meet the circles in A and B. Through Q, a straight line is drawn to meet the circles at Cand D. Prove that AC is parallel to BD.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 55
Solution:

Question 28.
ABCD is a cyclic quadrilateral in which AB and DC on being produced, meet at P such that PA = PD. Prove that AD is parallel to BC.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 57

Question 29.
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find:
(i) ∠PRB
(ii) ∠PBR
(iii) ∠BPR.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 58
Solution:

Question 30.
In the given figure, SP is the bisector of angle RPT and PQRS is a cyclic quadrilateral. Prove that: SQ = SR.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 60
Solution:

Question 31.
In the figure, O is the centre of the circle, ∠AOE = 150°, DAO = 51°. Calculate the sizes of the angles CEB and OCE.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 62
Solution:

Question 32.
In the figure, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 64
Solution:

Question 33.
The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given that ∠APB = a°. Calculate, in terms of a°, the value of:
(i) obtuse ∠AOB
(ii) ∠ACB
(iii) ∠ADB.
Give reasons for your answers clearly.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 66
Solution:

Question 34.
In the given figure, O is the centre of the circle and ∠ ABC = 55°. Calculate the values of x and y.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 68
Solution:

Question 35.
In the given figure, A is the centre of the circle, ABCD is a parallelogram and CDE is a straight line. Prove that ∠BCD = 2∠ABE
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 70
Solution:

Question 36.
ABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given ∠BED = 65°; calculate:
(i) ∠ DAB,
(ii) ∠BDC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 72
Solution:

Question 37.
∠ In the given figure, AB is a diameter of the circle. Chord ED is parallel to AB and ∠ EAB = 63°; calculate:
(i) ∠EBA,
(ii) BCD.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 74
Solution:

Question 38.
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°; calculate:
(i) ∠ DAB,
(ii) ∠ DBA,
(iii) ∠ DBC,
(iv) ∠ ADC.
Also, show that the ∆AOD is an equilateral triangle.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 76
Solution:

Question 39.
In the given figure, I is the incentre of the ∆ ABC. Bl when produced meets the circumcirle of ∆ ABC at D. Given ∠BAC = 55° and ∠ ACB = 65°, calculate:
(i) ∠DCA,
(ii) ∠ DAC,
(iii) ∠DCI,
(iv) ∠AIC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 78
Solution:

Question 40.
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that:
(i) ∠ABC = 2 ∠APQ
(ii) ∠ACB = 2 ∠APR
(iii) ∠QPR = 90° – \(\frac{1}{2}\)BAC
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 80
Solution:

Question 40 (old).
The sides AB and DC of a cyclic quadrilateral ABCD are produced to meet at E; the sides DA and CB are produced to meet at F. If ∠BEC = 42° and ∠BAD = 98°; calculate:
(i) ∠AFB,
(ii) ∠ADC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 82
Solution:

Question 41.
Calculate the angles x, y and z if: \(\frac{x}{3}=\frac{y}{4}=\frac{z}{5}\)
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 84
Solution:

Question 42.
In the given figure, AB = AC = CD and ∠ADC = 38°. Calculate:
(i) Angle ABC
(ii) Angle BEC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 86
Solution:

Question 43.
In the given figure, AC is the diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, and r in terms of x.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 88
Solution:

Question 44.
In the given figure, AC is the diameter of circle, centre O. CD and BE are parallel. Angle AOB = 80° and angle ACE = 10°. Calculate:
(i) Angle BEC;
(ii) Angle BCD;
(iii) Angle CED.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 90
Solution:

Question 45.
In the given figure, AE is the diameter of circle. Write down the numerical value of ∠ABC + ∠CDE. Give reasons for your answer.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 92
Solution:

Question 46.
In the given figure, AOC is a diameter and AC is parallel to ED. If ∠CBE = 64°, calculate ∠DEC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 94
Solution:

Question 47.
Use the given figure to find
(i) ∠BAD
(ii) ∠DQB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 96
Solution:

Question 48.
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠ CAB = x°; find (in terms of x) the values of:
(i) ∠COB
(ii) ∠DOC
(iii) ∠DAC
(iv) ∠ADC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 98
Solution:

Question 49.
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130°. Find:
(i) ∠DAB
(ii) ∠DBA
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 100
Solution:

Question 50.
In the given figure, PQ is the diameter of the circle whose centre is O. Given ∠ROS = 42°; calculate ∠RTS.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 102
Solution:

Question 51.
In the given figure, PQ is a diameter. Chord SR is parallel to PQ. Given that ∠PQR = 58°; calculate
(i) ∠RPQ
(ii) ∠STP.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 104
Solution:

Question 52.
AOD = 60°; calculate the numerical values of:
AB is the diameter of the circle with centre O. OD is parallel to BC and ∠AOD = 60°; calculate the numerical values of:
(i) ∠ABD,
(ii) ∠DBC,
(iii) ∠ADC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 106
Solution:

Question 53.
In the given figure, the centre of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40″; find:
(i) ∠AOB,
(ii) ∠ACB,
(iii) ∠ABD,
(iv) ∠ADB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 108
Solution:

Question 54.
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°; find:
(i) ∠BCD,
(ii) ∠ACB.
Hence, show that AC is a diameter.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 110
Solution:

Question 55.
In a cyclic quadrilateral ABCD, ∠A : ∠C = 3 : 1 and ∠B : ∠D = 1 : 5; find each angle of the quadrilateral.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 112

Question 56.
The given figure shows a circle with centre O and ∠ABP = 42°. Calculate the measure of
(i) ∠PQB
(ii) ∠QPB + ∠PBQ
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 195
Solution:

Question 57.
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If ∠ MAD =x and ∠BAC = y.
(i) express ∠AMD in terms of x.
(ii) express ∠ABD in terms of y.
(iii) prove that : x = y
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 197
Solution:

Question 61 (old).
In a circle, with centre O, a cyclic quadrilateral ABCD is drawn with AB as a diameter of the circle and CD equal to radius of the circle. If AD and BC produced meet at point P; show that ∠APB = 60°.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 199

Circles Exercise 17B – Selina Concise Mathematics Class 10 ICSE Solutions

Question 1.
In a cyclic-trapezium, the non-parallel sides are equal and the diagonals are also equal.
Prove it.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 200

Question 2.
In the following figure, AD is the diameter of the circle with centre 0. chords AB, BC and CD are equal. If ∠DEF = 110°, calculate:
(i) ∠ AFE,
(ii) ∠FAB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 201

Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 202

Question 3.
If two sides of a cycli-quadrilateral are parallel; prove thet:
(i) its other two side are equal.
(ii) its diagonals are equal.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 203

Question 4.
The given figure show a circle with centre O. also, PQ = QR = RS and ∠PTS = 75°. Calculate:
(i) ∠POS,
(ii) ∠ QOR,
(iii) ∠PQR.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 204
Solution:

Question 5.
In the given figure, AB is a side of a regular six-sided polygon and AC is a side of a regular eight-sided polygon inscribed in the circle with centre O. calculate the sizes of:
(i) ∠ AOB,
(ii) ∠ ACB,
(iii) ∠ABC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 206
Solution:

Question 6.
In a regular pentagon ABCDE, inscribed in a circle; find ratio between angle EDA and angel ADC.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 208

Question 7.
In the given figure. AB = BC = CD and ∠ABC = 132°, calculate:
(i) ∠AEB,
(ii) ∠ AED,
(iii) ∠COD.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 209
Solution:

Question 8.
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find:
(i) ∠ CAB,
(ii) ∠ADB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 211
Solution:

Question 9.
The figure shows a circle with centre O. AB is the side of regular pentagon and AC is the side of regular hexagon. Find the angles of triangle ABC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 213
Solution:

Question 10.
In the given figire, BD is a side of a regularhexagon, DC is a side of a regular pentagon and AD is adiameter. Calculate:
(i) ∠ ADC
(ii) ∠BAD,
(iii) ∠ABC
(iv) ∠ AEC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 215
Solution:

Circles Exercise 17C – Selina Concise Mathematics Class 10 ICSE Solutions

Question 1.
In the given circle with diametre AB, find the value of x.

Selina Concise Mathematics Class 10 ICSE Solutions Circles - 218
Solution:

∠ABD = ∠ACD = 30° (Angle in the same segment)
Now in ∆ ADB,
∠BAD + ∠ADB + ∠DBA = 180° (Angles of a A)
But ∠ADB = 90° (Angle in a semi-circle)
∴ x + 90° + 30° = 180° ⇒ x + 120° = 180°
∴ x= 180° – 120° = 60° Ans.

Question 1.
In the given figure, O is the centre of the circle with radius 5 cm, OP and OQ are perpendiculars to AB and CD respectively. AB = 8cm and CD = 6cm. Determine the length of PQ.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 220
Solution:

Selina Concise Mathematics Class 10 ICSE Solutions Circles - 221

Question 2.
In the given figure, ABC is a triangle in which ∠ BAC = 30° Show that BC is equal to the radius of the circum-circle of the triangle ABC, whose centre is O.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 222
Solution:

Question 3.
Prove that the circle drawn on any one a the equalside of an isoscele triangle as diameter bisects the base.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 224

Question 3 (old).
The given figure show two circles with centres A and B; and radii 5 cm and 3cm respectively, touching each other internally. If the perpendicular bisector of AB meets the bigger circle in P and Q, find the length of PQ.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 225
Solution:

Question 4.
In the given figure, chord ED is parallel to diameter AC of the circle. Given ∠ CBE = 65°, calculate ∠DEC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 227
Solution:

Question 5.
The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic. Prove it.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 229

Question 6.
In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate:
(i) ∠BDC
(ii) ∠BEC
(iii) ∠BAC
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 230
Solution:

Question 7.
D and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD = AE. Provet that the points B, C, E and D are concyclic.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 232

Question 7 (old).
Chords AB and CD of a circle intersect each other at point P such that AP = CP.
Show that: AB = CD.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 233
Solution:

Question 8.
In the given rigure, ABCD is a cyclic eqadrilateral. AF is drawn parallel to CB and DA is produced to point E. If ∠ ADC = 92°, ∠ FAE = 20°; determine ∠ BCD. Given reason in support of your answer.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 235
Solution:

Question 9.
If I is the incentre of triangle ABC and Al when produced meets the cicrumcircle of triangle ABC in points D. if ∠ BAC = 66° and ∠ = 80o.calculate:
(i) ∠ DBC
(ii) ∠ IBC
(iii) ∠ BIC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 237
Solution:

Question 10.
In the given figure, AB = AD = DC = PB and ∠ DBC = x°. Determine, in terms of x:
(i) ∠ ABD,
(ii) ∠ APB.
Hence or otherwise, prove thet AP is parallel to DB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 240
Solution:

Question 11.
In the given figure; ABC, AEQ and CEP are straight lines. Show that ∠APE and ∠ CQE are supplementary.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 242
Solution:

Question 12.
In the given, AB is the diameter of the circle with centre O.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 244
If ∠ ADC = 32°, find angle BOC.
Solution:

Question 13.
In a cyclic-quadrilateral PQRS, angle PQR = 135°. Sides SP and RQ prouduced meet at point A: whereas sides PQ and SR produced meet at point B.
If ∠A: ∠B = 2 : 1;find angles A and B.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 246

Question 17 (old).
If the following figure, AB is the diameter of a circle with centre O and CD is the chord with lengh equal radius OA.
If AC produced and BD produed meet at point p; show that ∠APB = 60°
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 248
Solution:

Question 14.
In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 251
If the bisector of angle A meet BC at point E and the given circle at point F, prove that:
(i) EF = FC
(ii) BF =DF
Solution:

Question 15.
ABCD is a cyclic quadrilateral. Sides AB and DC produced meet at point e; whereas sides BC and AD produced meet at point F. I f ∠ DCF : ∠F : ∠E = 3 : 5 : 4, find the angles of the cyclic quadrilateral ABCD.
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 253

Question 16.
The following figure shows a cicrcle with PR as its diameter. If PQ = 7 cm and QR = 3RS = 6 cm, Find the perimetre of the cyclic quadrilateral PORS.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 255
Solution:

Question 17.
In the following figure, AB is the diameter of a circle with centre O. If chord AC = chord AD.prove that:
(i) arc BC = arc DB
(ii) AB is bisector of ∠ CAD.
Further if the lenghof arc AC is twice the lengthof arc BC find :
(a) ∠ BAC
(b) ∠ ABC
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 257
Solution:

Question 18.
In cyclic quadrilateral ABCD; AD = BC, ∠ = 30° and ∠ = 70°; find;
(i) ∠ BCD
(ii) ∠BCA
(iii) ∠ABC
(iv) ∠ ADC
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 260

Question 19.
In the given figure, ∠ACE = 43° and ∠ = 62°; find the values of a, b and c.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 262
Solution:

Question 20.
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠ BAC = 25°
Find
(i) ∠ CAD
(ii) ∠ CBD
(iii) ∠ ADC
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 264
Solution:

Question 21.
ABCD is a cyclic quadrilateral of a circle with centre o such that AB is a diameter of this circle and the length of the chord CD is equal to the radius of the circle..if AD and BC produced meet at P, show that APB =60°
Solution:
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 266

Question 22.
In the figure, given alongside, CP bisects angle ACB. Show that DP bisects angle ADB.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 267
Solution:

Question 23.
In the figure, given below, AD = BC, ∠ BAC = 30° and ∠ = 70° find:
(i) ∠ BCD
(ii) ∠ BCA
(iii) ∠ ABC
(iv) ∠ADC
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 269
Solution:

Question 24.
In the figure given below, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°. Find :
(i) ∠OBD
(ii) ∠AOB
(iii) ∠BED (2016)
Solution:
i. AD is parallel to BC, i.e., OD is parallel to BC and BD is transversal.
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 271

Selina Concise Mathematics Class 10 ICSE Solutions Circles - 272

Selina Concise Mathematics Class 10 ICSE Solutions Circles - 273

Question 25.
In the figure given, O is the centre of the circle. ∠DAE = 70°. Find giving suitable reasons, the measure of
i. ∠BCD
ii. ∠BOD
iii. ∠OBD
Selina Concise Mathematics Class 10 ICSE Solutions Circles - 274
Solution:
∠DAE and ∠DAB are linear pair
So,
∠DAE + ∠DAB = 180°
∴∠DAB = 110°

Also,
∠BCD + ∠DAB = 180°……Opp. Angles of cyclic quadrilateral BADC
∴∠BCD = 70°
∠BCD = \(\frac { 1 }{ 2 }\) ∠BOD…angles subtended by an arc on the centre and on the circle
∴∠BOD = 140°

In ΔBOD,
OB = OD……radii of same circle
So,
∠OBD =∠ODB……isosceles triangle theorem
∠OBD + ∠ODB + ∠BOD = 180°……sum of angles of triangle
2∠OBD = 40°
∠OBD = 20°

More Resources for Selina Concise Class 10 ICSE Solutions

ICSE Solutions Selina ICSE Solutions

ICSE Solutions for Class 10 Mathematics – Circles

November 10, 2023May 12, 2022 by Veerendra

ICSE Solutions for Class 10 Mathematics – Circles

ICSE Solutions Selina ICSE Solutions

Get ICSE Solutions for Class 10 Mathematics Chapter 15 Circles for ICSE Board Examinations on APlusTopper.com. We provide step by step Solutions for ICSE Mathematics Class 10 Solutions Pdf. You can download the Class 10 Maths ICSE Textbook Solutions with Free PDF download option.

Download Formulae Handbook For ICSE Class 9 and 10

Formulae

Theorems based on chord properties:

Theorem: A straight line drawn from the centre of the circle to bisect a chord, which is not a diameter, is at right angles to the chord.
Conversely, the perpendicular to a chord, from the centre of the circle, bisects the chord.
Theorem: There is one circle, and only one, which passes through three given points not in a straight line.
Theorem: Equal chords of a circle are equidistant from the centre.
Conversely, chords of a circle, equidistant from the centre of the circle, are equal.

Theorems based on Arc and Chord properties:

Theorem: The angle which an arc of a circle subtends at the centre is double, that which it subtends at any point on the remaining part of the circumference.
Theorem: Angles in the same segment of a circle are equal.
Theorem: The angle in a semicircle is a right angle.
Theorem: In equal circles (or, in the same circle), if two arcs subtends equal angles at the centre, they are equal.
Conversely, in equal circles (or, in the same circle), if two arcs are equal, they subtend equal angles at the centre.
Theorem: In equal circles (or, in the same circle), if two chord are equal, they cut off equal arcs.
Conversely, in equal circles (or, in the same circle, if two arcs are equal the chords of the arcs are also equal.

Theorems based on Cyclic properties: ABCD is a cyclic quadrilateral.

icse-solutions-class-10-mathematics-30

Theorem: The opposite angles of a cyclic quadrilateral (quadrilateral inscribed in a circle) are supplementary.
Theorem: The exterior angle of a cyclic quadrilateral is equal to the opposite interior angle.

Theorems based on Tangent Properties:

icse-solutions-class-10-mathematics-30-1

Theorem: The tangent at any point of a circle and the radius through this point are perpendicular to each other.
Theorem: If two circles touch each other, the point of contact lies on the straight line through the centres.
Theorem: From any point outside a circle two tangents can be drawn and they are equal in length.
Theorem: If a chord and a tangent intersect externally, then the product of the lengths of segments of the chord is equal to the square of the length of the tangent from the point of contact to the point of intersection.
Theorem: If a line touches a circle and from the point of contact, a chord is drawn, the angles between the tangent and the chord are respectively equal to the angles in the corresponding alternate segments.

Prove the Following

Question 1. If a diameter of a circle bisect each of the two chords of a circle, prove that the chords are parallel.
Circles-icse-solutions-class-10-mathematics-1

Question 2. If two chords of a circle are equally inclined to the diameter through their point of intersection, prove that the chords are equal.
Circles-icse-solutions-class-10-mathematics-2

Question 3. In the given figure, OD is perpendicular to the chord AB of a circle whose centre is O. If BC is a diameter, show that CA = 2 OD.
Circles-icse-solutions-class-10-mathematics-3

Question 4. In Fig., l is a line intersecting the two concentric circles, whose common centre is O, at the points A, B, C and D. Show that AB = CD.

Circles-icse-solutions-class-10-mathematics-4

Circles-icse-solutions-class-10-mathematics-5

Question 6. ABCD is a cyclic quadrilateral AB and DC are produced to meet in E. Prove that
Circles-icse-solutions-class-10-mathematics-7

Question 7. In an isosceles triangle ABC with AB = AC, a circle passing through B and C intersects the sides. AB, and AC at D and E respectively. Prove that DE || BC.
Circles-icse-solutions-class-10-mathematics-8

Question 8. ABCD is quadrilateral inscribed in circle, having ∠A = 60°, O is the centre of the circle, show that
Circles-icse-solutions-class-10-mathematics-10

Question 9. Two equal circles intersect in P and Q. A straight line through P meets the circles in A and B. Prove that QA = QB
Circles-icse-solutions-class-10-mathematics-11

Circles-icse-solutions-class-10-mathematics-13

Question 11. Two circles are drawn with sides AB, AC of a triangle ABC as diameters. The circles intersect at a point D. Prove that D lies on BC.
Circles-icse-solutions-class-10-mathematics-14

Question 12. In the given figure, PT touches a circle with centre O at R. Diameter SQ when
Circles-icse-solutions-class-10-mathematics-15

Circles-icse-solutions-class-10-mathematics-16

Question 14. Prove that the line segment joining the midpoints of two equal chords of a circle substends equal angles with the chord.
Circles-icse-solutions-class-10-mathematics-17

Question 15. In an equilateral triangle, prove that the centroid and centre of the circum-circle (circumcentre) coincide.
Circles-icse-solutions-class-10-mathematics-19

Question 16. In Fig. AB and CD are two chords of a circle intersecting each other at P such that AP = CP. Show that AB = CD.

Question 17. Prove that the angle bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (Provided they are not parallel) intersect at right angle.
Circles-icse-solutions-class-10-mathematics-23

Question 18. In Fig. P is any point on the chord BC of a circle such that AB = AP. Prove that CP = CQ.
Circles-icse-solutions-class-10-mathematics-25

Question 19. The diagonals of a cyclic quadrilateral are at right angles. Prove that the perpendicular from the point of their intersection on any side when produced backward bisects the opposite side.
Circles-icse-solutions-class-10-mathematics-27

Question 20. In a circle with centre O, chords AB and CD intersect inside the circumference at E. Prove that ∠AOC + ∠BOD = 2∠AEC.
Circles-icse-solutions-class-10-mathematics-29

Question 21. In Fig. ABC is a triangle in which ∠BAC = 30°. Show that BC is the radius of the circum circle of A ABC, whose centre is O.
Circles-icse-solutions-class-10-mathematics-30

Question 22. Prove that the circle drawn on any one of the equal sides of an isosceles triangles as diameter bisects the base.
Circles-icse-solutions-class-10-mathematics-32

Question 23. In Fig. ABCD is a cyclic quadrilateral. A circle passing through A and B meets AD and BC in the points E and F respectively. Prove that EF || DC.
Circles-icse-solutions-class-10-mathematics-34

Circles-icse-solutions-class-10-mathematics-35

Question 25. If PA and PB are two tangent drawn from a point P to a circle with centre C touching it A and B, prove that CP is the perpendicular bisector of AB.
Circles-icse-solutions-class-10-mathematics-37

Question 26. Two circle with radii r₁ and r₂ touch each other externally. Let r be the radius of a circle which touches these two circle as well as a common tangent to the two circles, Prove that:

Circles-icse-solutions-class-10-mathematics-39

Question 27. If AB and CD are two chords which when produced meet at P and if AP = CP, show that AB = CD.
Circles-icse-solutions-class-10-mathematics-40

Question 28. In the figure, PM is a tangent to the circle and PA = AM. Prove that:
Circles-icse-solutions-class-10-mathematics-41

Question 29. In Fig. the incircle of ΔABC, touches the sides BC, CA and AB at D, E respectively. Show that:
Circles-icse-solutions-class-10-mathematics-42

Question 30. In Fig. TA is a tangent to a circle from the point T and TBC is a secant to the circle. If AD is the bisector of ∠BAC, prove that ΔADT is isosceles.

Circles-icse-solutions-class-10-mathematics-44

Question 32. In Fig. l and m are two parallel tangents at A and B. The tangent at C makes an intercept DE between n and m. Prove that ∠ DFE = 90⁰
Circles-icse-solutions-class-10-mathematics-47

Question 33. A circle touches the sides of a quadrilateral ABCD at P, Q, R, S respectively. Show that the angles subtended at the centre by a pair of opposite sides are supplementary.
Circles-icse-solutions-class-10-mathematics-49

Question 34. Two equal chords AB and CD of a circle with centre O, when produced meet at a point E, as shown in Fig. Prove that BE = DE and AE = CE.
Circles-icse-solutions-class-10-mathematics-51

Question 35. Prove that the quadrilateral formed by angle bisectors of a cyclic quadrilateral ABCD is also cyclic.
Circles-icse-solutions-class-10-mathematics-53

Figure Based Questions

Question 1. Two concentric circles with centre 0 have A, B, C, D as the points of intersection with the lines L shown in figure. If AD = 12 cm and BC s = 8 cm, find the lengths of AB, CD, AC and BD.
circles-icse-solutions-class-10-mathematics-1

Question 2. In the given circle with diameter AB, find the value of x.
circles-icse-solutions-class-10-mathematics-3

Question 3. In the given figure, the area enclosed between the two concentric circles is 770 cm². If the radius of outer circle is 21 cm, calculate the radius of the inner circle.
circles-icse-solutions-class-10-mathematics-4

Question 4. Two chords AB and CD of a circle are parallel and a line L is the perpendicular bisector of AB. Show that L bisects CD.
circles-icse-solutions-class-10-mathematics-6

Question 5. In the adjoining figure, AB is the diameter of the circle with centre O. If ∠BCD = 120°, calculate:
circles-icse-solutions-class-10-mathematics-7

Question 6. Find the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 13 cm.
circles-icse-solutions-class-10-mathematics-9

Question 7. The radius of a circle is 13 cm and the length of one of its chord is 10 cm. Find the distance of the chord from the centre.
circles-icse-solutions-class-10-mathematics-10

circles-icse-solutions-class-10-mathematics-11

Question 9. AB is a diameter of a circle with centre C = (- 2, 5). If A = (3, – 7). Find
(i) the length of radius AC
(ii) the coordinates of B.
circles-icse-solutions-class-10-mathematics-12

Question 10. AB is a diameter of a circle with centre O and radius OD is perpendicular to AB. If C is any point on arc DB, find ∠ BAD and ∠ ACD.
circles-icse-solutions-class-10-mathematics-13

Question 11. In the given below figure,
circles-icse-solutions-class-10-mathematics-14

circles-icse-solutions-class-10-mathematics-15

circles-icse-solutions-class-10-mathematics-16

Question 14. In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of the circle.
circles-icse-solutions-class-10-mathematics-17

Question 15. In Fig., chords AB and CD of the circle intersect at O. AO = 5 cm, BO = 3 cm and CO = 2.5 cm. Determine the length of DO.
circles-icse-solutions-class-10-mathematics-19

Question 16. In the figure given below, PT is a tangent to the circle. Find PT if AT = 16 cm and AB = 12 cm.
circles-icse-solutions-class-10-mathematics-20

circles-icse-solutions-class-10-mathematics-21

Question 18. A, B and C are three points on a circle. The tangent at C meets BN produced at T. Given that ∠ ATC = 36° and ∠ ACT = 48°, calculate the angle subtended by AB at the centre of the circle.
circles-icse-solutions-class-10-mathematics-23

Question 19. In the given figure, O is the centre of the circle and ∠PBA = 45°. Calculate the value of ∠PQB.
circles-icse-solutions-class-10-mathematics-24

Question 20. Two chords AB, CD of lengths 16 cm and 30 cm, are parallel. If the distance between AB and CD is 23 cm. Find the radius of the circle.
circles-icse-solutions-class-10-mathematics-25

Question 21. Two circles of radii 10 cm and 8 cm intersect and the length of the common chord is 12 cm. Find the distance between their centres.
circles-icse-solutions-class-10-mathematics-27

Question 22. AB and CD are two chords of a circle such that AB = 6 cm, CD = 12 cm and AB || CD. If the distance between AB and CD is 3 cm, find the radius of the circle.
circles-icse-solutions-class-10-mathematics-29

circles-icse-solutions-class-10-mathematics-31

Question 24. AB and CD are two parallel chords of a circle such that AB = 10 cm and CD = 24 cm. If the chords are on the opposite sides of the centre and the distance between them is 17 cm, find the radius of the circle.
circles-icse-solutions-class-10-mathematics-32

circles-icse-solutions-class-10-mathematics-34

Question 26. In the figure given below,O is the centre of the circle. AB and CD are two chords of the circle. OM is perpendicular to AB and ON is perpendicular to CD.
circles-icse-solutions-class-10-mathematics-36

Question 27. If O is the centre of the circle, find the value of x in each of the following figures
circles-icse-solutions-class-10-mathematics-38

circles-icse-solutions-class-10-mathematics-41

Question 29. In the given figure, AB is the diameter of a circle with centre O.
circles-icse-solutions-class-10-mathematics-43

Question 30. In ABCD is a cyclic quadrilateral; O is the centre of the circle. If BOD = 160°, find the measure of BPD.
circles-icse-solutions-class-10-mathematics-45

circles-icse-solutions-class-10-mathematics-46

Question 32. In the given figure O is the centre of the circle, ∠ BAD = 75° and chord BC = chord CD. Find:
circles-icse-solutions-class-10-mathematics-47

circles-icse-solutions-class-10-mathematics-48

circles-icse-solutions-class-10-mathematics-49

circles-icse-solutions-class-10-mathematics-50

circles-icse-solutions-class-10-mathematics-52

Question 37. ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.
circles-icse-solutions-class-10-mathematics-53

circles-icse-solutions-class-10-mathematics-55

circles-icse-solutions-class-10-mathematics-56

Question 40. P and Q are the centre of circles of radius 9 cm and 2 cm respectively; PQ = 17 cm. R is the centre of circle of radius x cm, which touches the above circles externally, given that ∠ PRQ = 90°. Write an equation in x and solve it.
circles-icse-solutions-class-10-mathematics-58

circles-icse-solutions-class-10-mathematics-59

circles-icse-solutions-class-10-mathematics-61

circles-icse-solutions-class-10-mathematics-63

circles-icse-solutions-class-10-mathematics-65

circles-icse-solutions-class-10-mathematics-66

circles-icse-solutions-class-10-mathematics-68

circles-icse-solutions-class-10-mathematics-70

circles-icse-solutions-class-10-mathematics-71

circles-icse-solutions-class-10-mathematics-73

circles-icse-solutions-class-10-mathematics-75

circles-icse-solutions-class-10-mathematics-78

circles-icse-solutions-class-10-mathematics-79

circles-icse-solutions-class-10-mathematics-81

circles-icse-solutions-class-10-mathematics-82

circles-icse-solutions-class-10-mathematics-84

circles-icse-solutions-class-10-mathematics-87

circles-icse-solutions-class-10-mathematics-89

circles-icse-solutions-class-10-mathematics-91

For More Resources

How To Find The Area Of A Sector Of A Circle

December 19, 2020December 19, 2020 by Veerendra

How To Find The Area Of A Sector Of A Circle

How To Find The Area Of A Sector Of A Circle 1
If the arc subtends an angle of θ at the centre, then its arc length is
\( \frac{\text{ }\!\!\theta\!\!\text{ }}{\text{180}}\text{ }\!\!\times\!\!\text{ }\!\!\pi\!\!\text{ r} \)
Hence, the arc length ‘l’ of a sector of angle θ in a
circle of radius r is given by
\( l=\frac{\text{ }\!\!\theta\!\!\text{ }}{\text{180}}\text{ }\!\!\times\!\!\text{ }\!\!\pi\!\!\text{ r }…….\text{ (i)} \)
If the arc subtends an angle θ, then area of the corresponding sector is
\( \frac{\pi {{r}^{2}}\theta }{360} \)
Thus, the area A of a sector of angle θ in a circle of radius r is given by
\( A=~\frac{\theta }{360}\text{ }\times \text{ }\!\!\pi\!\!\text{ }{{r}^{2}} \)
\( =\frac{\theta }{360}\text{ }\times \text{ }\left( \text{Area of the circle} \right)\text{ }……..\text{ (ii)} \)
= × (Area of the circle) ….(ii)
Some useful results to remember:
(i) Angle described by minute hand in 60 minutes = 360º
Angle described by minute hand in one minute
\( ={{\left( \frac{360}{60} \right)}^{0}}=\text{ }6{}^\text{o} \)
Thus, minute hand rotates through an angle of 6º in one minute.
(ii) Angle described by hour hand in 12 hours = 360º
Angle described by hour hand in one hour
\( =\left( \frac{360}{12} \right)_{{}}^{0}=30{}^\text{o} \)

Read More:

Area Of A Sector Of A Circle With Examples

Example 1: A sector is cut from a circle of radius 21 cm. The angle of the sector is 150º. Find the length of its arc and area.
Sol. The arc length l and area A of a sector of angle θ in a circle of radius r are given by
\( l=\frac{\theta }{360}\times 2\pi r\text{ and A=}\frac{\theta }{360}\times \pi {{r}^{2}}\text{ respectively}\text{.} \)
Here, r = 21 cm and q = 150
\(\text{ }l=\left\{ \frac{150}{360}\times 2\times \frac{22}{7}\times 21 \right\}\text{cm}=\text{55 cm} \)
\( \text{and A}=\left\{ \frac{150}{360}\times \frac{22}{7}\times {{(21)}^{2}} \right\}c{{m}^{2}}=\frac{1155}{2}c{{m}^{2}} \)
= 577.5 cm²

Example 2: Find the area of the sector of a circle whose radius is 14 cm and angle of sector is 45º.
Sol. We know that the area A of a sector of angle θ in a circle of radius r is given by
\( A=\frac{\theta }{360}\text{ }\times \text{ }\pi {{r}^{2}} \)
Here, r = 14 cm and θ = 45
\(A=\left\{ \frac{45}{360}\times \frac{22}{7}\times {{(14)}^{2}} \right\}c{{m}^{2}}\)
\( =\left\{ \frac{1}{8}\times \frac{22}{7}\times 14\times 14 \right\}c{{m}^{2}} \)
= 77 cm²

Example 3: In Fig. there are shown sectors of two concentric circles of radii 7 cm and 3.5 cm. Find the area of the shaded region. (Use π = \(\frac { 22 }{ 7 }\)).
Sol. Let A₁ and A₂ be the areas of sectors OAB and OCD respectively. Then, A₁ = Area of a sector of angle 30º in a circle of radius 7 cm
How To Find The Area Of A Sector Of A Circle 2
\( {{A}_{1}}=\left\{ \frac{30}{360}\times \frac{22}{7}\times {{7}^{2}} \right\}c{{m}^{2}}\text{ }\left[ U\sin g:A=\frac{\theta }{360}\times \pi {{r}^{2}}\, \right] \)
⇒ A₁ = \(\frac { 77 }{ 6 }\) cm²
A₂ = Area of a sector of angle 30º in a circle of radius 3.5 cm.
∴ Area of the shaded region
\({{A}_{2}}=\left\{ \frac{30}{360}\times \frac{22}{7}\times {{(3.5)}^{2}} \right\}c{{m}^{2}} \)
\({{A}_{2}}=\left\{ \frac{1}{12}\times \frac{22}{7}\times \frac{7}{2}\times \frac{7}{2} \right\}c{{m}^{2}}=\frac{77}{24}c{{m}^{2}} \)
\( ={{A}_{1}}-{{A}_{2}}=\left( \frac{77}{6}-\frac{77}{24} \right) \)
= \(\frac { 77 }{ 24 }\) × (4 – 1) cm² = \(\frac { 77 }{ 8 }\) cm² = 9.625 cm²

Example 4: A pendulum swings through an angle of 30º and describes an arc 8.8 cm in length. Find the length of the pendulum.
Sol. Here, q = 30º, l = arc = 8.8 cm
\( l=\frac{\theta }{360}\times 2\pi r \)
\( 8.8=~\frac{30}{360}\times 2\times \frac{22}{7}\times r~ \)
\( r=\frac{8.8\times 6\times 7}{22}=16.8\text{ }cm \)

Example 5: The length of minute hand of a clock is 14 cm. Find the area swept by the minute hand in one minute. (Use π = 22/7)
Sol. Clearly, minute hand of a clock describes a circle of radius equal to its length i.e., 14 cm.
Since the minute hand rotates through 6º in one minute. Therefore, area swept by the minute hand in one minute is the area of a sector of angle 6º in a circle of radius 14 cm. Hence, required area A is given by
\( A=\frac{\theta }{360}\times \pi {{r}^{2}}~ \)
\( A=\left\{ \frac{6}{360}\times \frac{22}{7}\times {{(14)}^{2}} \right\} \)
\( A=\left\{ \frac{1}{60}\times \frac{22}{7}\times 14\times 14 \right\}=\frac{154}{15} \)
= 10.26 cm²

Example 6: The perimeter of a sector of a circle of radius 5.2 cm is 16.4 cm. Find the area of the sector.
Sol. Let OAB be the given sector. Then,
Perimeter of sector OAB = 16.4 cm
How To Find The Area Of A Sector Of A Circle 3
⇒ OA + OB + arc AB = 16.4 cm
⇒ 5.2 + 5.2 + arc AB = 16.4
⇒ arc AB = 6 cm
⇒ l = 6 cm
∴ Area of sector OAB = \(\frac { 1 }{ 2 }\) lr
= \(\frac { 1 }{ 2 }\) × 6 × 5.2 cm² = 15.6 cm²

Example 7: The minute hand of a clock is 10cm long. Find the area of the face of the clock described by the minute hand between 9 A.M. and 9.35 A.M.
Sol. We have,
Angle described by the minute hand in one minute = 6º
∴ Angle described by the minute hand in
35 minutes = (6 × 35)º = 210º
∴ Area swept by the minute hand in 35 minutes
= Area of a sector of angle 210º in a circle of radius 10 cm
\(=\left\{ \frac{210}{360}\times \frac{22}{7}\times {{(10)}^{2}} \right\}c{{m}^{2~}}=\text{ }183.3\text{ }c{{m}^{2}}~\text{ }\left[ \text{Using }A=\frac{\theta }{360{}^\text{o}}\times \pi {{r}^{2}} \right] \)

Example 8: The short and long hands of a clock are 4 cm and 6 cm long respectively. Find the sum of distances travelled by their tips in 2 days.
(Take π = 22/7)
Sol. In 2 days, the short hand will complete 4 rounds.
∴ Distance moved by its tip = 4 (Circumference of a circle of radius 4 cm)
\( =4\times \left( 2\times \frac{22}{7}\times 4 \right)=\frac{704}{7}\text{ cm} \)
In 2 days, the long hand will complete 48 rounds.
∴ Distance moved by its tip
= 48 (Circumference of a circle of radius 6 cm)
\( =48\times \left( 2\times \frac{22}{7}\times 4 \right)=\frac{12672}{7}\text{ cm} \)
Hence,
Sum of the distance moved by the tips of two hands of the clock
\( =\left( \frac{704}{7}+\frac{12672}{7} \right)=\text{ }1910.57\text{ }cm \)

Example 9: An elastic belt is placed round the rim of a pulley of radius 5 cm. One point on the belt is pulled directly away from the centre O of the pulley until it is at P, 10 cm from O. Find the length of the belt that is in contact with the rim of the pulley. Also, find the shaded area.
Sol. In the adjacent figure, let ∠AOP = ∠BOP = θ. Clearly, portion AB of the belt is not in contact with the rim of the pulley. In right triangle OAP, we have
How To Find The Area Of A Sector Of A Circle 4
\( \cos \theta =\frac{OA}{OP}=\frac{5}{10}=\frac{1}{2}\text{ }\Rightarrow \text{ }\theta =\text{ }60{}^\text{o} \)
⇒ ∠AOB = 2θ = 120º
\(\text{Arc AB}=\frac{120{}^\text{o}\times 2\times \pi \times 5}{360}=\frac{10\pi }{3}cm \)
\( \left[ \text{Using }l=\frac{\theta }{360}\times 2\pi r \right] \)
Hence, Length of the belt that is in contact with the rim of the pulley
= Circumference of the rim – Length of arc AB
\( =\text{ }2\pi \times 5-\frac{10\pi }{3}=\frac{20\pi }{3}~cm \)
Now,
Area of sector OAQB = \(\frac { 1 }{ 2 }\) × π × 5² cm²
\( =\frac{25\pi }{3}\text{ c}{{\text{m}}^{2}}\text{ }\left[ \text{Using}Area=\frac{\theta }{360}\times \pi {{r}^{2}} \right] \)
Area of quadrilateral OAPB = 2 (Area of ∆OAP)
= 2 × (1/2 × OA × AP)
= 5 × 5√3 cm²
[∵ OP² = OA² + AP² ⇒ AP = \(\sqrt{100-25}\) = 5√3 ]
= 25√3 cm²
Hence,
Shaded area = Area of quadrilateral
OAPB – Area of sector OAQB.
\( =\left( 25\sqrt{3}-\frac{25\pi }{3} \right)=\frac{25}{3}(3\sqrt{3}-\pi )\text{ c}{{\text{m}}^{\text{2}}} \)

Example 10: An arc of a circle is of length 5π cm and the sector it bounds has an area of 20 π cm². Find the radius of the circle.
Sol. Let the radius of the circle be r cm and the arc AB of length 5π cm subtends angle θ at the centre O of the circle. Then,
Arc AB = 5π cm and
Area of sector OAB = 20π cm²
How To Find The Area Of A Sector Of A Circle 5
\( \Rightarrow \frac{\theta }{360}\times 2\pi r=5\pi \text{ and }\frac{\theta }{360}\times \pi {{r}^{2}}=20\pi \)
\( \Rightarrow \frac{\frac{\theta }{360}\times \pi {{r}^{2}}}{\frac{\theta }{360}\times 2\pi r}=\frac{20\pi }{5\pi } \)
⇒ r/2 = 4 ⇒ r = 8 cm
ALTER: We have, Area = 1/2 l r ⇒20π
= 1/2 × 5π × r = 8 cm

Example 11: An umbrella has 8 ribs which are equally spaced. Assuming umbrella to be a flat circle of radius 45 cm. Find the area between the two consecutive ribs of the umbrella.
Sol. Since ribs are equally spaced. Therefore,
Angle made by two consecutive ribs at the centre = \(\frac { 360 }{ 8 }\) = 45º
How To Find The Area Of A Sector Of A Circle 6
Thus,
Area between two consecutive ribs
= Area of a sector of a circle of radius 45 cm and sector angle 45º
\(=\left\{ \frac{45}{360}\times \frac{22}{7}\times 45\times 45 \right\}\text{c}{{\text{m}}^{2}}\text{ }\left[ \text{Using }Area=\frac{\theta }{360}\times \pi {{r}^{2}} \right] \)
= \(\frac { 1 }{ 8 }\) × \(\frac { 22 }{ 7 }\) × 45× 45 cm² = 795.53 cm²

Example 12: A brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire also used in making 5 diameters which divide the circle into 10 equal sectors as shown in Fig. Find:
(i) the total length of the silver wire required
(ii) the area of each sector of the brooch.
Sol. (i) We have,
How To Find The Area Of A Sector Of A Circle 7
Total length of the silver wire = Circumference of the circle of radius
35/2 mm + Length of five diameters
= 2π × \(\frac { 35 }{ 2 }\) + 5 × 35 mm
\( =\left( 2\times \frac{22}{7}\times \frac{35}{2}+175 \right)\text{ mm} \)
= 285 mm
(ii) The circle is divided into 10 equal sectors, Therefore, Area of each sector of the brooch
= 1/10 (Area of the circle)
= 1/10 × π × (35/2)² cm²
\( \text{=}\frac{1}{10}\times \frac{22}{7}\times \frac{35}{2}\times \frac{35}{2}\text{ m}{{\text{m}}^{2}} \)
\( =\frac{385}{4}\text{ m}{{\text{m}}^{2}} \)

Area of Polygons and Circles

December 17, 2020December 17, 2020 by Veerendra

Area of Polygons and Circles

Area formulas can be found at “Reference Table for Areas”
Let’s pick up some hints for those more challenging problems involving area.

Regular polygons have a center and a radius (coinciding with their circumscribed circle), and the distance from the center perpendicular to any side is called its apothem.

Area of Polygons 1

The apothem of a regular polygon is a line segment from the center of the polygon perpendicular to any side of the polygon. Triangle DOC is an isosceles triangle, making the apothem the altitude of this triangle and the median of this triangle (going to the midpoint P.) The apothem is also the radius of the inscribed circle.
The apothem can be used to determine area:

Area of Polygons 2

Area of Polygons 3

Area of Sector of a Circle

Area of Polygons 4