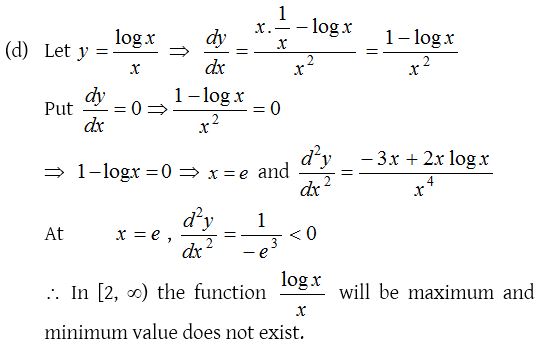How do you find the Minimum and Maximum Values of a Function?
Maxima and Minima
(1) A function f(x) is said to attain a maximum at x = a if there exists a neighbourhood (a – δ, a + δ) such that f(x) < f(a) for all x ∈ (a – δ, a + δ), x ≠ a
⇒ f(x) – f(a) < 0 for all x ∈ (a – δ, a + δ), x ≠ a
In such a case, f (a) is said to be the maximum value of f(x) at x = a.
(2) A function f(x) is said to attain a minimum at x = a if there exists a nbd(a – δ, a + δ) such that f(x) > f(a) for all x ∈ (a – δ, a + δ), x ≠ a
⇒ f(x) – f(a) > 0 for all x ∈ (a – δ, a + δ), x ≠ a
In such a case, f(a) is said to be the minimum value of f(x) at x = a. The points at which a function attains either the maximum values or the minimum values are known as the extreme points or turning points and both maximum and minimum values of f(x) are called extreme or extreme values.
Thus a function attains an extreme value at x = a if f(a) is either a maximum or a minimum value. Consequently at an extreme point a, f(x) – f(a) keeps the same sign for all values of x in a deleted nbd of a.
Necessary condition for extreme values
A necessary condition for f(a) to be an extreme value of a function f(x) is that f’(a) = 0, in case it exists.
Note :
- This result states that if the derivative exists, it must be zero at the extreme points. A function may however attain an extreme value at a point without being derivable there at.
For example, the function f(x) = |x| attains the minimum value at the origin even though it is not differentiable at x = 0. - This condition is only a necessary condition for the point x = a to be an extreme point. It is not sufficient i.e., f’(a) = 0 does not necessarily imply that x = a is an extreme point. There are functions for which the derivatives vanish at a point but do not have an extreme value there at e.g. f(x) = x3 at x = 0 does not attain an extreme value at x = 0 and f’(0) = 0.
- Geometrically, the above condition means that the tangent to the curve y = f(x) at a point where the ordinate is maximum or minimum is parallel to the x-axis.
- The values of x for which f’(x) = 0 are called stationary values or critical values of x and the corresponding values of f(x) are called stationary or turning values of f(x).
- The points where a function attains a maximum (or minimum) are also known as points of local maximum (or local minimum) and the corresponding values of f(x) are called local maximum (or local minimum) values.
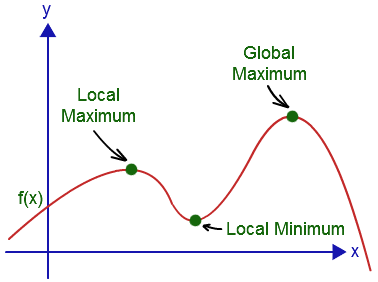
Sufficient criteria for extreme values (1st derivative test)
Let f(x) be a function differentiable at x = a.
Then (a) x = a is a point of local maximum of f(x) if
- f’(a) = 0 and
- f’(a) changes sign from positive to negative as x passes through a i.e., f’(x) > 0 at every point in the left neighbourhood (a – δ, a) of a and f’(x) < 0 at every point in the right neighbourhood (a, a + δ) of a.
(b) x = a is a point of local minimum of f(x) if
- f’(a) = 0 and
- f’(a) changes sign from negative to positive as x passes through a, i.e., f’(x) < 0 at every point in the left neighbourhood (a – δ, a) of a and f’(x) > 0 at every point in the right neighbourhood (a, a + δ) of a.
(c) If f’(a) = 0 but f’(a) does not change sign, that is, has the same sign in the complete neighbourhood of a, then a is neither a point of local maximum nor a point of local minimum.
Working rule for determining extreme values of a function f(x)

If dy/dx changes its sign from positive to negative as x passes through c1, then the function attains a local maximum at x = c1. If dy/dxchanges its sign from negative to positive as x passes through c1, then the function attains a local minimum at x = c1. In case there is no change of sign, then x = c1 is neither a point of local maximum nor a point of local minimum.
Higher order derivative test
- Find f’(x) and equate it to zero. Solve f’(x) = 0 let its roots are x = a1, a2…..
- Find f’’(x) and at x = a1;
- If f’’(a1) is positive, then f(x) is minimum at x = a1.
- If f’’(a1) is negative, then f(x) is maximum at x = a1.
- If f’’(a1) = 0, go to step 3.
- If at x = a1, f’’(a1) = 0, then find f’’’(x). If f’’’(a1) ≠ 0, then f(x) is neither maximum nor minimum at x = a1.
If f’’’(a1) = 0, then find fiv(x).
If fiv(x) is +ve (Minimum value)
fiv(x) is – ve (Maximum value) - If at x = a1, fiv(a1) = 0 then find fv(x) and proceed similarly.
Properties of maxima and minima
- Maxima and minima occur alternately, that is between two maxima there is one minimum and vice-versa.
- If f(x) → ∞ as x → a or b and f’(x) = 0 only for one value of x (say c) between a and b, then f(c) is necessarily the minimum and the least value.
If f(x) → -∞ as x → a or b, then f(c) is necessarily the maximum and the greatest value.
Greatest and least values of a function defined on an interval [a, b]
By maximum (or minimum) or local maximum (or local minimum) value of a function f(x) at a point c ∈ [a, b] we mean the greatest (or the least) value in the immediate neighbourhood of x = c. It does not mean the greatest or absolute maximum (or the least or absolute minimum) of f(x) in the interval [a, b].
A function may have a number of local maxima or local minima in a given interval and even a local minimum may be greater than a relative maximum.
Thus a local maximum value may not be the greatest (absolute maximum) and a local minimum value may not be the least (absolute minimum) value of the function in any given interval.
However, if a function f(x) is continuous on a closed interval [a, b], then it attains the absolute maximum (absolute minimum) at critical points, or at the end points of the interval [a, b]. Thus, to find the absolute maximum (absolute minimum) value of the function, we choose the largest and smallest amongst the numbers f(a), f(c1), f(c2),….f(cn), f(b) where x = c1, c2, ….., cn are the critical points.
Maxima and Minima Problems with Solutions
1.
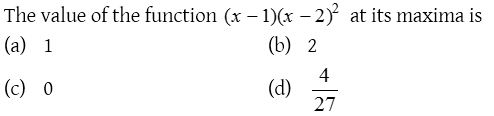
Solution:
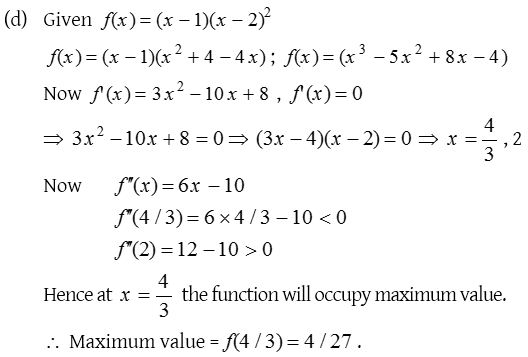
2.
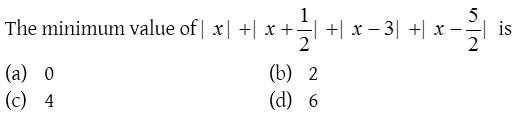
Solution:
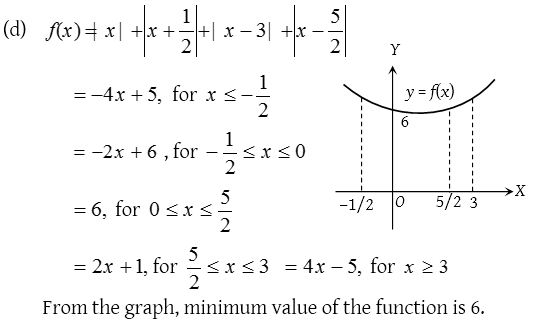
3.

Solution:
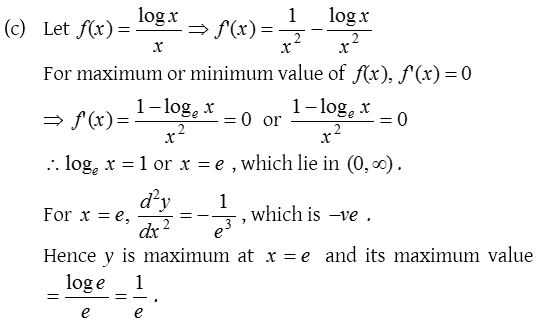
4.

Solution:
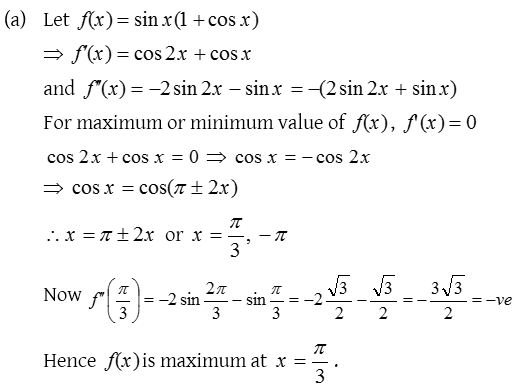
5.

Solution:

6.
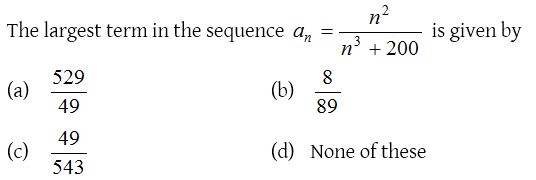
Solution:
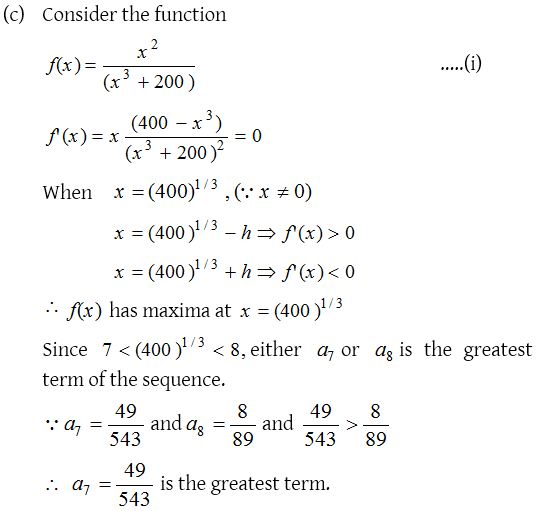
7.
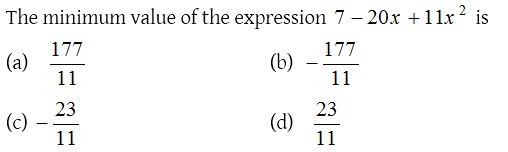
Solution:
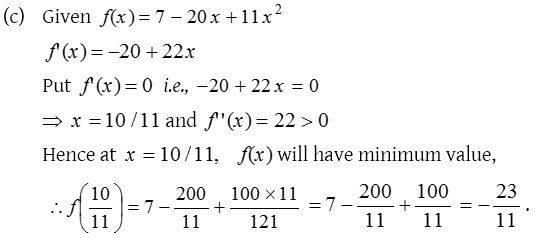
8.

Solution:

9.
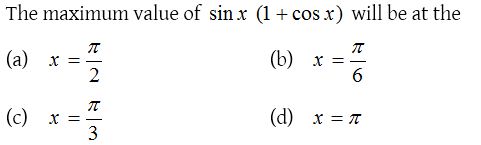
Solution:
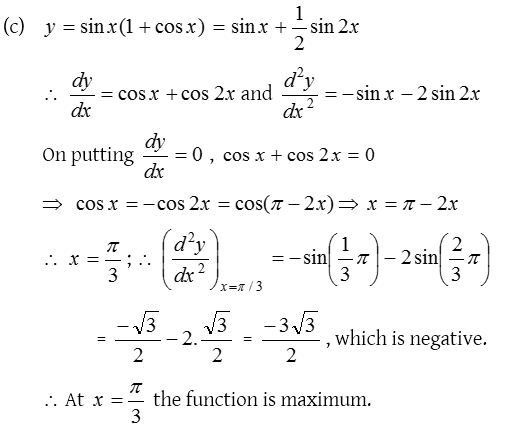
10.
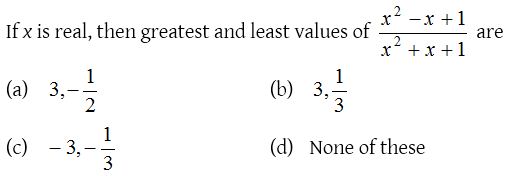
Solution:
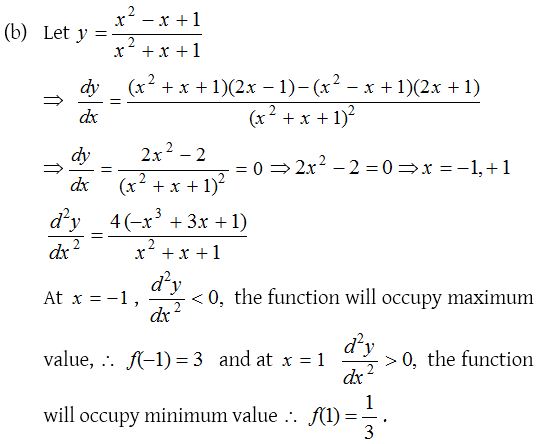
11.
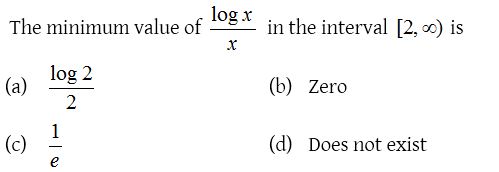
Solution: