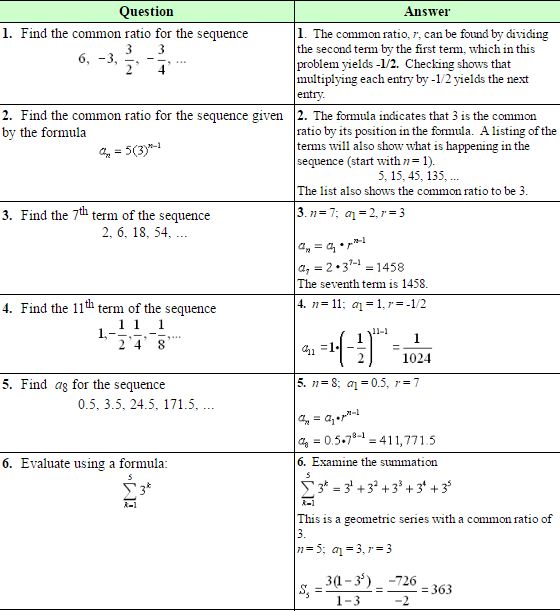
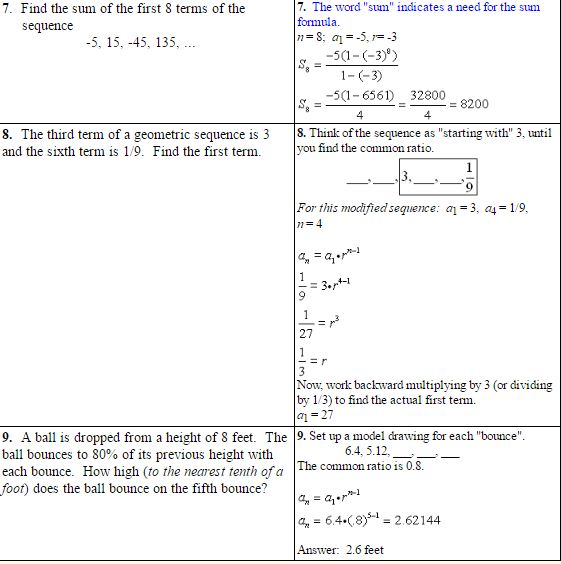
Geometric Sequences and Series
A sequence is an ordered list of numbers.
The sum of the terms of a sequence is called a series.
While some sequences are simply random values, other sequences have a definite pattern that is used to arrive at the sequence’s terms.
Two such sequences are the arithmetic and geometric sequences. Let’s investigate the geometric sequence.
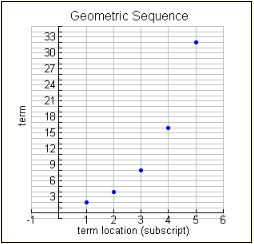
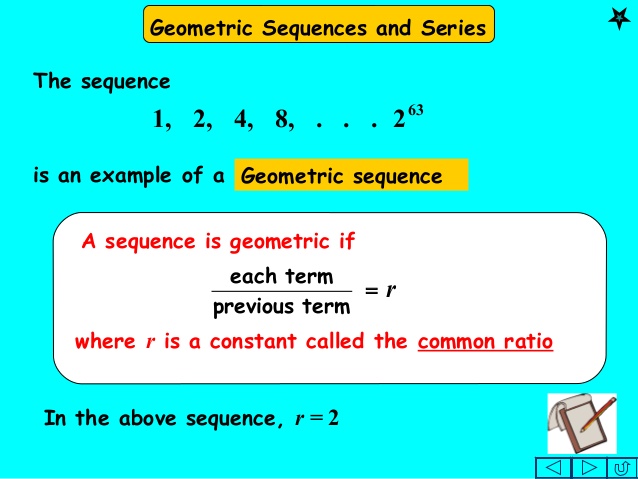
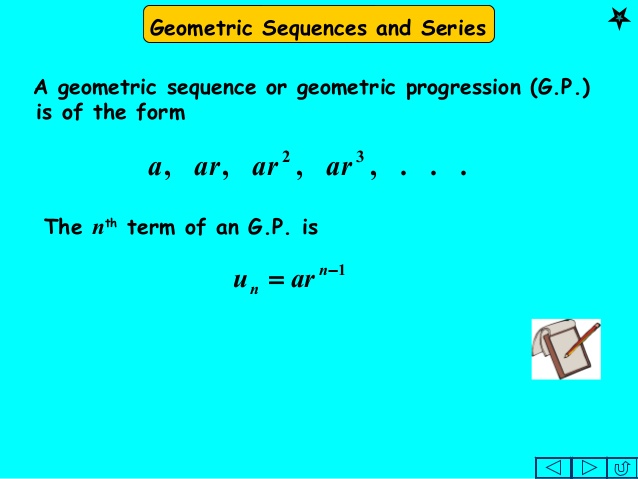
If a sequence of values follows a pattern of multiplying a fixed amount (not zero) times each term to arrive at the following term, it is referred to as a geometric sequence. The number multiplied each time is constant (always the same).
The fixed amount multiplied is called the common ratio, r, referring to the fact that the ratio (fraction) of the second term to the first term yields this common multiple. To find the common ratio, divide the second term by the first term.
Notice the non-linear nature of the scatter plot of the terms of a geometric sequence. The domain consists of the counting numbers 1, 2, 3, 4, … and the range consists of the terms of the sequence. While the x value increases by a constant value of one, the y value increases by multiples of two (for this graph).
Examples:
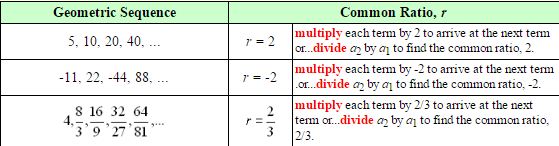
Formulas used with geometric sequences and geometric series:
Examples:
