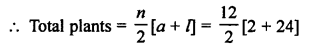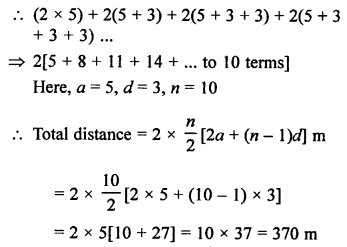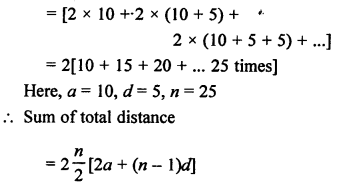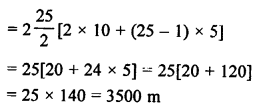Board exams are perhaps the most important aspects of any budding student’s life. When we talk of Board exams, many worries, formalities, tasks, and problems naturally jump in – mostly due to the large scale applicability to every candidate who’s appearing in a particular year. Some of the most important details for any student are the registration number, roll number, unique ID/ registration number, examination centre, and so on. Knowing the course and syllabus is equally essential, but without a unique id and the CBSE registration number, no student can appear for their Boards in a particular year.
If you are someone appearing for class 10 CBSE board exams this year, you might be finding some difficulty in keeping it all together. More so, due to unprecedented situations. But worry no more, this article right here will guide you on everything you need to know about finding your CBSE registration number.
What is the CBSE registration number?
CBSE Registration Number as with other boards is an 8-digit number with the first four digits representing the centre code (usually your school code assigned by CBSE) and the last four numbers follow the roll number depending upon the number of candidates in a school. This number is assigned after the CBSE verification and registration in class 9 that every aspirant has to complete to be eligible for Boards.
This is usually the first step into confirming yourself as a CBSE candidate for a particular session, and naturally, completing it properly should be a priority. This is so because everything else directly or indirectly depends on whether you are correctly registered in the initial stage with the Board. The registration number can be found on the registration card that you get, and this is not available on the admit card for exams.
CBSE registrations for regular and private candidates
Usually, there are two kinds of candidates appearing for CBSE Board exams each year – Regular and Private candidates. The method for carrying out the registration process is different for both these cases.
Regular candidates
A student enrolled in any CBSE affiliated school is known as a Regular Candidate.
The CBSE registration for Class 10 regular candidates is carried by their respective schools. Schools need to enter the number of students for whom registration is to be done. This must be decided before commencing the CBSE online registration. Each and every detail has to be very carefully entered with precision and verification as schools are not allowed to change the information later.
Private candidates
Students who do not attend the regular classes in schools and study on their own without any guidance from their school are registered as private candidates. The only difference is that their certificate will be different from the regular candidates. But the percentage of marks and marking scheme given will be the same as any regular student.
Compartment in Class 10 Board Exams
Students declared as ESSENTIAL REPEAT in their 2020 exams, candidates who have been placed in COMPARTMENT in the main exam, candidates with COMPARTMENT in September 2020, candidates declared FAIL in 2015, 2016, 2017, 2018, 2019, candidates of 2020 who wish to appear for improving their performance in any or all subjects, candidates of 2015 or after, willing to appear in an Additional subject, women candidates (bonafide resident of the National Capital Territory of Delhi and has attained the age for appearing in Class X), disabled candidates (bonafide resident of the National Capital Territory of Delhi and has attained the age for appearing in Class X) are all eligible to register themselves as private candidates.
The detailed steps of registration can be found for both Private and Regular candidates on the CBSE official website.
CBSE registrations 2021 – All you need to know
The essential details to be filled in the registration forms include:
- Name of the candidate
- Date of birth
- Father name
- Mother name
- Gender
- Caste category
- Email ID
- Address
- Photo
- Signature
- Mobile number
For CBSE Registration for the academic year 2020-21, The Central Board of Secondary Education has extended the registration dates for all private candidates. An official notification regarding the extension of registration dates was released by the Board on its official website – cbse.nic.in. According to the new update, those candidates who were not able to register can do so from 5th December 2020 to 9th December 2020.
All those candidates who are already registered can make necessary corrections on their registration form from 10th December 2020 to 14th December 2020. Candidates who wish to apply must visit the official CBSE website.
FAQ’s (Frequently Asked Questions) on finding class 10 CBSE registration number
Q 1. Is it necessary to pay a fee for registration?
Answer. Each candidate needs to pay a minimal fee to register.
Q 2. Is it possible to register past the last date?
Answer. No. Students must be very careful to complete the procedure by the last date.
Q 3. Can forms be corrected afterwards?
Answer. There is no provision for correction. Candidates and schools need to be very mindful.