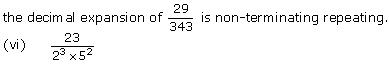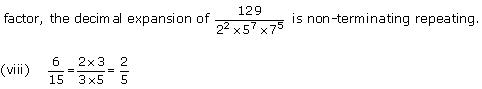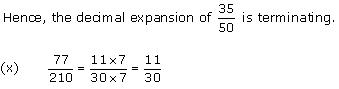Dattu, Author at CBSE Library

ML Aggarwal ICSE Solutions for Class 6 Maths Chapter 12 Symmetry

November 9, 2023May 28, 2022 by Dattu

ML Aggarwal Class 6 Solutions Chapter 12 Symmetry

ML Aggarwal ICSE Solutions for Class 6 Maths Chapter 12 Symmetry

ML Aggarwal Solutions ICSE Solutions Selina ICSE Solutions

ML Aggarwal ICSE Solutions for Class 6 Maths Chapter 12 Symmetry Exercise 12.1

Solution 01:

(i) One
(ii) None
(iii) One
(iv) One
(v) None
(vi) None
(vii) One
(viii) One
(ix) Three

Solution 02:

(i) One
(ii) None
(iii) One
(iv) One
(v) None
(vi) None

Solution 03:
(i) One
(ii) None
(iii) One
(iv) One

Solution 04:

(i) One
(ii) None
(iii) One
(iv) One

Solution 05:

(i) One
(ii) None
(iii) One
(iv) One
(v) None
(vi) None

Solution 06:

J, S, L and K have no line of symmetry.

Solution 07:

ML Aggarwal ICSE Solutions for Class 6 Maths Chapter 12 Symmetry Exercise 12.2

Solution 01:
(i)

(ii)

(iii)

Solution 02:
(i)

(ii)

(iii)

Solution 03:

Solution 04:

Solution 05:

Frank ICSE Solutions for Class 9 Maths Irrational Numbers Ex 1.2

November 10, 2023May 13, 2022 by Dattu

Frank ICSE Solutions for Class 9 Maths Irrational Numbers Ex 1.2

Download Formulae Handbook For ICSE Class 9 and 10

Frank ICSE Solutions for Class 9 Maths Chapter 1 Irrational Numbers Ex 1.2

Answer 1A.

Answer 1B.

Answer 1C.

Answer 1D.

Answer 2A.

Answer 2B.

Answer 2C.

Answer 2D.

Answer 3.

Answer 4.

Answer 5A.

Answer 5B.

Answer 5C.

Answer 5D.

Answer 5E.

Answer 5F.

Answer 6A.

Answer 6B.

Answer 7A.

Answer 7B.

Answer 7C.

Answer 7D.

Answer 8A.

Answer 8B.

Answer 8C.

Answer 9.

Answer 10.

Answer 11.

Answer 12.

Answer 13A.

Answer 13B.

Answer 13C.

Answer 13D.

Answer 13E.

Answer 13F.

Answer 14.

Maths Physics Chemistry Biology

NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.2

November 27, 2020September 4, 2020 by Dattu

In this article, we have provided step by step Chapter 5 Ex 5.2 Class 10 Maths NCERT Solutions.

NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.2 are part of
NCERT Solutions for Class 10 Maths. Here are we have given Chapter 5 Arithmetic Progressions Class 10 NCERT Solutions Ex 5.2.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 5
Chapter Name	Arithmetic Progressions
Exercise	Ex 5.2
Number of Questions Solved	20
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.2

You can also Read Latest NCERT Solutions for Class 10 Maths Chapter-wise to help you to revise the complete Syllabus and score more marks in your examinations.

Page No: 105

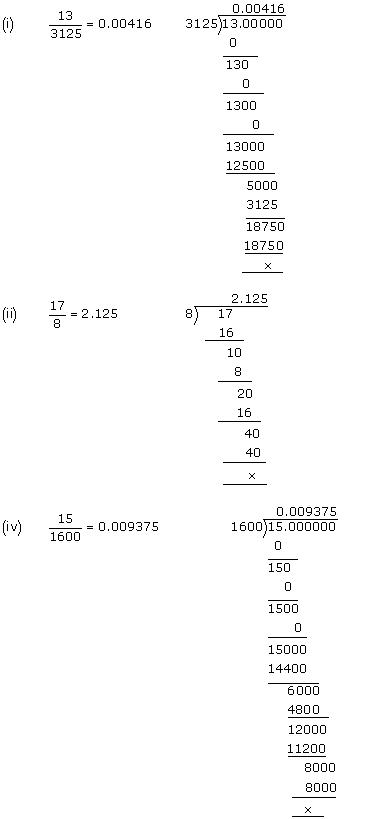
Question 1. Fill in the blanks in the following table, given that a is the first term, d the common difference and a_n the n^th term of the A.P.
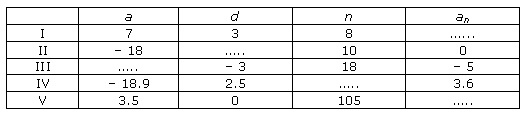
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 67

Solution :
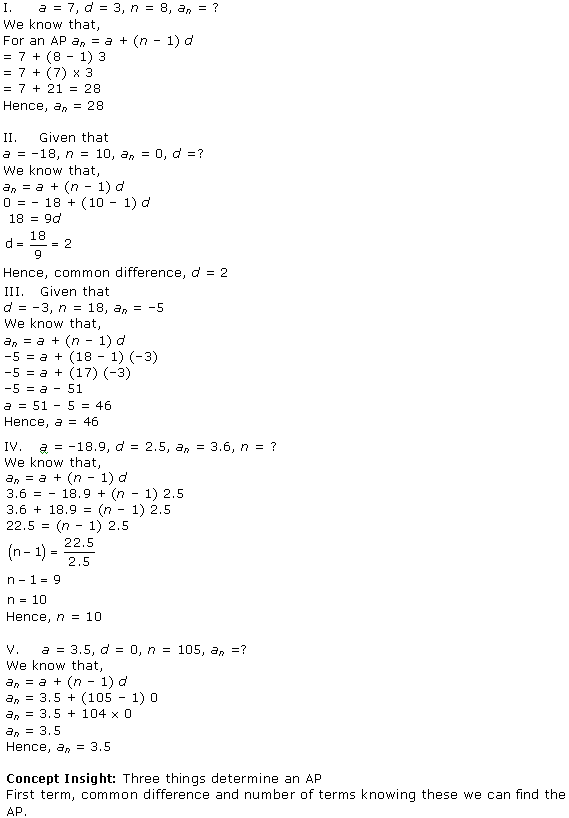
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 6

Question 2. Choose the correct choice in the following and justify
(i) 30^th term of the A.P: 10, 7, 4, …, is
(A) 97 (B) 77 (C) −77 (D.) −87

(ii) 11^thterm of the A.P. -3, -1/2, ,2 …. is
(A) 28 (B) 22 (C) – 38 (D) -48×1/2

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 7

Question 3. In the following APs find the missing term in the boxes.
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 8

Solution :
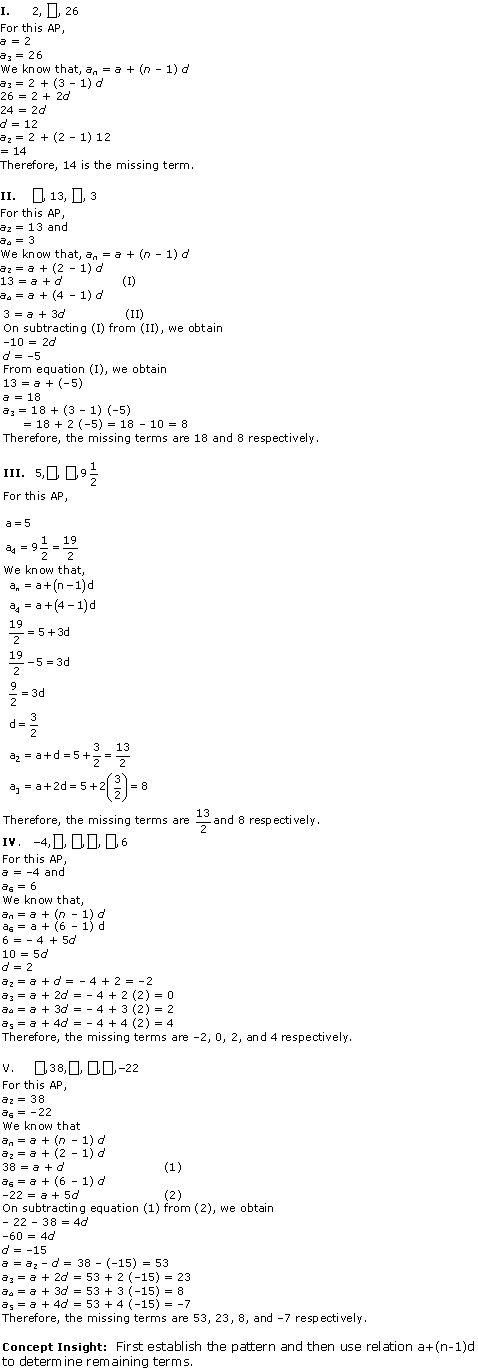
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 9

Question 4. Which term of the A.P. 3, 8, 13, 18, … is 78?

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 10

Question 5. Find the number of terms in each of the following A.P.

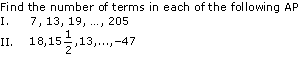
Solution :
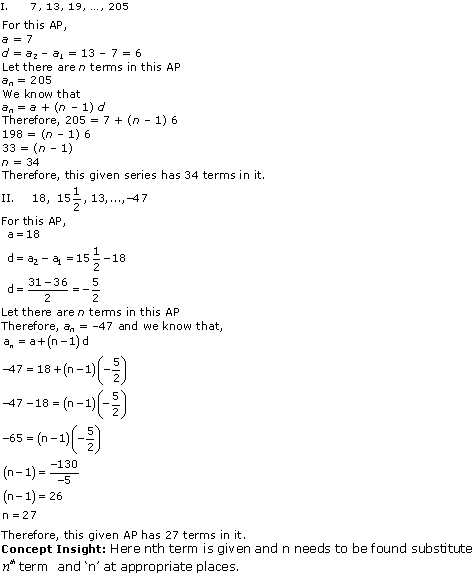
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 12

Question 6. Check whether -150 is a term of the A.P. 11, 8, 5, 2, …

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 13

Question 7. Find the 31^st term of an A.P. whose 11^th term is 38 and the 16^thterm is 73.

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 14

Question 8. An A.P. consists of 50 terms of which 3^rd term is 12 and the last term is 106. Find the 29^th term.

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 15

Question 9. If the 3^rd and the 9^th terms of an A.P. are 4 and − 8 respectively. Which term of this A.P. is zero.

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 16

Question 10. If 17^th term of an A.P. exceeds its 10^th term by 7. Find the common difference.

Solution :
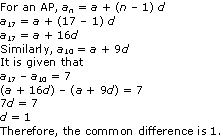
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 17

Question 11. Which term of the A.P. 3, 15, 27, 39, … will be 132 more than its 54^th term?

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 18

Question 12. Two APs have the same common difference. The difference between their 100^th term is 100, what is the difference between their 1000^th terms?

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 19

Question 13. How many three digit numbers are divisible by 7?

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 20

Question 14. How many multiples of 4 lie between 10 and 250?

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 21

Question 15. For what value of n, are the n^th terms of two APs 63, 65, 67, and 3, 10, 17, … equal?

Solution :
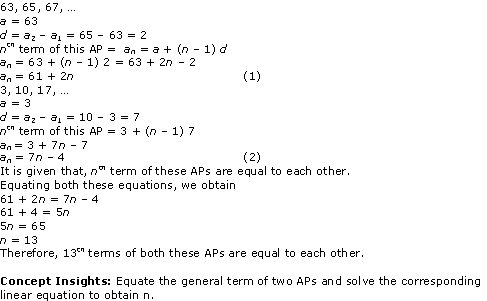
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 22

Question 16. Determine the A.P. whose third term is 16 and the 7^th term exceeds the 5^th term by 12.

Solution :
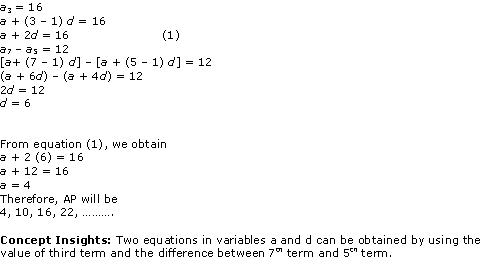
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 23

Page No: 107

Question 17. Find the 20^th term from the last term of the A.P. 3, 8, 13, …, 253.

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 24

Question 18. The sum of 4^th and 8^th terms of an A.P. is 24 and the sum of the 6^th and 10^th terms is 44. Find the first three terms of the A.P.

Solution :
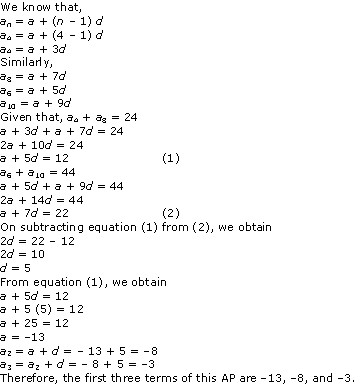
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 25

Question 19. Subba Rao started work in 1995 at an annual salary of Rs 5000 and received an increment of Rs 200 each year. In which year did his income reach Rs 7000?

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 26

Question 20. Ramkali saved Rs 5 in the first week of a year and then increased her weekly saving by Rs 1.75. If in the n^th week, her week, her weekly savings become Rs 20.75, find n.

Solution :
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions 27

We hope the NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.2 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.4

November 27, 2020July 11, 2020 by Dattu

Get the comprehensive Chapter 14 Ex 14.4 Class 10 Maths NCERT Solutions from here and score good marks in CBSE Class 10 Board Examinations.

NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.4 are part of NCERT Solutions for Class 10 Maths. Here are we have given Chapter 14 Statistics Class 10 NCERT Solutions Ex 14.4.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 14
Chapter Name	Statistics
Exercise	Ex 14.4
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.4

NCERT Solutions for Class 10 Maths

Question 1.
The following distribution gives the daily income of 50 workers of a factory.

Daily income (in Rs)	100 – 120	120 – 140	140 – 160	160 – 180	180 – 200
Number of workers	12	14	8	6	10

Convert the distribution above to a less than type cumulative frequency distribution, and draw its ogive.

Solution:

We can find frequency distribution table of less than type as following –

Daily income (in Rs)(upper class limits)	Cumulative frequency
Less than 120	12
Less than 140	12 + 14 = 26
Less than 160	26 + 8 = 34
Less than 180	34 + 6 = 40
Less than 200	40 + 10 = 50

Now taking upper class limits of class intervals on x-axis and their respective frequencies on y-axis we can draw its ogive as following –

NCERT Solutions for Class 10 Maths Chapter 14 Statistics Excercise 14.4 1s

Question 2.
During the medical check-up of 35 students of a class, their weights were recorded as follows:

Weight (in kg)	Number of students
Less than 38	0
Less than 40	3
Less than 42	5
Less than 44	9
Less than 46	14
Less than 48	28
Less than 50	32
Less than 52	35

Draw a less than type ogive for the given data. Hence obtain the median weight from the graph verify the result by using the formula.

Solution:
The given cumulative frequency distributions of less than type is –

Weight (in kg) upper class limits	Number of students (cumulative frequency)
Less than 38	0
Less than 40	3
Less than 42	5
Less than 44	9
Less than 46	14
Less than 48	28
Less than 50	32
Less than 52	35

Now taking upper class limits on x-axis and their respective cumulative frequency on y-axis we may draw its ogive as following

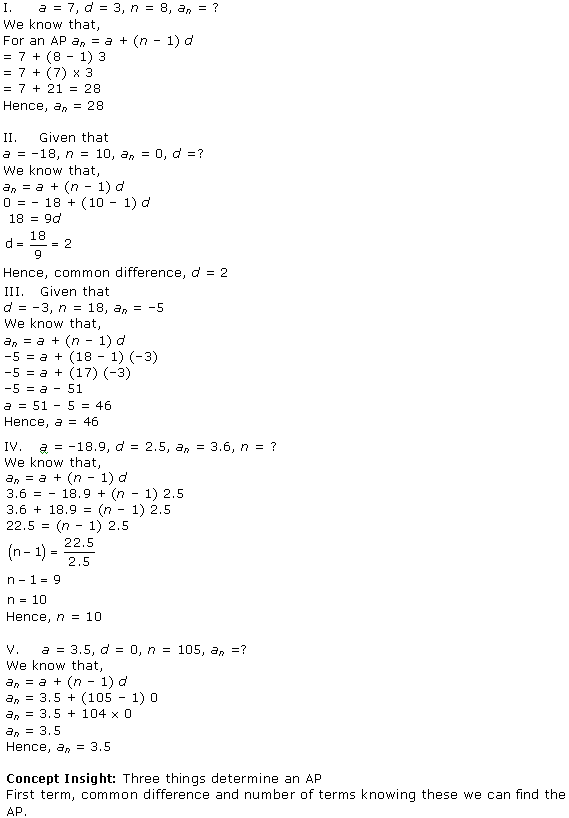
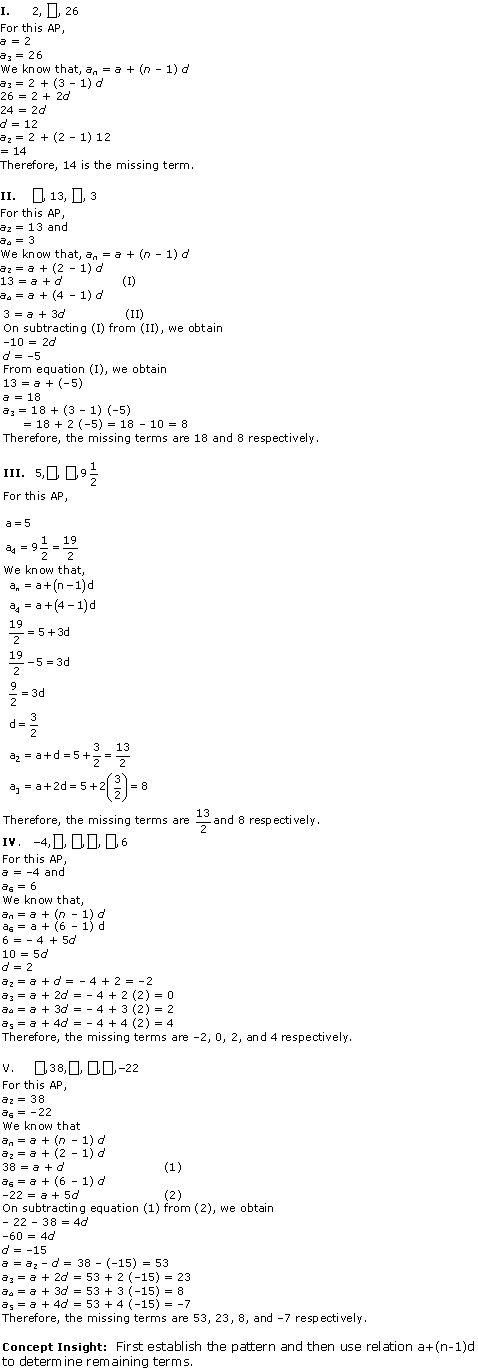
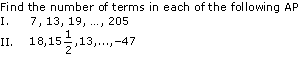
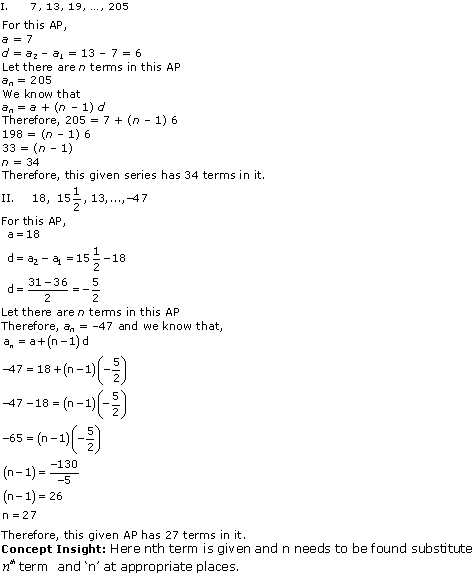
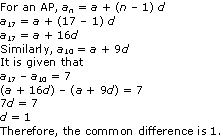
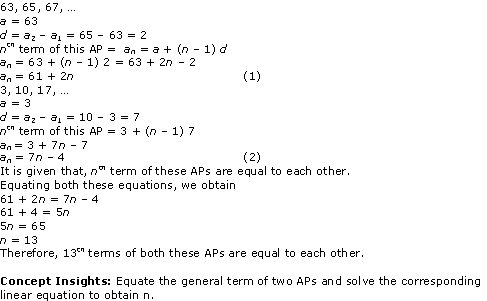
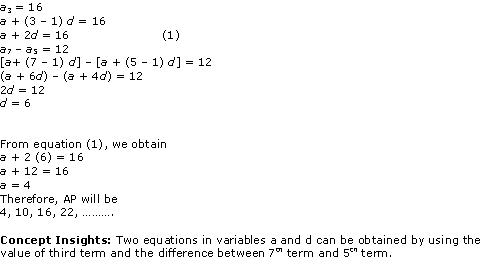
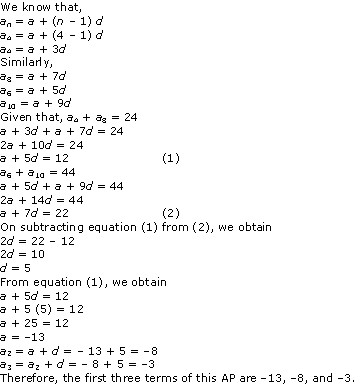
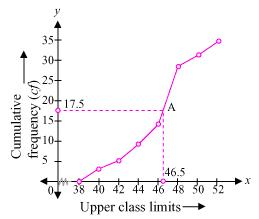
NCERT Solutions for Class 10 Maths Chapter 14 Statistics Excercise 14.4 2s

Now mark the point A whose ordinate is 17.5 its x-coordinate is 46.5. So median of this data is 46.5.
We may observe that difference between two consecutive upper class limits is 2. Now we may obtain class marks with their respective frequencies as below

Weight (in kg)	Frequency (f)	Cumulative frequency
Less than 38	0	0
38 – 40	3 – 0 = 3	3
40 – 42	5 – 3 = 2	5
42 – 44	9 – 5 = 4	9
44 – 46	14 – 9 = 5	14
46 – 48	28 – 14 = 14	28
48 – 50	32 – 28 = 4	32
50 – 52	35 – 32 = 3	35
Total (n)	35

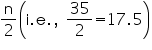Now the cumulative frequency just greater than NCERT Solutions for Class 10 Maths Chapter 14 Statistics Excercise 14.4 2s1 is 28 belonging to class interval 46 – 48
Median class = 46 – 48
Lower class limit (l) of median class = 46
Frequency (f) of median class = 14
Cumulative frequency (cf) of class preceding median class = 14
Class size (h) = 2
NCERT Solutions for Class 10 Maths Chapter 14 Statistics Excercise 14.4 2s2
So median of this data is 46.5
Hence, value of median is verified.

Question 3.
The following table gives production yield per hectare of wheat of 100 farms of a village.

Production yield (in kg/ha)	50 – 55	55 – 60	60 – 65	65 – 70	70 – 75	75 – 80
Number of farms	2	8	12	24	38	16

Change the distribution to a more than type distribution and draw ogive.

Solution:
We can obtain cumulative frequency distribution of more than type as following –

Production yield (lower class limits)	Cumulative frequency
more than or equal to 50	100
more than or equal to 55	100 – 2 = 98
more than or equal to 60	98 – 8 = 90
more than or equal to 65	90 – 12 = 78
more than or equal to 70	78 – 24 = 54
more than or equal to 75	54 – 38 = 16

Now taking lower class limits on x-axis and their respective cumulative frequencies on y-axis we can obtain its ogive as following.
NCERT Solutions for Class 10 Maths Chapter 14 Statistics Excercise 14.4 3s

We hope the NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.4 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.4, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers Ex 1.4

November 27, 2020July 11, 2020 by Dattu

NCERT Maths Solutions for Ex 1.4 Class 10 Real Numbers is the perfect guide to boost up your preparation during CBSE 10th Class Maths Examination.

NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers Ex 1.4 are part of NCERT Solutions for Class 10 Maths. Here are we have given Chapter 1 Real Numbers Class 10 NCERT Solutions Ex 1.4.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 1
Chapter Name	Real Numbers
Exercise	Ex 1.4
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers Ex 1.4

Page No: 17

Question 1
Without actually performing the long division, state whether the following rational numbers will have a terminating decimal expansion or a non-terminating repeating decimal expansion:
(i) 13/3125
(ii) 17/8
(iii) 64/455
(iv) 15/1600
(v) 29/343
(vi) 23/2³× 5²(vii) 129/2²× 5⁷× 7⁵(viii) 6/15
(ix) 35/50
(x) 77/210

Solution:

3125 = 5^₅
The denominator is of the form 5^m.
NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers 17(i)
8 = 2³
The denominator is of the form 2^m.

455 = 5 x 7 x 13
Since the denominator is not in the form 2^_m x 5^_n, and it also contains 7 and 13 as its factors, its decimal expansion will be non-terminating repeating.

1600 = 2^₆ × 5^₂
The denominator is of the form 2^_m x 5^_n.

343 = 7^₃
Since the denominator is not in the form 2^_m x 5^_n, and it has 7 as its factor,
NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers 23
The denominator is of the form 2^_m x 5^_n. Hence, the decimal expansion of is terminating.

(vii)
Since the denominator is not of the form 2^m 5ⁿ, and it also has 7 as its
NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers 26
The denominator is of the form 5^_n.
10 = 2 x 5
The denominator is of the form 2^_m x 5^_n.

30 = 2 x 3 x 5
Since the denominator is not of the form 2^m × 5ⁿ, and it also has 3 as its factors,

Concept Insight: The concept used in this problem is that The decimal expansion of rational number p/q where p and q are coprime numbers, terminates if and only if the prime factorization of q is of the form 2ⁿ5^m, where n and m are non negative integers. Do not forget that 0 is also a non negative integer so n or m can take value 0.
Generally, mistake is committed in identifying terminating decimals when either of the two prime numbers 2 or 5 is appearing in the prime factorization.

Page No: 18

Question 2
Write down the decimal expansions of those rational numbers in Question 1 above which have terminating decimal expansions.

Solution:

NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers 29

Concept Insight:
This is based on performing the long division and expressing the rational number in the decimal form learned in lower classes.

Question 3
The following real numbers have decimal expansions as given below. In each case, decide whether they are rational or not. If they are rational, and of the form p, q you say about the prime factors of q?
(i) 43.123456789
(ii) 0.120120012000120000…
(iii) 43.123456789

Solution:
(i) 43.123456789
Since this number has a terminating decimal expansion, it is a rational number of the form p\q and q is of the form 2^m x 5ⁿ,
i.e., the prime factors of q will be either 2 or 5 or both.

(ii) 0.120120012000120000…
The decimal expansion is neither terminating nor recurring. Therefore, the given number is an irrational number.

(iii) 43.123456789
Since the decimal expansion is non-terminating recurring, the given number is a rational number of the form p/q and q is not of the form 2^_m x 5^_n i.e., the prime factors of q will also have a factor other than 2 or 5.

Concept Insight: The concept used in this problem is that, If the decimal expansion of rational number p\q, [where p and q are coprime numbers] terminates, then prime factorization of q is of the form 2ⁿ5^m, where n and m are non negative integers.

We hope the NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers Ex 1.4 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 1 Real Numbers Ex 1.4, drop a comment below and we will get back to you at the earliest.